Aftershock rate decay induced by postseismic creep: A case study from the MW7.9 Wenchuan earthquake sequence
-
摘要: 基于Dieterich地震活动性理论,本文推导出计算余震发生率和余震累积次数的一般表达式,其中主震后发震断层内部的剪切应力随时间的演化过程遵从Jeffreys-Lomnitz蠕变模型,且与修正Omori定律直接相关。修正Omori定律中的p值与震后断层的短时应力加卸载过程正相关。采用Rubin和Ampuero 给出的震后断层自维持蠕滑模型本文得出计算余震发生率的近似表达式,并对2008年汶川地震序列进行拟合。结果表明,p值的大小直接对应了速率-状态摩擦定律中摩擦参量b/a,而修正Omori定律中的c值则与速率-状态摩擦定律中的临界滑移Dc相关。对于汶川余震序列而言,拟合结果显示b/a约为1.13,Dc约为2—3 cm。Rubin-Ampuero震后自维持蠕滑描述了震后孕震层内部短暂的速率变化特征,是孕震断层演化过程不可缺少的环节。Abstract: Based on the Dieterich seismicity theory, this study derived a general formula used to calculate decay rate and cumulative frequency of aftershocks following a mainshock, of which the shear stress variation in the fault plane follows the Jeffreys-Lomnitz creep law and is directly related to the modified Omori law. The p value in the modified Omori law is positively correlated with post earthquake loading and unloading process of the transient stress. Combined with the postseismic slip model proposed by Rubin and Ampuero, this study obtained approximate formulas to describe the variation of seismicity and to fit aftershock sequence following the 2008 MW7.9 Wenchuan earthquake. The results show that the p value is directly associated with the ratio of b/a, where a and b are the frictional parameters used in rate- and state-dependent friction, and the c value used in the modified Omori law is associated with Dc, a critical creep distance described by rate- and state-dependent friction. For the MW7.9 Wenchuan earthquake sequence, the fitting results indicate the ratio of b/a and Dc are about 1.13 and 2 to 3 cm, respectively. It needs to be emphasized that postseismic creep proposed by Rubin and Ampuero is an inherent stage during earthquake cycle, which describes characteristics of the transient velocity change in seismogenic zone after the mainshock.
-
Keywords:
- aftershock rate /
- postseismic creep /
- stress /
- stressing rate
-
引言
在天然地震学的研究中,最令人关注的现象之一就是中强地震发生后所产生的大量余震,以及强余震本身又触发的各自余震。地震的余震序列为研究地震的成因机制提供了丰富的数据资料。余震时空分布特征的研究和相关地震触发机制的探讨在近二十多年得到了广泛的关注。正如主震后的余震活动,其影响范围在空间上往往超过了主震断层的破裂尺度;时间上,其区域地震活动性显著增加,同时余震的发生率R(t)随时间的增加而逐步衰减。余震的统计特性已得到广泛的关注,其主要集中在对余震时间域和空间域的分布以及余震震级分布特征的探讨。Omori (1894)首次提出了Omori定律即余震发生率R(t,m)与主震后的时间t成反比,即余震发生率随时间不断衰减。经Utsu (1961)修正后得出描述余震发生率的定律—修正Omori定律(modified Omori law,简写为 MOL),即
$R\left({t{\text{,}}m} \right) {\text{=}} \frac{{{\rm d}N\left({t{\text{,}}m} \right)}}{{{\rm d}t}} {\text{=}} \frac{K}{{{{\left[ {c\left(m \right) {\text{+}} t} \right]}^p}}}{\text{,}}$
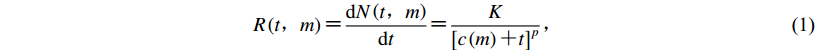
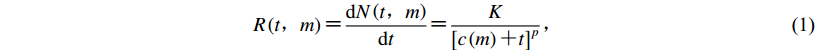
(1) 式中R(t,m)和N(t,m)分别为t时刻震级大于m的余震发生率和累积次数,K和c均为依赖于主震震级的参数,而p的取值通常接近于1,其中间值约为1.1 (Utsu et al,1995 )。
从统计意义上分析,虽然全球大多数中强地震序列满足该经验公式,但仍然无法解答余震成因的物理及力学机制。由于余震数量众多且震级各异,其可以发生在很短的时间尺度和不同的空间尺度内,故其本身也为我们提供了有关地壳内部应力状态的丰富信息,可用于探讨地震的成因机制。构造环境和断裂模式等可作为控制余震序列的主要因素(Kisslinger,Jones,1991;Tahir,Grasso,2012),因此余震序列为研究震源物理提供了极佳的条件。
本文主要分析了余震发生率R(t)随时间的衰减特征,其可能包含震源区的孕震过程和物理条件。例如式(1)中的p值变化可能与断层结构的不均性和地壳内部的应力状态及温度有关,由此可分析出断层孕震区的内摩擦过程及震后的应力松弛和震后蠕滑机制对R(t)的影响(Freed,2005)。
余震的触发机制一般可分为静态应力触发和动态应力触发(Freed,2005)。静态触发与同震位移造成的主断层内部以及周边应力场变化、震后形变和震后应力松弛有关;而动态触发则来自应力波所造成的次生断裂失稳。有关余震触发机制的讨论至今仍是学术研究中的一个重要课题(Freed,2005;Gomberg et al,2005 ;Hainzl,Marsan,2008;Helmstetter,Shaw,2009;Kame et al,2013 ),对该问题的深入研究有助于地震成因分析。本文拟以2008年汶川MW7.9地震序列为例,探讨汶川地震余震序列时变图案特征,并比较不同形式应力变化对应于不同模型的余震触发机制;基于Dieterich (1994)余震触发机制的广义演化模型,阐述应力变化或扰动对余震发生率的影响,以及静态应力扰动Dieterich模型(Dieterich,1994)的局限性;根据Rubin和Ampuero (2005)提出的震后蠕滑模型,采用最小二乘法对汶川余震序列的累积次数进行拟合,同时以MOL的拟合结果作为参考,对比分析不同模型所得拟合的结果,并讨论MOL中参数p和c可能隐含的物理意义。
1. Dieterich地震活动性模型
1.1 余震触发的Dieterich一般解
余震触发的力学机制一般可归结为主震后对区域“闭锁”断层或主震断层“闭锁”部分进行应力的重新加载,其加载源可以来自同震滑移造成的局部或区域应力场应力扰动变化,也可以来自断层震后形变和震后松弛的作用(Perfettini,Avouac,2004,2007;Freed,2005;Hsu et al,2006 ,2007;Savage et al,2007 ;Perfettini,Ampuero,2008;Helmstetter,Shaw,2009;Savage,2010)。因此,可以简单地假定余震发生率与余震区域所受应力或该区域历史应变率呈正相关。基于上述假定,Dieterich (1994)通过引入与应力加载速率相关的地震活动性状态演化变量γ,在假设断层面上所受正应力不变的前提下,将余震发生率R与断层所处的演化过程关联到一起,由此得到
$\left\{ \begin{aligned}& \frac {{\rm{d}} \gamma} {{\rm{d}}t} {\text{+}} \frac{{\dot \tau }}{{a\sigma }}\gamma {\text{=}} \frac{1}{{a\sigma }},\\[4pt]& \frac{R}{{{r_{\rm{o}}}}} {\text{=}} \frac{1}{{{{\dot \tau }_{\rm{r}}}\gamma }},\end{aligned} \right.$
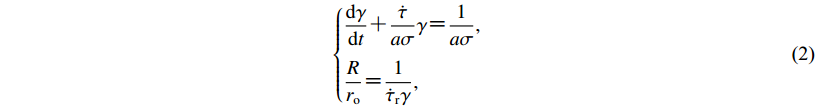
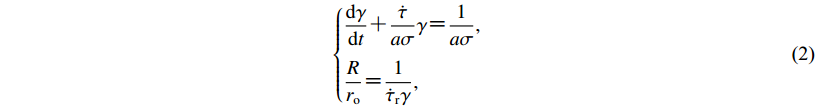
(2) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$R {\text{=}} \frac{{{\rm d}N}}{{{\rm d}t}} {\text{=}} \frac{{{r_{\rm o}}\exp {\displaystyle\frac{{\tau \left( t \right)}}{{a\sigma }}} }}{{1 {\text{+}} t_{\rm a}^{ - 1}\int_0^t {\exp {\displaystyle\frac{{\tau \left( s \right)}}{{a\sigma }}} {\rm d}s} }},$


(3) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$N\left( t \right) {\text{=}} {r_{\rm{o}}}{t_{\rm a}}\ln \left[ {1 {\text{+}} t_{\rm a}^{ - 1}\int_0^t {\exp {\frac{{\tau \left( s \right)}}{{a\sigma }}} {\rm d}s} } \right].$
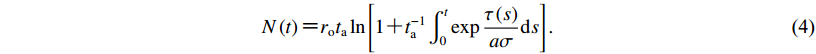
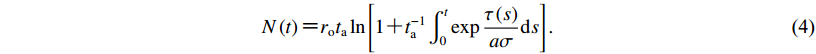
(4) 主震后剪应力τ (t)随时间的演化过程由3个主要部分组成(Perfettini,Avouac,2004),即
$\tau \left(t \right) {\text{=}} {\dot \tau _{\rm r}}t {\text{+}} \Delta {\tau _{\rm c}}\left(t \right) {\text{+}} {\tau _p}\left(t \right), $


(5) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$R\left( t \right){\text{=}} {r_{\rm {o}}}{\left\{ {\left[ {\exp \left( { - \frac{{\Delta {\tau _{{\rm {co}}}}}}{{a\sigma }}} \right) - 1} \right]\exp \left( { - \frac{t}{{{t_{\rm a}}}}} \right) {\text{+}} 1} \right\}^{ - 1}}\text{,}$
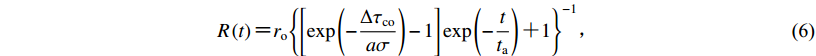
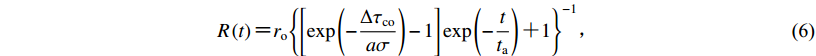
(6) 在t时刻的余震累积次数为
$N\left(t \right) {\text{=}} {r_{\rm o}}t {\text{+}} {r_{\rm o}}{t_{\rm a}}\ln \left[ {\exp{\frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}} {\text{+}} \left( {1 - \exp {\frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}} } \right)\exp \left({ - \frac{t}{{{t_{\rm a}}}}} \right)} \right]{\text{.}} $
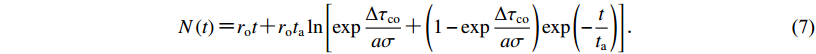
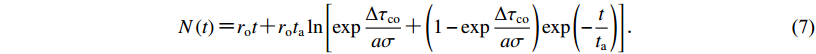
(7) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
1.2 断层的蠕滑
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2) 非对数型蠕滑。Helmstetter和Shaw (2009)提出震后蠕滑产生位移的一般解可写为
$\delta \left(t \right) {\text{=}} \frac{{{V_{\rm{o}}}{t^*}\left[ {{{\left({1 {\text{+}} \displaystyle\frac{t}{t^*}} \right)}^{1 - q}} - 1} \right]}}{{1 - q}} {\text{+}} {V_{\rm{r}}}t{\text{,}} \quad\ {q {\text{>}} 0{\text{且}}q \ne 1}{\text{,}}$
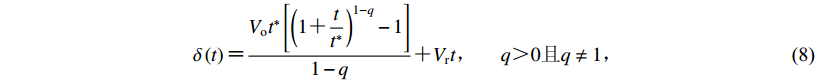
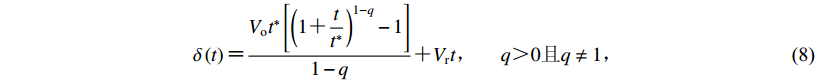
(8) 相应的滑移速率为
$V\left(t \right) {\text{=}} \frac{{{V_{\rm o}}}}{{{{\left({1 {\text{+}} \displaystyle\frac{t}{t^*}} \right)}^{q}}}}, $
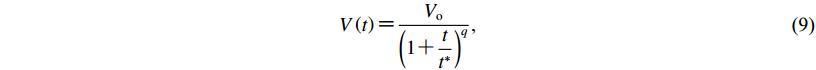
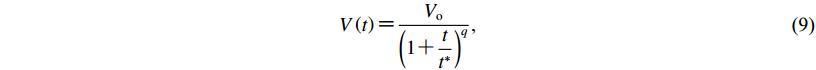
(9) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
3) Rubin-Ampuero自维持蠕滑。基于RSF定律和一维弹簧-滑块断层模型的设定,Rubin和Ampuero (2005)对地震成核和演化过程的模型进行研究得出,当断层的演化时段处于震后和震间阶段时,同震滑移后短时的蠕滑速率可近似为
$V\left( t \right) \approx \frac{{{V_{\rm{o}}}\exp {\displaystyle\frac{t}{{{t_{\rm a}}}}} }}{{{{\left( {1 {\text{+}} \displaystyle\frac{t}{{{\theta _{\rm{o}}}}}} \right)}^{{b/a}}}}},$
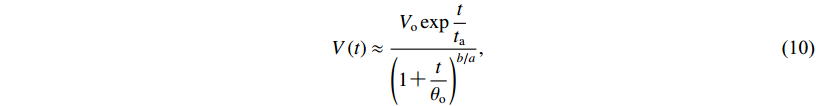
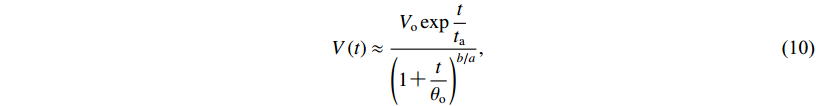
(10) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$V\left( t \right) \approx \frac{{{V_{\rm{o}}}}}{{{{\left( {1 {\text{+}} \displaystyle\frac{t}{{{\theta _{\rm{o}}}}}} \right)}^{{b/a}}}}}.$
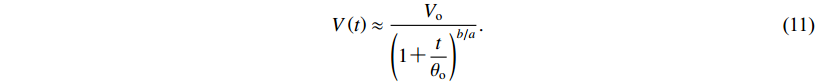
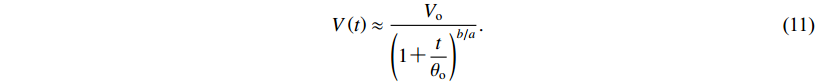
(11) 比较式(11)与式(1),可得到p的取值等于b/a的比值。式(11)与式(9)相同,但q的取值直接与RSF定律中的摩擦参数a和b的比值相关。
对比Rubin-Ampuero模型与上述提到的蠕滑模型(Marone et al,1991 ;Montesi,2004;Perfettini,Avouac,2004),可得:① Rubin-Ampuero模型是断层演化过程(成核—自加速—同震滑移—瞬态蠕滑—震间闭锁)中自身持有的一个特定阶段(Rubin,Ampuero,2005;Segall,2010),而Marone等(1991)的模型则为主震后应力变化所诱发的滑动,这样的滑动可以发生在孕震层的上部、下部或孕震层的内部,但滑动过程中摩擦过程必须是速率强化过程;② 根据Marone等(1991)给出的模型,蠕滑所触发的余震应该发生于孕震层的上部或下部的边界处,而由Rubin-Ampuero模型所导致的触发地震仍可发生在主震的破裂区内;③ 由Marone等(1991)给出的模型所得到的震后形变场变化可持续数年,因此也是一个地表可观测量,但Rubin-Ampuero模型所导致的蠕变可以是瞬态的,且滑移量与Dc的大小相关。Segall (2010)指出,当Dc的取值介于10—100 μm (岩石力学实验结果)时,蠕滑速率从同震滑移速率(约为1 m/s)下降到断层远场滑移速率(Vr)所需时间一般在0.3—3×104 s之间,最大的滑移量约为aDc/(b−a)。因此,在大多数情况下,现今的GPS或InSAR技术均不可能监测到这样的信息;④ 基于摩擦速度强化的对数型震后蠕滑模型本身并不能直接应用到Dieterich模型(式(3)和式(4))。例如在对2004年发生于美国加州Parkfield MW6.0地震的研究结果表明,震后3个月内的滑移量所累积的地震矩(大地测量值)已经相当于主震的地震矩,2.5年后所累积的地震矩则相当于MW6.35地震。而所有余震的积累地震矩却远远小于震后蠕滑所产生的地震矩,相当于MW5.15地震(Barbot et al,2009 )。震后蠕滑和余震地震矩之间的巨大差异只能说明对数型蠕滑只是触发余震的一种可能的机制,震后断层的滑移本身并不是以余震的形式得到松弛的;⑤ 与本文直接相关的问题是如何解释MOL中给出的p值不为1的问题。在Marone等(1991)的模型中所得到的p值约为1,但不能大于1,这与MOL经验定律相违(Utsu et al,1995 ;Ustu,2002),而在Rubin-Ampuero模型中,p值可直接与b/a相关,而b/a的比值大小又与断层的摩擦状态相关, 直接影响到断层的成核与否。基于上述的讨论,本文中我们采用Rubin-Ampuero模型来进一步探讨震后蠕滑与余震发生的相关性。
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${\dot \tau _p}\left( t \right) {\text{=}} \frac{{{{\dot \tau }_{\rm o}}}}{{{{\left( {1 {\text{+}} \displaystyle\frac{t}{{{t^*}}}} \right)}^{ q}}}}, $
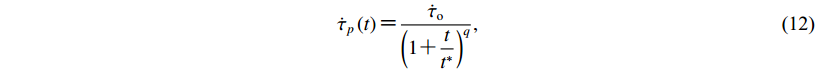
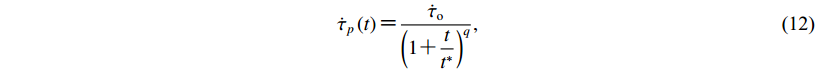
(12) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${\tau _p}\left(t \right) {\text{=}} {\dot \tau _{\rm o}}{t^*}\ln \left({1 {\text{+}} \frac{t}{{{t^*}}}} \right), $


(13) 其中τp(0)是在t=0时刻断层蠕滑产生的剪应力变化。而当q≠1时,则有Jeffreys-Lomnitz型的蠕滑函数
${\tau _p}\left( t \right) {\text{=}} {\dot \tau _{\rm o}}{t^*} {\frac{{{{\left( {{\rm{1}} {\text{+}} \displaystyle\frac{t}{{{t^*}}}} \right)}^{1 - q}} - 1}}{{1 - q}}} .$
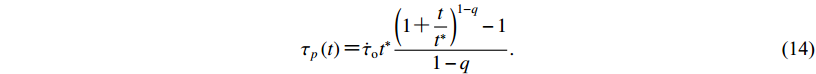
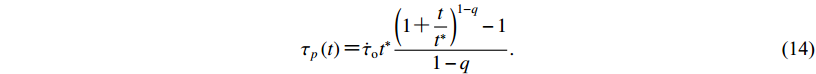
(14) 1.3 基于断层蠕滑模型的地震活动性分析
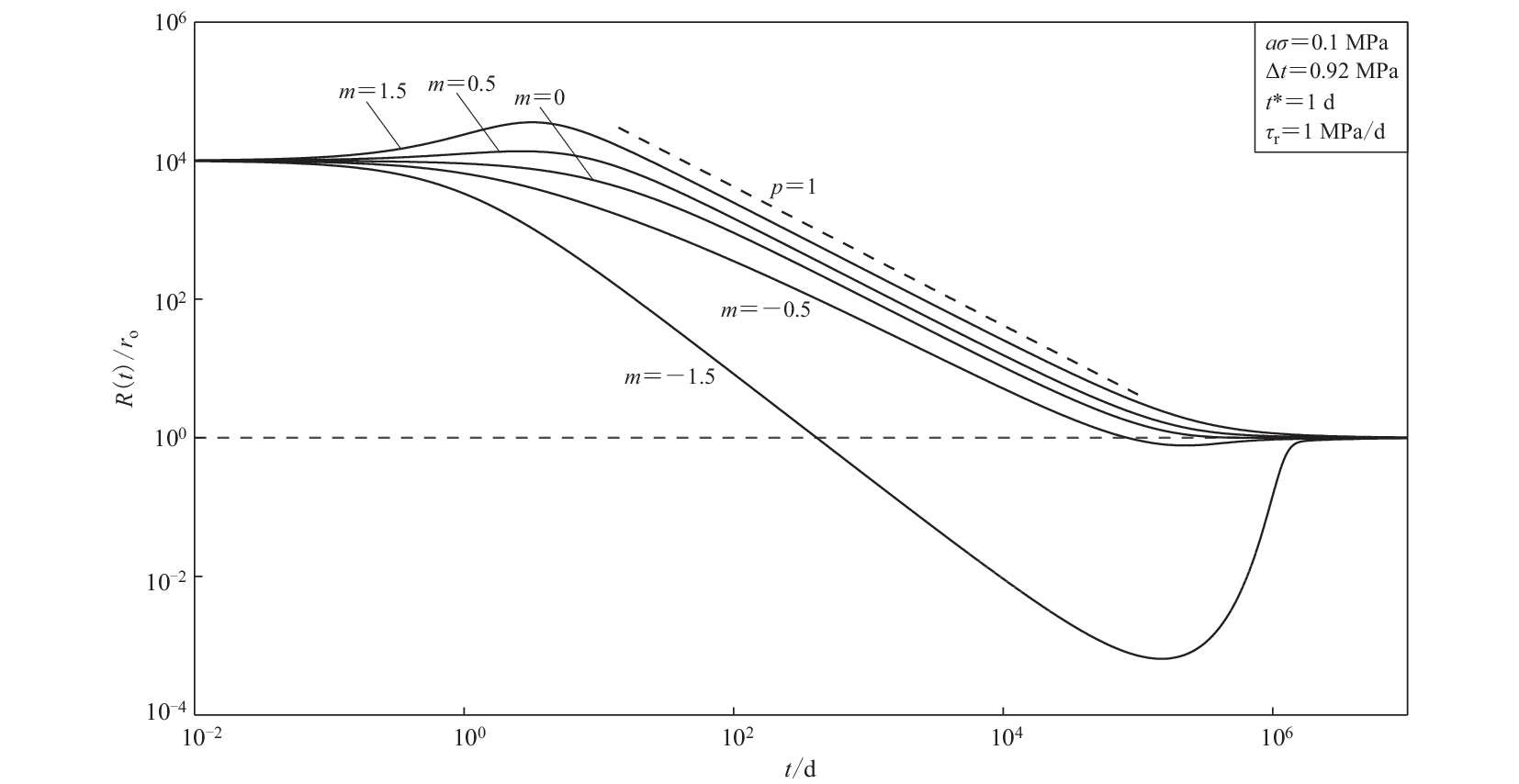
![]() 图 1
图 1This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
Figure 1.This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$I {\text{=}} \int_0^t {\exp \left\{ {\frac{1}{{a\sigma }}\left[ {{{\dot \tau }_{\rm r}}s {\text{+}} \Delta {\tau _{\rm {co}}}H\left(s \right) {\text{+}} {{\dot \tau }_{\rm o}}{t^*}\ln \left({1 {\text{+}} \frac{s}{{{t^*}}}} \right)} \right]} \right\}{\rm d}s} \text{,}$
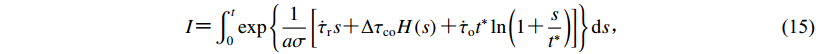
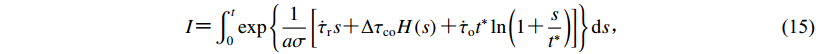
(15) 式(15)可简化为
$I {\text{=}} \exp {\frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}} \int_0^t {\exp {\frac{s}{{{t_{\rm a}}}}} {{\left({1 {\text{+}} \frac{s}{{{t^*}}}} \right)}^m}{\rm d}s}\text{,} $
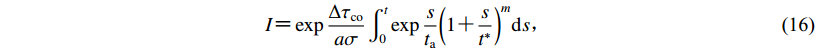
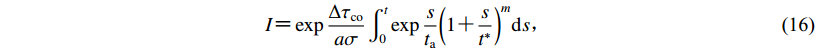
(16) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$I {\text{=}} {t^*}\frac{{{{\left({1 {\text{+}}\displaystyle\frac{t}{t^*}} \right)}^{m {\text{+}} 1}} - 1}}{{m {\text{+}} 1}} \exp {\frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}}\text{.} $
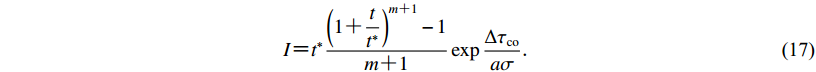
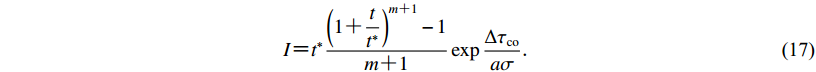
(17) 将式(17)代入式(3)和式(4)可得余震活动的发生率R(t)为(Dieterich,1994;Hainzl, Marsan, 2008;Helmstetter,Shaw, 2009)
$R\left( t \right) {\text{=}} \frac{{{r_{\rm o}}\exp {\displaystyle\frac{{\Delta {\tau _{{\rm {co}}}}}}{{a\sigma }}}{{\left( {1 {\text{+}} \displaystyle\frac{t}{{{t^*}}}} \right)}^m}}}{{\displaystyle\frac{1}{{{t_{\rm a}}}}\exp {\displaystyle\frac{{\Delta {\tau _{{\rm {co}}}}}}{{a\sigma }}} \left[ {\displaystyle\frac{{{t^*}}}{{m {\text{+}} 1}}{{\left( {1 {\text{+}} \displaystyle\frac{t}{{{t^*}}}} \right)}^{m {\text{+}} 1}} - \displaystyle\frac{{{t^*}}}{{m {\text{+}} 1}}} \right] {\text{+}} 1}}\text{,}$
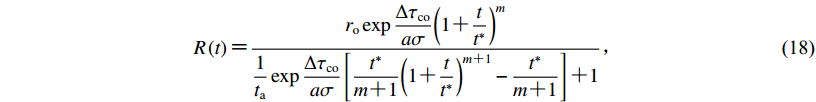
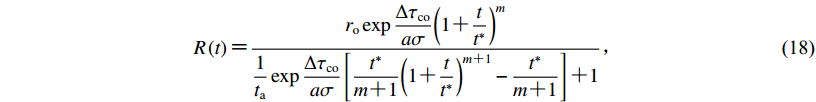
(18) 以及余震的累积次数N(t)为
$N\left(t \right) {\text{=}} {r_{\rm o}}{t_{\rm a}}\ln \left\{ {1 + \frac{{{t^*}}}{{{t_{\rm a}}}}\frac{1}{{m {\text{+}} 1}}\exp {\frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}} \left[ {{{\left({1 {\text{+}} \frac{t}{{{t^*}}}} \right)}^{m {\text{+}} 1}} - 1} \right]} \right\}. $
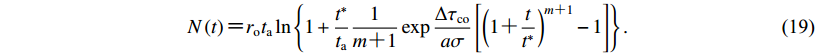
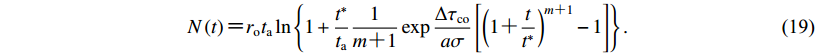
(19) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
1.4 余震触发机制的近似解
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$R\left(t \right) {\text{=}} \frac{{{r_{\rm o}}}}{{{{\dot \tau }_{\rm r}}\left( {{\gamma _{\rm o}} {\text{+}}\displaystyle\frac{t}{{{{a\sigma } }}} } \right)}}\text{,}$
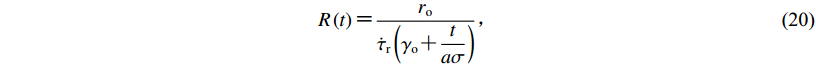
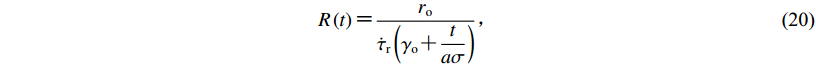
(20) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${t_m} {\text{=}} {t^*}\left\{ {{{\left[ {\exp \left({ - \frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}} \right)\frac{{m\left({m {\text{+}} 1} \right)}}{{{t^*}}}{t_{\rm a}} - m} \right]}^{1/\left({m {\text{+}} 1} \right)}} - 1} \right\}\text{,}$
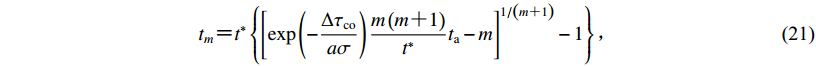
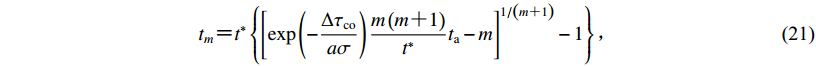
(21) 将式(18)改写为
$R\left(t \right) {\text{=}} \frac{{{r_{\rm{o}}}}}{{\displaystyle\frac{1}{{{t_{\rm a}}}}\displaystyle\frac{{{t^*}}}{{m {\text{+}} 1}}\left({1 {\text{+}} \frac{t}{{{t^*}}}} \right) {\text{+}} {{\left({1 {\text{+}} \displaystyle\frac{t}{{{t^*}}}} \right)}^{ - m}}\left[ {\exp \left({ - \frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}} \right) - \displaystyle\frac{1}{{{t_{\rm a}}}}\displaystyle\frac{{{t^*}}}{{m {\text{+}} 1}}} \right]}}\text{,}$
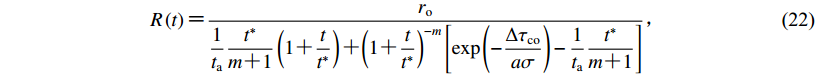
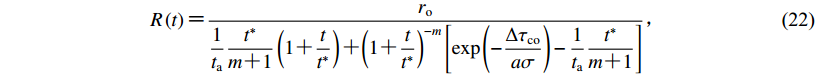
(22) 当ta<t<tm时,式(22)中分母中的第一项远大于第二项(Helmstetter, Shaw,2009),近似为
$R\left(t \right) \approx \frac{{{r_{\rm o}}}}{{\displaystyle\frac{1}{{{t_{\rm a}}}}\displaystyle\frac{{{t^*}}}{{m {\text{+}} 1}}\left({1 {\text{+}} \displaystyle\frac{t}{{{t^*}}}} \right)}} {\text{=}} \displaystyle\frac{{{r_{\rm o}}\left({m {\text{+}} 1} \right){{\dot \tau }_{\rm o}}}}{{\displaystyle\frac{{{t^*}{{\dot \tau }_{\rm o}}{{\dot \tau }_{\rm r}}}}{{a\sigma }}\left({1 {\text{+}} \frac{t}{{{t^*}}}} \right)}} {\text{=}} \displaystyle\frac{{{r_{\rm o}}{{\dot \tau }_{\rm o}}}}{{\left({1 {\text{+}} \displaystyle\frac{t}{{{t^*}}}} \right)}}\displaystyle\frac{{m {\text{+}} 1}}{m{{\dot \tau }_{\rm r}}} {\text{=}} {r_{\rm o}}\left({1 {\text{+}} \frac{1}{m}} \right)\displaystyle\frac{{\dot \tau }}{{{{\dot \tau }_{\rm r}}}}\text{.}$
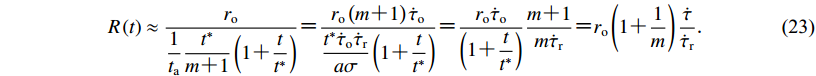
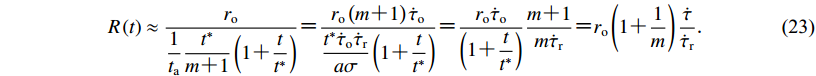
(23) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$R\left(t \right) {\text{≈}} {r_{\rm o}}\exp {\frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}} {\left({1 {\text{+}} \frac{t}{{{t^*}}}} \right)^{ - 1}} {\text{+}} {r_{\rm o}}{\text{.}}$
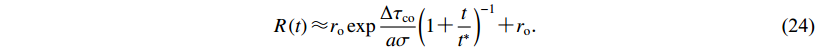
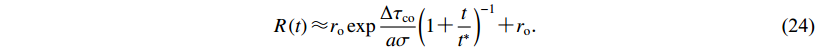
(24) 进而,Helmstetter和Shaw(2009)也证实当q≠1且m>0时,近似式(23)在ta<t<tm时仍然成立。于是在与式(24)同样的假设条件下,R(t)可近似为
$R\left(t \right) {\text{≈}} {r_{\rm o}}\left[ {1 {\text{+}} \exp {\frac{{\Delta {\tau _{\rm {co}}}}}{{a\sigma }}}{{\left({1 {\text{+}} \frac{t}{{{t^*}}}} \right)}^{ - q}}} \right]. $
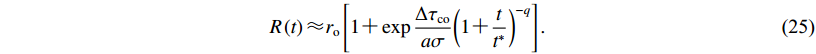
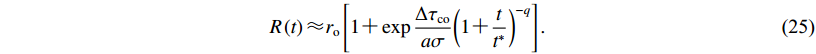
(25) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2. 2008年汶川MW7.9地震余震序列拟合
2.1 区域构造和地震活动性
根据前人的研究(Huang et al,2008 ;陈运泰等,2013;申文豪等,2013),选取汶川MW7.9地震主震及余震集中分布的龙门山断裂带和相邻断裂带为研究区域(30°N—34°N,102°E—107°E)。龙门山断裂带位于青藏高原与扬子地块挤压拼接的交会部位,是新生带以来强烈的褶皱隆起区,包括3条近似平行的主要断裂,即彭县—灌县断裂(前山断裂),映秀—北川断裂(中央断裂)和茂县—汶川断裂(后山断裂)(张勇等,2008;田勤俭等,2009)。历史上龙门山断裂带中强地震主要集在中南段,北段地震活动水平较弱(焦青等,2008)。根据中国地震台网中心得到的地震目录(国家地震科学数据共享中心,2017),并利用最大曲率方法(Mignan,Woessner,2012)可分别计算汶川地震前后地震目录的完备震级Mc,其结果为汶川地震前Mc=4.0,汶川地震后Mc=3.9,这与Jia等(2014)的结果一致,因此本文选择ML≥4.0地震进行分析研究。自1983年至震前ML≥4.0地震共82次,平均发生率为3.28次/年,约为0.009次/天。张致伟等(2009)认为该地区自1970年近38年间地震活动持续平稳,在汶川地震前该区域地震活动的异常现象不明显,可知背景地震发生率R=0.009次/天。根据中国地震台网中心地震目录,截至2013年4月19日,汶川地震共有ML≥3.0余震843次,ML≥4.0余震535次,ML≥5.0余震55次,最大余震为ML6.3。图2给出了主震后ML≥4.0余震序列的空间分布,可看出汶川余震的空间分布基本上与主震断层的走向一致并集中在主震断层周围。
2.2 数据拟合
对式(1)在时间域上积分可以得到MOL经验公式中余震的累积次数为
$N\left(t \right) {\text{=}} \left\{ \begin{aligned}& \displaystyle\frac{K}{{p - 1}}\left[ {{c^{1 - p}} - {{\left({c {\text{+}} t} \right)}^{1 - p}}} \right] {\text{+}} {r_{\rm o}}t\text{,}\;\;\;\;\;\;\;\;p \ne 1\text{,}\\& K{\rm {ln}}\left({c {\text{+}} t} \right) {\text{+}} {r_{\rm o}}t\text{,}\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;p {\text{=}} 1\text{,}\end{aligned} \right.$


(26) 而根据式(11)和式(25),断层蠕滑模型给出的余震累积次数为
$N\left(t \right) {\text{=}} {r_{\rm o}}{\theta _{\rm o}}\exp {\frac{{\Delta {\tau _{\rm co}}}}{{a\sigma }}} \left[{\frac{{{{\left({{\rm{1}} {\text{+}}\displaystyle\frac{t}{{{\theta _{\rm o}}}} } \right)}^{1 - b/a}} - 1}}{{1 - \displaystyle\frac{b}{a}}}} \right]{\text{+}} {r_{\rm o}}t\text{.}$
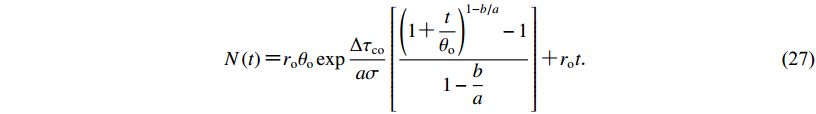
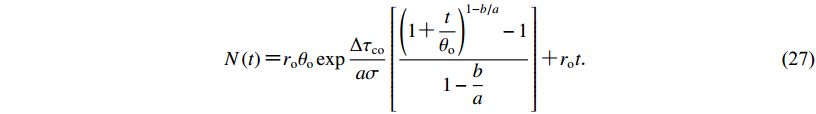
(27) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$r_{\rm d}^2 {\text{=}} 1 - \frac{{\sum\limits_i {{{\left({{N_i} - N_i^\prime } \right)}^2}} }}{{\sum\limits_i {{{\left({{N_i} - \overline N} \right)}^2}} }}\text{,}$
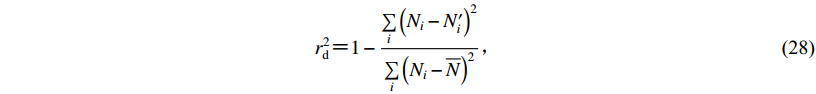
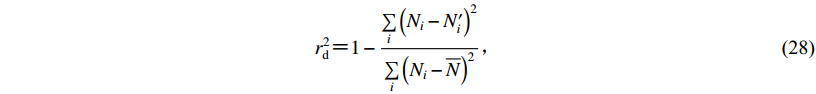
(28) ${\rm RMS}{\text{=}} \sqrt {\frac{{\sum\limits_i {{{\left({{N_i} - N_i^\prime} \right)}^2}} }}{n}} \text{,}$
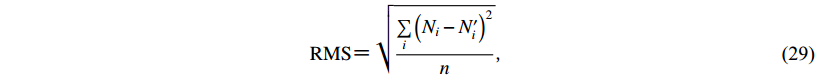
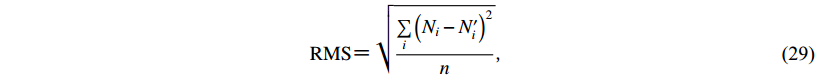
(29) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
表 1 MOL模型参数拟合值Table 1. Fitted parameters in the MOL modelK p c/d RMS $ {r_{\rm d}^2}$ 
76.290 796 433 386 3 1.129 714 921 398 86 0.174 700 213 127 629 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 201 251 5 1.129 714 919 607 34 0.174 700 212 134 727 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 599 558 6 1.129 714 921 574 52 0.174 700 214 565 022 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 420 031 1 1.129 714 920 241 93 0.174 700 213 526 721 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 407 508 2 1.129 714 920 303 60 0.174 700 213 369 922 4.723 176 877 126 94 0.999 064 715 754 963 76.290 795 889 337 1 1.129 714 918 858 40 0.174 700 210 045 648 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 203 779 0 1.129 714 919 793 27 0.174 700 211 916 160 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 358 633 6 1.129 714 920 749 10 0.174 700 212 952 576 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 339 850 3 1.129 714 920 639 84 0.174 700 212 665 134 4.723 176 877 126 93 0.999 064 715 754 963 76.290 796 251 218 9 1.129 714 919 679 88 0.174 700 212 577 751 4.723 176 877 126 94 0.999 064 715 754 963 注:表中K∈[0,500];p∈[0,5];c∈[−10, 10] ( Utsu et al,1995 ;Utsu,2002;Hainzl et al,2016) 。表 2 Dieterich模型参数拟合值Table 2. Fitted parameters in the Dieterich modelta/d exp[Δτco/(aσ)] Δτco/(aσ) RMS $ r_{\rm d}^2$ 
5 882.015 239 530 44 123 970.933 974 009 5.093 319 873 110 68 16.132 130 498 174 8 0.989 089 148 314 182 5 882.014 868 234 99 123 970.963 284 002 5.093 319 975 789 32 16.132 130 498 171 8 0.989 089 148 314 186 5 882.015 309 131 30 123 970.909 997 818 5.093 319 789 117 38 16.132 130 498 174 2 0.989 089 148 314 182 5 882.014 592 899 16 123 970.994 215 420 5.093 320 084 148 10 16.132 130 498 172 7 0.989 089 148 314 184 5 882.014 503 677 40 123 970.987 109 376 5.093 320 059 254 25 16.132 130 498 172 8 0.989 089 148 314 184 5 882.014 976 173 96 123 970.948 098 090 5.093 319 922 590 10 16.132 130 498 169 5 0.989 089 148 314 189 5 882.014 915 449 27 123 970.957 968 035 5.093 319 957 166 45 16.132 130 498 172 2 0.989 089 148 314 185 5 882.015 214 323 03 123 970.935 318 443 5.093 319 877 820 50 16.132 130 498 170 4 0.989 089 148 314 188 5 882.015 150 196 45 123 970.949 854 423 5.093 319 928 742 88 16.132 130 498 170 6 0.989 089 148 314 187 5 882.015 052 767 42 123 970.941 083 807 5.093 319 898 017 70 16.132 130 498 167 5 0.989 089 148 314 192 注:表中ta∈[0,106],exp[Δτco/(aσ)]∈[0,106] (Dieterich,2007; Kame et al,2013 )。表 3 断层蠕滑模型参数拟合值Table 3. Fitted parameters in the postseismic slip modelθo/d b/a exp[Δτco/(aσ)] Δτco/(aσ) RMS $ r_{\rm d}^2$ 
0.174 700 212 670 846 1.129 714 920 608 64 60 844.601 650 449 9 4.784 222 052 123 64 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 368 259 1.129 714 920 027 86 60 844.601 626 682 0 4.784 222 051 953 99 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 213 831 794 1.129 714 921 162 83 60 844.601 370 971 4 4.784 222 050 128 78 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 381 837 1.129 714 920 578 23 60 844.601 713 419 6 4.784 222 052 573 10 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 075 125 1.129 714 919 810 53 60 844.601 679 670 0 4.784 222 052 332 20 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 385 557 1.129 714 920 392 20 60 844.601 712 262 3 4.784 222 052 564 84 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 418 767 1.129 714 919 972 89 60 844.601 611 131 1 4.784 222 051 842 99 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 213 236 443 1.129 714 920 438 69 60 844.601 424 260 9 4.784 222 050 509 15 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 214 115 631 1.129 714 921 173 05 60 844.601 283 946 5 4.784 222 049 507 62 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 211 246 645 1.129 714 919 459 10 60 844.601 866 461 2 4.784 222 053 665 47 4.723 176 877 126 94 0.999 064 715 754 963 注:表中θo∈[0,1],b/a∈[0,5],exp[Δτco/(aσ)]∈[0,106] (Dieterich,2007)。 2.3 结果分析
This page contains the following errors:
error on line 1 at column 68: Extra content at the end of the documentBelow is a rendering of the page up to the first error.
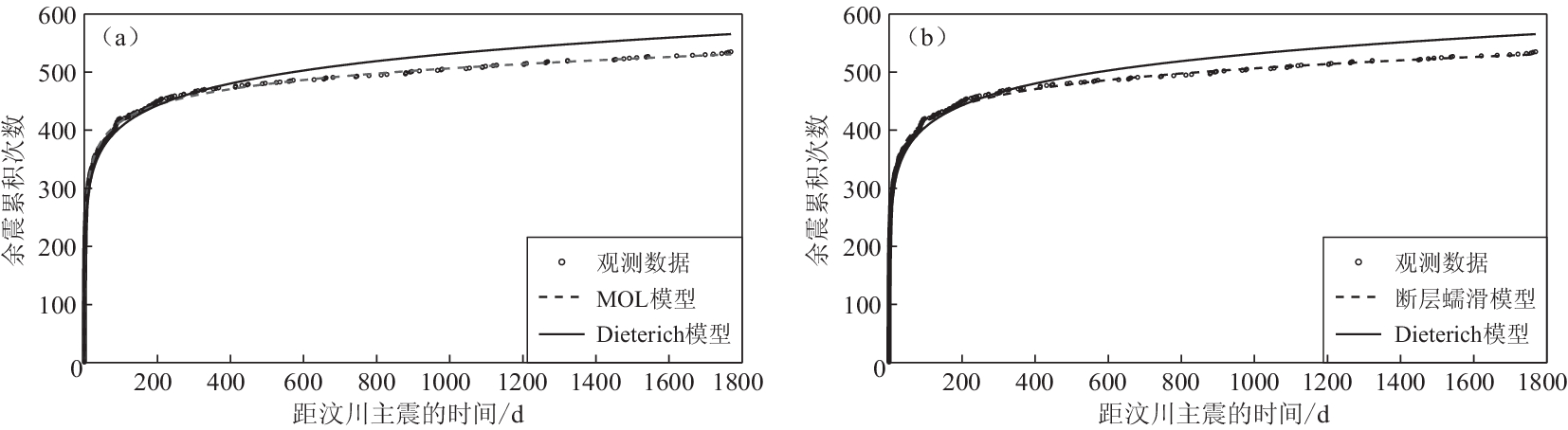
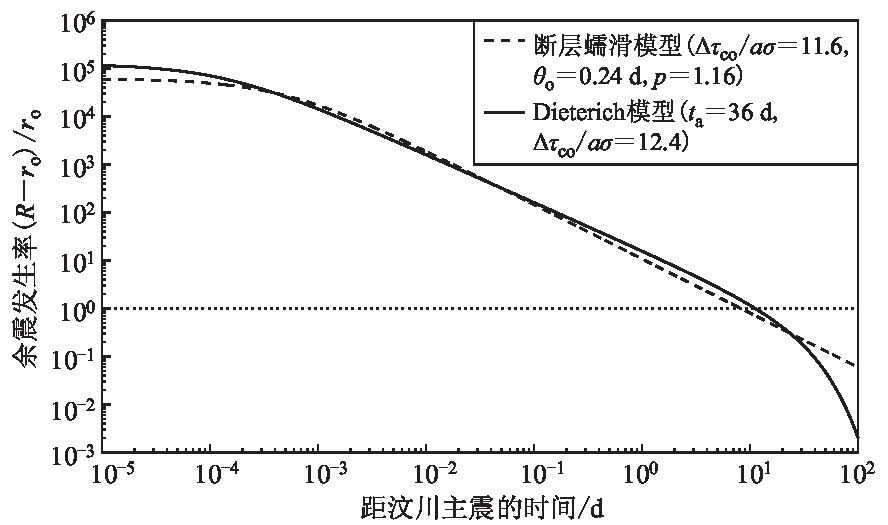
表1图3给出了上述3个模型拟合曲线同实际累积余震次数之间的对比。图3a可见,Dieterich模型所给出的地震累积次数远远大于真实地震次数,尤其当主震后时间大于300 天,拟合曲线所示N(t)远远偏离了真实地震次数。其原因是由Dieterich模型所描述的余震发生率的衰减率p值为1,而实际余震次数的衰减率给出的p值可达1.13。而图3b可以看出,由于断层蠕滑模型和MOL模型具有相同的形式,那么其拟合结果中p应该是相同的,区别在于MOL模型仅为经验模型,拟合过程中的待求参数(K,p和c)并未赋予任何物理意义,而断层蠕滑模型中的待拟合参数则与RSF定律中的摩擦参数a,b和Dc一一对应,这些参数的取值大小决定了断层的摩擦强度和演化过程。
以下我们讨论上述拟合参数所隐含的物理意义。在RSF定律中(Dieterich,1978;Ruina,1983),a和b为摩擦参数,a和b的取值范围一般介于0.001—0.02之间,且b−a约为0.005或更小(Blanpied et al,1998 ;Segall,2010;King,Marone, 2012;He et al,2013 )。模型分析指出(Marone,1998;Scholz,1998),当b>a时,断层可以发生摩擦失稳(宏观地震),对应了摩擦过程的速度弱化,而当b<a时,断层摩擦过程为速度强化过程。上述结论一般均由数值模型和理论分析而得出(Rice,1983;Rice, Ruina,1983;Scholz,1998)。RSF模型中的a和b的取值也只是由岩石物理实验而取得。真实断层摩擦过程中a和b值的取值范围是否与实验结果相一致,也是大家一直所关心的。虽然许多研究人员作了不同的尝试,但对a和b的解读仍然无法达成一致。在目前的研究中,通过余震数据的拟合给出了b/a约为1.13,对应了b>a,且b值只是省略偏离a值。因此,如果b−a约为0.005,a的取值约为0.038,与实验结果具有相同的数量级。Rubin和Ampuero (2005)的断层蠕滑模型也表达了震后蠕滑可以在孕震层中发生,这一点同以往的断层蠕滑模型是有区别的。
在目前所进行的拟合中,能够得到的另一个参数值就是θo,其值具有时间量纲,得出断层蠕滑开始时的状态演化量的取值。而θ本身是RSF定律中的状态演化量,描述了断层摩擦过程中的接触面随时间的演化特征。对于断层蠕滑模型,Rubin和Ampuero (2005)给出θo=Dc/Vo。若Vo给定且θo已知,则可估计Dc的大小。与前述的假设相同,得出Vo=exp[Δτco/(aσ)]Vr,若已知Δτco/(aσ)和Vr,就可估算Dc尺度。
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
![]() 图 3 余震累积次数和不同模型最佳拟合曲线的对比(a) MOL模型与Dieterich模型;(b) 断层蠕滑模型与Dieterich模型Figure 3. Comparison between the temporal distribution of the cumulative number of triggered events corresponding to real data and the best fits obtained by the different models(a) MOL model and Dieterich model;(b) Postseismic slip model and Dieterich model
图 3 余震累积次数和不同模型最佳拟合曲线的对比(a) MOL模型与Dieterich模型;(b) 断层蠕滑模型与Dieterich模型Figure 3. Comparison between the temporal distribution of the cumulative number of triggered events corresponding to real data and the best fits obtained by the different models(a) MOL model and Dieterich model;(b) Postseismic slip model and Dieterich model3. 讨论与结论
基于Dieterich地震活动性模型,我们推导出在任意剪切应力加载演化历史情况下计算余震发生率R(t)和计算余震累积次数N(t)的一般表达式,并指出其适用范围。我们也探讨了当应力函数取αln(1+t/t*)时,余震发生率随时间的衰减,同α的取值有关。α的正负对应了不同的震后过程。因此仅仅采用余震数据拟合无法判别震后应力场的演化情况。当震后蠕滑速率公式中p值不为1时,通过对Helmstetter和Shaw (2009)提出的理论模型中余震发生率公式的近似,可以推导出了计算余震发生率的近似解。采用Rubin和Ampuero (2005)给出的震后蠕滑函数,对2008年汶川地震震级ML≥4.0的余震序列进行了拟合。结果表明RSF定律中的摩擦参数b/a约为1.13,RSF定律中的Dc约为2—3 cm。对于Dieterich模型,它无法对现有的数据进行恰当地拟合,由此预测的余震累积次数远大于实际数据给出的结果。而我们所提出的Rubin-Ampuero蠕滑模型却可以很好的拟合数据并解释MOL,无疑可以成为解释余震成因机制模型中新的一员,不过如何判断不同模型(应力腐蚀,损伤力学和岩石流变学模型,均可对MOL给出部分合理的解释)哪一个更为合理,更符合实际,则需要我们作进一步的研究和讨论。
-
图 1
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
Figure 1.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
图 3 余震累积次数和不同模型最佳拟合曲线的对比
(a) MOL模型与Dieterich模型;(b) 断层蠕滑模型与Dieterich模型
Figure 3. Comparison between the temporal distribution of the cumulative number of triggered events corresponding to real data and the best fits obtained by the different models
(a) MOL model and Dieterich model;(b) Postseismic slip model and Dieterich model
表 1 MOL模型参数拟合值
Table 1 Fitted parameters in the MOL model
K p c/d RMS $ {r_{\rm d}^2}$ 
76.290 796 433 386 3 1.129 714 921 398 86 0.174 700 213 127 629 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 201 251 5 1.129 714 919 607 34 0.174 700 212 134 727 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 599 558 6 1.129 714 921 574 52 0.174 700 214 565 022 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 420 031 1 1.129 714 920 241 93 0.174 700 213 526 721 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 407 508 2 1.129 714 920 303 60 0.174 700 213 369 922 4.723 176 877 126 94 0.999 064 715 754 963 76.290 795 889 337 1 1.129 714 918 858 40 0.174 700 210 045 648 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 203 779 0 1.129 714 919 793 27 0.174 700 211 916 160 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 358 633 6 1.129 714 920 749 10 0.174 700 212 952 576 4.723 176 877 126 94 0.999 064 715 754 963 76.290 796 339 850 3 1.129 714 920 639 84 0.174 700 212 665 134 4.723 176 877 126 93 0.999 064 715 754 963 76.290 796 251 218 9 1.129 714 919 679 88 0.174 700 212 577 751 4.723 176 877 126 94 0.999 064 715 754 963 注:表中K∈[0,500];p∈[0,5];c∈[−10, 10] ( Utsu et al,1995 ;Utsu,2002;Hainzl et al,2016) 。表 2 Dieterich模型参数拟合值
Table 2 Fitted parameters in the Dieterich model
ta/d exp[Δτco/(aσ)] Δτco/(aσ) RMS $ r_{\rm d}^2$ 
5 882.015 239 530 44 123 970.933 974 009 5.093 319 873 110 68 16.132 130 498 174 8 0.989 089 148 314 182 5 882.014 868 234 99 123 970.963 284 002 5.093 319 975 789 32 16.132 130 498 171 8 0.989 089 148 314 186 5 882.015 309 131 30 123 970.909 997 818 5.093 319 789 117 38 16.132 130 498 174 2 0.989 089 148 314 182 5 882.014 592 899 16 123 970.994 215 420 5.093 320 084 148 10 16.132 130 498 172 7 0.989 089 148 314 184 5 882.014 503 677 40 123 970.987 109 376 5.093 320 059 254 25 16.132 130 498 172 8 0.989 089 148 314 184 5 882.014 976 173 96 123 970.948 098 090 5.093 319 922 590 10 16.132 130 498 169 5 0.989 089 148 314 189 5 882.014 915 449 27 123 970.957 968 035 5.093 319 957 166 45 16.132 130 498 172 2 0.989 089 148 314 185 5 882.015 214 323 03 123 970.935 318 443 5.093 319 877 820 50 16.132 130 498 170 4 0.989 089 148 314 188 5 882.015 150 196 45 123 970.949 854 423 5.093 319 928 742 88 16.132 130 498 170 6 0.989 089 148 314 187 5 882.015 052 767 42 123 970.941 083 807 5.093 319 898 017 70 16.132 130 498 167 5 0.989 089 148 314 192 注:表中ta∈[0,106],exp[Δτco/(aσ)]∈[0,106] (Dieterich,2007; Kame et al,2013 )。表 3 断层蠕滑模型参数拟合值
Table 3 Fitted parameters in the postseismic slip model
θo/d b/a exp[Δτco/(aσ)] Δτco/(aσ) RMS $ r_{\rm d}^2$ 
0.174 700 212 670 846 1.129 714 920 608 64 60 844.601 650 449 9 4.784 222 052 123 64 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 368 259 1.129 714 920 027 86 60 844.601 626 682 0 4.784 222 051 953 99 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 213 831 794 1.129 714 921 162 83 60 844.601 370 971 4 4.784 222 050 128 78 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 381 837 1.129 714 920 578 23 60 844.601 713 419 6 4.784 222 052 573 10 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 075 125 1.129 714 919 810 53 60 844.601 679 670 0 4.784 222 052 332 20 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 385 557 1.129 714 920 392 20 60 844.601 712 262 3 4.784 222 052 564 84 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 212 418 767 1.129 714 919 972 89 60 844.601 611 131 1 4.784 222 051 842 99 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 213 236 443 1.129 714 920 438 69 60 844.601 424 260 9 4.784 222 050 509 15 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 214 115 631 1.129 714 921 173 05 60 844.601 283 946 5 4.784 222 049 507 62 4.723 176 877 126 94 0.999 064 715 754 963 0.174 700 211 246 645 1.129 714 919 459 10 60 844.601 866 461 2 4.784 222 053 665 47 4.723 176 877 126 94 0.999 064 715 754 963 注:表中θo∈[0,1],b/a∈[0,5],exp[Δτco/(aσ)]∈[0,106] (Dieterich,2007)。 -
陈学忠, 李艳娥, 郭祥云. 2008. 2008年5月12日四川汶川8.0级地震前后震源区应力水平估计[J]. 国际地震动态, (6): 1-3. Chen X Z, Li Y E, Guo X Y. 2008. Estimation of the stress levels in the focal region before and after the May 12, 2008 Wenchuan, Sichuan earthquake with MS8.0[J]. Recent Developments in World Seismology, (6): 1-3 (in Chinese).
陈运泰, 杨智娴, 张勇, 刘超. 2013. 从汶川地震到芦山地震[J]. 中国科学: 地球科学, 43(6): 1064-1072. Chen Y T, Yang Z X, Zhang Y, Liu C. 2013. From 2008 Wenchuan earthquake to 2013 Lushan earthquake[J]. Science China :Earth Science, 43(6): 1064-1072 (in Chinese).
国家地震科学数据共享中心. 2017. 中国台网统一地震目录[EB/OL]. [2017–09–01]. http://data.earthquake.cn/gcywfl/index.html. Chinese Earthquake Datacenter. 2017. The uniform catalog of China Seismic Network[EB/OL]. [2017–09–01]. http://data.earthquake.cn/gcywfl/index.html (in Chinese).
焦青, 杨选辉, 许丽卿, 王博. 2008. 汶川8.0级地震前后龙门山断裂活动特征浅析[J]. 大地测量与地球动力学, 28(4): 7-11, 37. Jiao Q, Yang X H, Xu L Q, Wang B. 2008. Preliminary study on motion characteristics of Longmenshan fault before and after MS8.0 Wenchuan earthquake[J]. Journal of Geodesy and Geodynamics, 28(4): 7-11, 37 (in Chinese).
申文豪, 刘博研, 史保平. 2013. 汶川MW7.9地震余震序列触发机制研究[J]. 地震学报, 35(4): 461-476. Shen W H, Liu B Y, Shi B P. 2013. Triggering mechanism of aftershocks triggered by Wenchuan MW7.9 earthquake[J]. Acta Seismologica Sinica, 35(4): 461-476 (in Chinese).
田勤俭, 刁桂苓, 郝平, 冯向东, 孙晴. 2009. 汶川8.0级地震及余震破裂的地质构造特征[J]. 地震, 29(1): 141-148. Tian Q J, Diao G L, Hao P, Feng X D, Sun Q. 2009. Earthquake geology of the Wenchuan M8.0 mainshock and its aftershocks[J]. Earthquake, 29(1): 141-148 (in Chinese).
张培震, 徐锡伟, 闻学泽, 冉勇康. 2008. 2008年汶川8.0级地震发震断裂的滑动速率、复发周期和构造成因[J]. 地球物理学报, 51(4): 1066-1073. Zhang P Z, Xu X W, Wen X Z, Ran Y K. 2008. Slip rates and recurrence intervals of the Longmenshan active fault zone, and tectonic implications for the mechanism of the May 12 Wenchuan earthquake, 2008, Sichuan, China[J]. Chinese Journal of Geophysics, 54(4): 1066-1073 (in Chinese).
张勇, 冯万鹏, 许力生, 周成虎, 陈运泰. 2008. 2008年汶川大地震的时空破裂过程[J]. 中国科学: D辑, 38(10): 1186-1194. Zhang Y, Feng W P, Xu L S, Zhou C H, Chen Y T. 2009. Spatial and temporal rupture process of 2008 MW7.8 Wenchuan earthquake, China[J]. Science in China: Series D, 52(2): 145-154.
张致伟, 程万正, 阮祥, 吴朋. 2009. 汶川8.0级地震前龙门山断裂带的地震活动性和构造应力场特征[J]. 地震学报, 31(2): 117-127. Zhang Z W, Cheng W Z, Ruan X, Wu P. 2009. Seismicity and tectonic stress of the Longmenshan fault zone before 2008 Wenchuan MS8.0 earthquake[J]. Acta Seismologica Sinica, 31(2): 117-127 (in Chinese).
Ader T J, Lapusta N, Avouac J P, Ampuero J P. 2014. Response of rate-and-state seismogenic faults to harmonic shear-stress perturbations[J]. Geophys J Int, 198(1): 385-413.
Ariyoshi K, Hori T, Ampuero J P, Kaneda Y, Matsuzawa T, Hino R, Hasegawa A. 2009. Influence of interaction between small asperities on various types of slow earthquakes in a 3-D simulation for a subduction plate boundary[J]. Gondwana Res, 16(3/4): 534-544.
Barbot S, Fialko Y, Bock Y. 2009. Postseismic deformation due to the MW6.0 2004 Parkfield earthquake: Stress-driven creep on a fault with spatially variable rate-and-state friction parameters[J]. J Geophys Res, 114(B7): B07405.
Beeler N M, Lockner D A. 2003. Why earthquakes correlate weakly with the solid Earth tides: Effects of periodic stress on the rate and probability of earthquake occurrence[J]. J Geophys Res, 108(B8): 2391.
Beeler N M. 2004. Review of the physical basis of laboratory-derived relations for brittle failure and their implications for earthquake occurrence and earthquake nucleation[J]. Pure Appl Geophys, 161(9/10): 1853-1876.
Bizzarri A. 2012. What can physical source models tell us about the recurrence time of earthquakes?[J]. Earth-Sci Rev, 115(4): 304-318.
Blanpied M L, Tullis T E, Weeks J D. 1998. Effects of slip, slip rate, and shear heating on the friction of granite[J]. J Geophys Res, 103(B1): 489-511.
Dieterich J H. 1978. Preseismic fault slip and earthquake prediction[J]. J Geophys Res, 83(B8): 3940-3948.
Dieterich J H. 1992. Earthquake nucleation on faults with rate- and state-dependent strength[J]. Tectonophysics, 211(1/2/3/4): 115-134.
Dieterich J. 1994. A constitutive law for rate of earthquake production and its application to earthquake clustering[J]. J Geophys Res, 99(B2): 2601-2618.
Dieterich J H. 2007. Applications of rate- and state-dependent friction to models of fault slip and earthquake occurrence[G]// Treatise on Geophysics, Volume 4: Earthquake Seismology. Amsterdam: Elsevier: 107–129.
Freed A M. 2005. Earthquake triggering by static, dynamic, and postseismic stress transfer[J]. Ann Rev Earth Planet Sci, 33: 335-367.
Freedman D, Pisani R, Purves R. 2007. Statistics[M]. New York: W W Norton & Company Inc: 185–187.
Gomberg J, Reasenberg P, Cocco M, Belardinelli M E. 2005. A frictional population model of seismicity rate change[J]. J Geophys Res, 110(B5): B05S03.
Hainzl S, Marsan D. 2008. Dependence of the Omori-Utsu law parameters on main shock magnitude: Observations and modeling[J]. J Geophys Res, 113(B10): B10309.
Hainzl S, Steacy S, Marsan D. 2010. Seismicity models based on Coulomb stress calculations[EB/OL]. [2010–10–10]. http://www.corssa.org/export/sites/corssa/.galleries/articles-pdf/Hainzl-et-al-2010-CORSSA-Coulomb-models.pdf.
Hainzl S, Christophersen A, Rhoades D, Harte D. 2016. Statistical estimation of the duration of aftershock sequences[J]. Geophys J Int, 205(2): 1180-1189.
He C R, Luo L, Hao Q M, Zhou Y S. 2013. Velocity-weakening behavior of plagioclase and pyroxene gouges and stabilizing effect of small amounts of quartz under hydrothermal conditions[J]. J Geophys Res, 118(7): 3408-3430.
Helmstetter A, Shaw B E. 2009. Afterslip and aftershocks in the rate-and-state friction law[J]. J Geophys Res, 114(B1): B01308.
Hirose T, Shimamoto T. 2005. Slip-weakening distance of faults during frictional melting as inferred from experimental and natural pseudotachylytes[J]. Bull Seismol Soc Am, 95(5): 1666-1673.
Hsu Y J, Segall P, Yu S B, Kuo L C, Williams C A. 2007. Temporal and spatial variations of post-seismic deformation following the 1999 Chi-Chi, Taiwan earthquake[J]. Geophys J Int, 169(2): 367-379.
Hsu Y J, Simons M, Avouac J P, Galetzka J, Sieh K, Chlieh M, Natawidjaja D, Prawirodirdjo L, Bock Y. 2006. Frictional afterslip following the 2005 Nias-Simeulue earthquake, Sumatra[J]. Science, 312(5782): 1921-1926.
Huang Y, Wu J P, Zhang T Z, Zhang D N. 2008. Relocation of the M8.0 Wenchuan earthquake and its aftershock sequence[J]. Science in China: Series D, 51(12): 1703-1711.
Ide S, Takeo M. 1997. Determination of constitutive relations of fault slip based on seismic wave analysis[J]. J Geophys Res, 102(B12): 27379-27391.
Jia K, Zhou S Y, Zhuang J C, Jiang C S. 2014. Possibility of the independence between the 2013 Lushan earthquake and the 2008 Wenchuan earthquake on Longmenshan fault, Sichuan, China[J]. Seismol Res Lett, 85(1): 60-67.
Kame N, Fujita S, Nakatani M, Kusakabe T. 2013. Effects of a revised rate- and state-dependent friction law on aftershock triggering model[J]. Tectonophysics, 600: 187-195.
King D S H, Marone C. 2012. Frictional properties of olivine at high temperature with applications to the strength and dynamics of the oceanic lithosphere[J]. J Geophys Res, 117(B12): B12203.
Kisslinger C, Jones L M. 1991. Properties of aftershock sequences in southern California[J]. J Geophys Res, 96(B7): 11947-11958.
Mainardi F, Spada G. 2012. On the viscoelastic characterization of the Jeffreys-Lomnitz law of creep[J]. Rheol Acta, 51(9): 783-791.
Marone C. 1998. Laboratory-derived friction laws and their application to seismic faulting[J]. Ann Rev Earth Planet Sci, 26: 643-696.
Marone C J, Scholtz C H, Bilham R. 1991. On the mechanics of earthquake after slip[J]. J Geophys Res, 96(B5): 8441-8452.
Mignan A, Woessner J. 2012. Estimating the magnitude of completeness for earthquake catalogs[EB/OL]. [2012-04-01]. http://www.corssa.org/export/sites/corssa/.galleries/articles-pdf/Mignan-Woessner-2012-CORSSA-Magnitude-of-completeness.pdf.
Montesi L G J. 2004. Controls of shear zone rheology and tectonic loading on postseismic creep[J]. J Geophys Res, 109(B10): B10404.
Motulsky H J, Ransnas L A. 1987. Fitting curves to data using nonlinearregression: A practical and nonmathematical review[J]. FASEB J, 1(5): 365-374.
Omori F. 1894. On the after-shocks of earthquakes[J]. J Coll Sci Imp Univ of Tokyo, 7(2): 111-200.
Perfettini H, Avouac J P. 2004. Postseismic relaxation driven by brittle creep: A possible mechanism to reconcile geodetic measurements and the decay rate of aftershocks, application to the Chi-Chi earthquake, Taiwan[J]. J Geophys Res, 109(B2): B02304.
Paterson M S, Wong T F. 2005. Experimental Rock Deformation: The Brittle Field[M]. Berlin: Springer Science & Business Media: 187.
Perfettini H, Avouac J P. 2007. Modeling afterslip and aftershocks following the 1992 Landers earthquake[J]. J Geophys Res, 112(B7): B07409.
Perfettini H, Ampuero J P. 2008. Dynamics of a velocity strengthening fault region: Implications for slow earthquakes and postseismic slip[J]. J Geophys Res, 113(B9): B09411.
Perfettini H, Avouac J P. 2014. The seismic cycle in the area of the 2011 MW9.0 Tohoku-Oki earthquake[J]. J Geophys Res, 119(5): 4469-4515.
Remy D, Perfettini H, Cotte N, Avouac J P, Chlieh M, Bondoux F, Sladen A, Tavera H, Socquet A. 2016. Postseismic relocking of the subduction megathrust following the 2007 Pisco, Peru, earthquake[J]. J Geophys Res, 121(5): 3978-3995.
Rice J R, Ruina A L. 1983. Stability of steady frictional slipping[J]. J Appl Mech, 50(2): 343-349.
Rice J R. 1983. Constitutive relations for fault slip and earthquake instabilities[J]. Pure Appl Geophys, 121(3): 443-475.
Rubin A M, Ampuero J P. 2005. Earthquake nucleation on (aging) rate and state faults[J]. J Geophys Res, 110(B11): B11312.
Ruina A. 1983. Slip instability and state variable friction laws[J]. J Geophys Res, 88(B12): 10359-10370.
Savage J C, Svarc J L, Yu S B. 2007. Postseismic relaxation and aftershocks[J].J Geophys Res, 112(B6): 1–19.
Savage J C. 2010. Calculation of aftershock accumulation from observed postseismic deformation: M6 2004 Parkfield, California, earthquake[J]. Geophys Res Lett, 37(13): L13302.
Scholz C H. 1990. The Mechanics of Earthquakes and Faulting[M]. New York: Cambridge University Press: 263–265.
Scholz C H. 1998. Earthquakes and friction laws[J]. Nature, 391(6662): 37-42.
Segall P. 2010. Earthquake and Volcano Deformation[M]. Princeton: Princeton University Press: 339–348.
Shen W H, Li Y S, Zhang J F.2017. Hybrid stochastic ground motion modeling of the MW7.8 Gorkha, Nepal earthquake of 2015 based on InSAR inversion[J]. Journal of Asian Earth Sciences, 141:
Shen Z K, Sun J B, Zhang P Z, Wan Y G, Wang M, Bürgmann R, Zeng Y H, Gan W J, Liao H, Wang Q L. 2009. Slip maxima at fault junctions and rupturing of barriers during the 2008 Wenchuan earthquake[J]. Nat Geosci, 2(10): 718-724.
Sladen A. 2008. Preliminary result 05/12/2008 (MW7.9), east Sichuan[EB/OL]. [2012–06–21]. http://www.tectonics.caltech.edu/slip_history/2008_e_sichuan/e_sichuan.html.
Tahir M, Grasso J R. 2012. Faulting style controls on the Omori law parameters from global earthquake catalogs[EB/OL]. [2012–01–19]. http://citeseerx.ist.psu.edu/viewdoc/summary?doi=10.1.1.461.1272.
Tsutsumi A, Shimamoto T. 1997. High-velocity frictional properties of gabbro[J]. Geophys Res Lett, 24(6): 699-702.
Utsu T. 1961. A statistical study on the occurrence of aftershocks[J]. Geophys Mag, 30: 521-605.
Utsu T, Ogata Y, Matsu’ura R S. 1995. The centenary of the Omori formula for a decay law of aftershock activity[J]. J Phys Earth, 43(1): 1-33.
Utsu T. 2002. Statistical features of seismicity[J]. Int Geophys, 81: 719-732.
Wang K, Fialko Y. 2014. Space geodetic observations and models of postseismic deformation due to the 2005 M7.6 Kashmir (Pakistan) earthquake[J]. J Geophys Res, 119(7): 7306-7318.
-
期刊类型引用(2)
1. 侯金欣,王宝善,彭志刚,袁松湧. 2008年M_W7.9汶川地震早期余震频度衰减速率空间分布特征分析. 地球物理学报. 2024(08): 3017-3031 .  百度学术
百度学术
2. 刘燕翔,王妍,李金,符泽宇,荣伟健,杨永旺,路彤. 2019年唐山M_L 4.9地震与1976年唐山7.8级地震的相关性分析. 地震地磁观测与研究. 2021(01): 42-47 .  百度学术
百度学术
其他类型引用(0)




 下载:
下载: