Simulation of paleotectonic stress field of Silurian Longmaxi shale reservoirs in Wulong area of southeastern Chongqing
-
摘要:
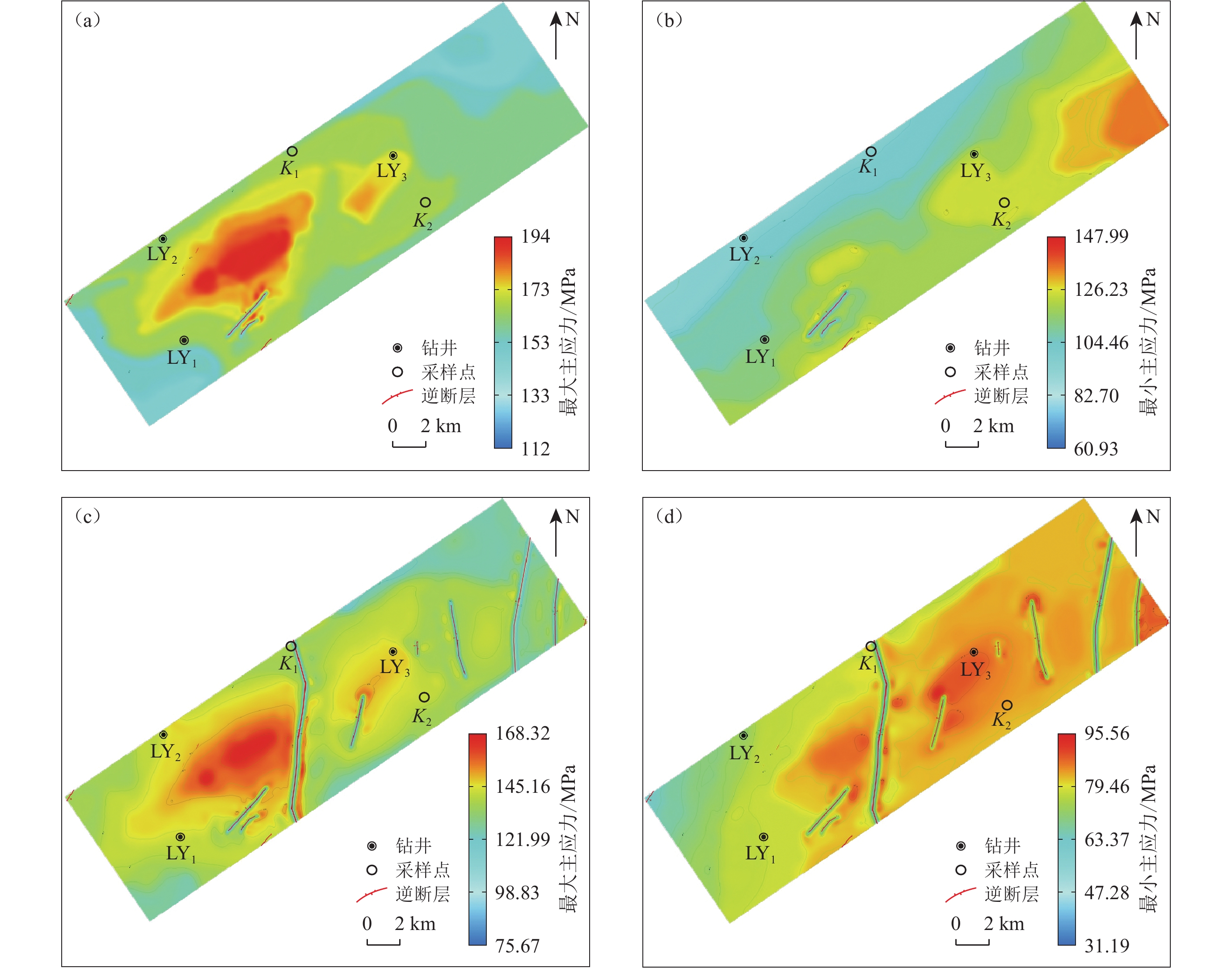
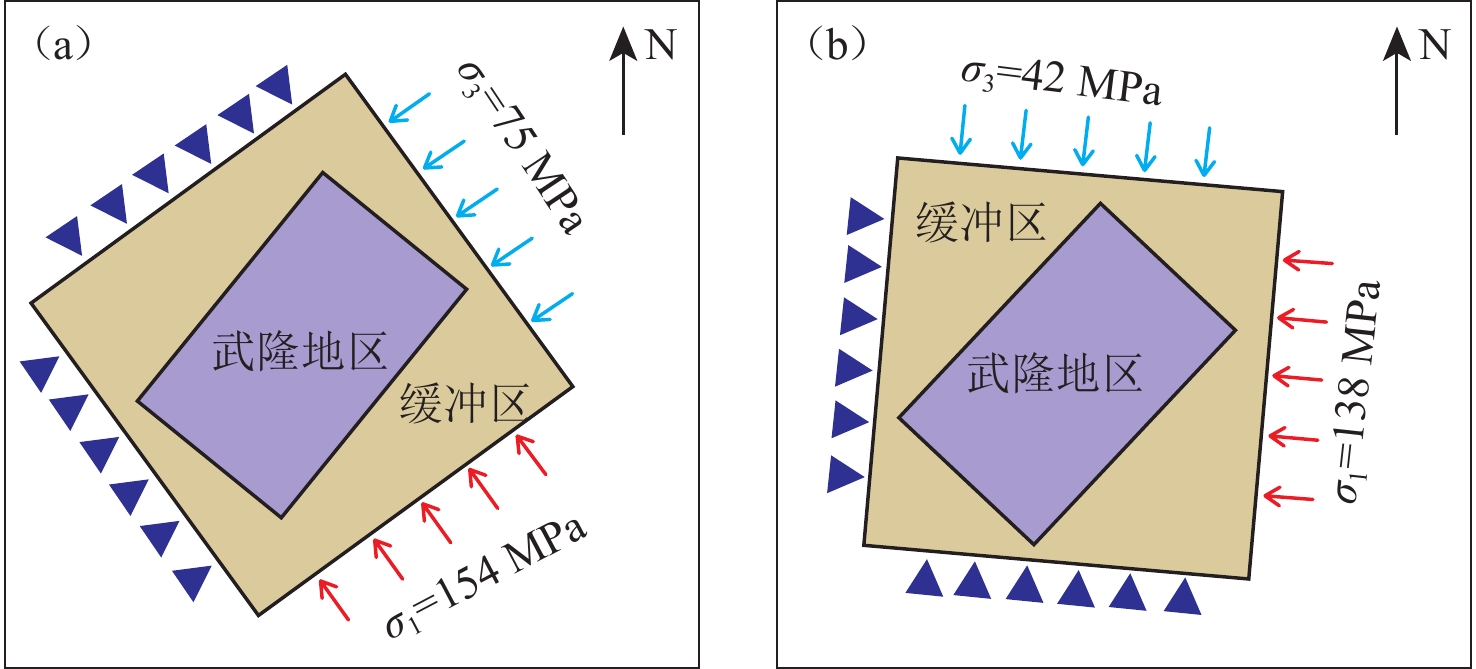
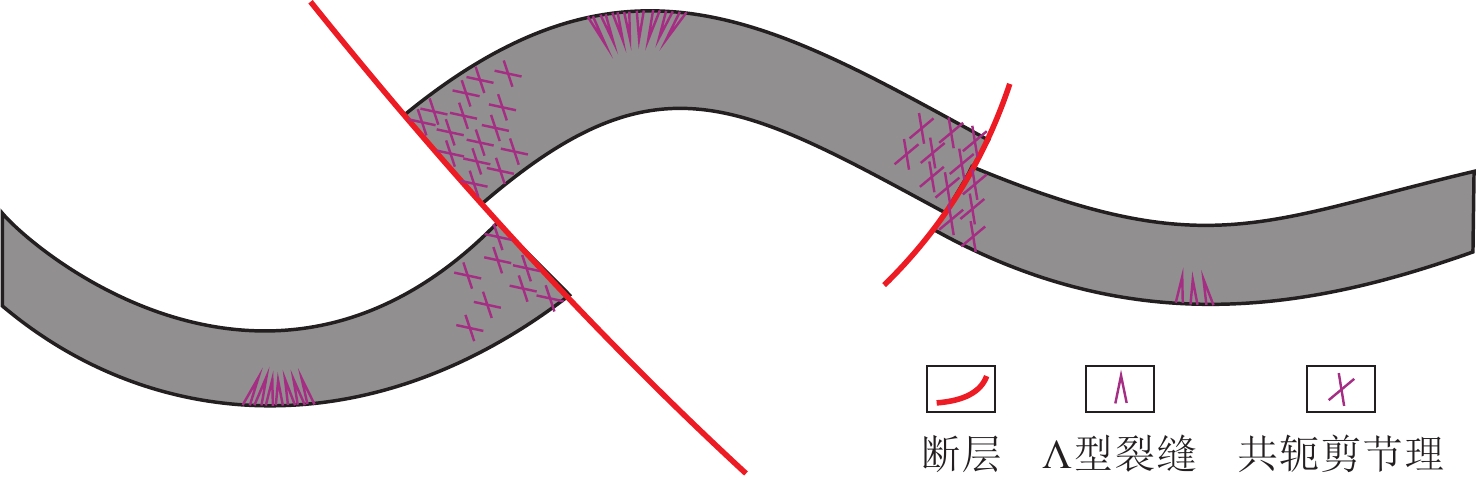
渝东南武隆地区志留系龙马溪组页岩储层发育，构造裂缝是页岩气运移和聚集的主控因素，而古构造应力场控制着构造裂缝的发育，对武隆地区页岩气的勘探开发具有较大影响。本文对武隆地区及周缘发育的节理进行分期配套处理，明确了武隆地区的古构造应力方向，同时通过岩石声发射实验计算得到了古构造应力的大小。在此基础上，利用ANSYS有限元软件建立了武隆地区志留系龙马溪组地层关键构造变形期的地质模型，并通过构造应力场的分析结果明确了该地区关键构造期的构造应力特征，以确定构造应力场数值模拟的边界条件，进而对构造应力场进行数值模拟。研究结果表明：武隆地区受雪峰陆内造山运动的影响，其主要构造变形期为中燕山中期和中燕山晚期，其中燕山中期的最大、最小水平主应力分别为112—194 MPa和60.93—147.99 MPa，中燕山晚期的最大、最小水平主应力分别为75.67—168.32 MPa和31.19—95.56 MPa。模拟结果显示最大水平主应力的高值区大多集中于武隆西向斜核部、断层上盘的局部区域以及断层的拐点和端点处。页岩气有利保存区与断层上盘的距离要远于断层下盘。向斜的核部曲率越大，越易形成“Λ”型裂缝，越有利于页岩气的储集和保存，因此武隆西向斜的页岩气有利保存区优于武隆东向斜。
Abstract:The development of the shale reservoir within the Silurian Longmaxi Formation in the Wulong area of southeastern Chongqing is significantly affected by the superposition of multiple tectonic processes. This has led to intricate structural deformations in the region, making it imperative to analyze the principal controlling elements for shale gas preservation. Given the shale reservoir’s characteristic low porosity and low permeability, tectonic fractures play a pivotal role as the main controlling factor in the migration and accumulation of shale gas. The paleotectonic stress field determines the development of these tectonic fractures, augmenting the reservoir’s storage capacity and enhancing the connectivity among fractures. Consequently, this has a profound impact on the exploration and development of shale gas in the Wulong area. Thus, analyzing the sequence of tectonic evolution, deciphering the direction of the paleotectonic stress field, and quantitatively characterizing the features of the tectonic stress field hold substantial theoretical and practical significance for shale gas exploration and exploitation in this area.
In this research paper, comprehensive field measurements were carried out on the data of conjugate shear joints in the Wulong area and its adjacent periphery. These data were then systematically classified into distinct stages and carefully matched. By leveraging the trend and superposition relationships of fold axes both within the study area and its surrounding regions, the direction of the paleotectonic stress was determined. Through the integration of geochronological data and the outcomes of rock acoustic emission experiments, the tectonic evolution process of the Wulong area was accurately reconstructed. This enabled the determination of the tectonic evolution periods and the corresponding stress magnitudes for each period, while also clarifying the key tectonic deformation period and the fracture distribution traits of the study area. Employing finite element software ANSYS, a three-dimensional geological model was established, specifically focusing on the key tectonic deformation period of the Silurian Longmaxi Formation in the Wulong area. In light of the distribution patterns of rock mechanical parameters in the study area, the mechanical units were appropriately divided, and a corresponding mechanical model was constructed. After multiple rounds of simulation corrections, the optimal boundary stress application conditions for the model were precisely ascertained by analyzing the distinct characteristics of the paleotectonic stress field. Subsequently, numerical simulations of the paleotectonic stress field were executed to elucidate the structural stress characteristics during the key tectonic deformation periods.
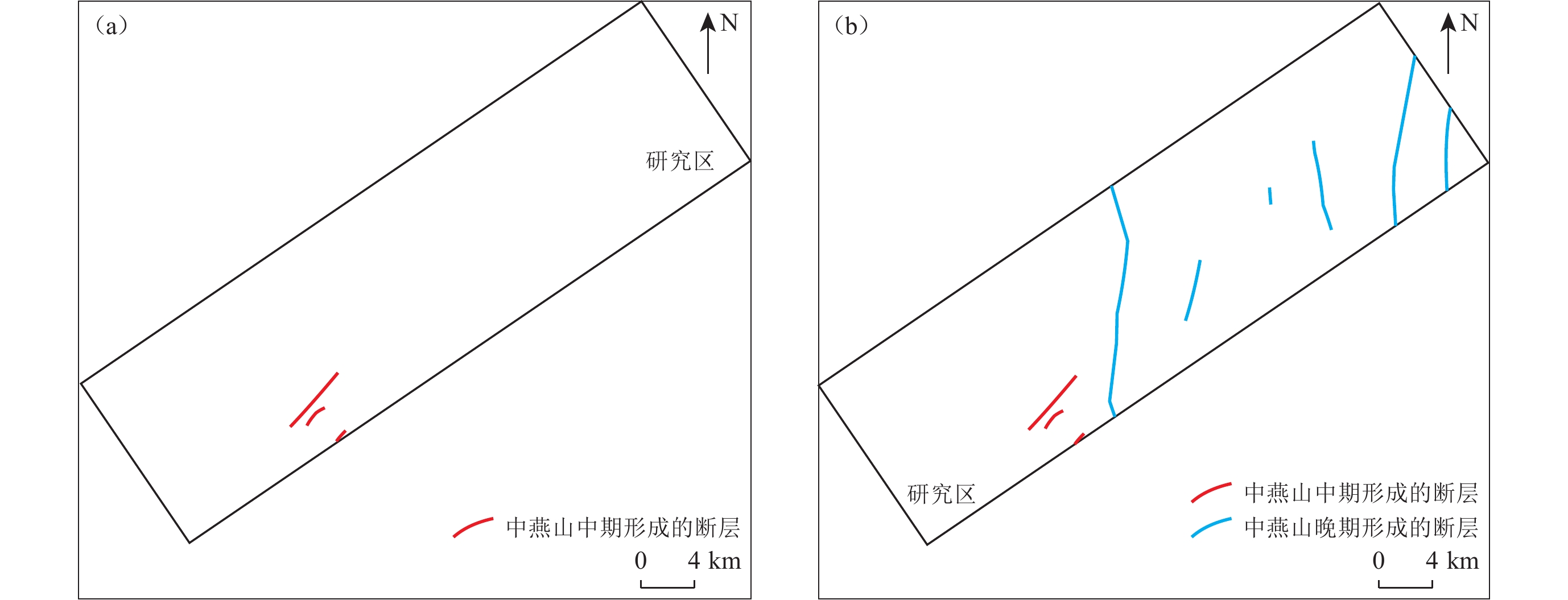
The research findings reveal that since the Middle Yanshanian, the Wulong area has mainly experienced two phases of structural stress. These phases are characterized by stress directions in the SE−NW orientation and close to the EW direction, with the tectonic stress manifesting as compressive. During the Middle Yanshanian, under the influence of the progressive expansion of the Xuefeng intracontinental orogenic movement from the SE−NW direction, a series of NE-trending faults and associated folds emerged in the Wulong area and its neighboring areas. These formations coalesced to form the primary structural pattern of the Wulong area, marking the principal structural deformation period. This pattern has since become the dominant tectonic configuration in the region. However, during the early stage of Middle Yanshanian, the stress environment generated by the Xuefeng intracontinental orogeny underwent a transformation in the study area. The stress changed from the SE−NW compressive state to a nearly EW compressive state. This led to the formation of near SN-trending faults and related folds in the area, which superimposed upon the pre-existing structural morphology. In the process, L-shaped and T-shaped fold structures materialized in the study area and its environs. Although the tectonic activity during this late stage of Middle Yanshanian did not succeed in changing the main NE-trending tectonic pattern in the Wulong area.
Regarding the stress magnitudes, in the middle stage of Middle Yanshanian, the maximum horizontal principal stress ranged from 112 MPa to 194 MPa, while the minimum horizontal principal stress ranged from 60.93 MPa to 147.99 MPa. Notably, the high values of the maximum principal stress were concentrated in the core of the western Wulong syncline. In the late stage of Middle Yanshanian, the maximum horizontal principal stress fluctuated from 75.67 MPa to 168.32 MPa, and the minimum horizontal principal stress varied from 31.19 MPa to 95.56 MPa. Significantly, the stress value of the western Wulong syncline surpassed that of the eastern syncline. It is also worth noting that there exists a discernible correlation between the stress distribution characteristics and the burial depth. The stress contours were distributed in a ring-like fashion, with the maximum stress value at the central region progressively diminishing outward. Additionally, the stress gradient of the low-value zone exhibited a close relationship with fault intensity. Specifically, the greater the fault intensity, the steeper the stress gradient of the maximum principal stress. At the endpoints and inflection points of the faults, the high stress regions emerged, where stress concentration occurred. Moreover the stress on the hanging wall of the fault was higher than that on the footwall. As a result, the degree of fracture development on the hanging wall of the fault was more pronounced than that on the footwall. Consequently, the favorable preservation area for shale gas was located farther from the hanging wall of the fault compared to the footwall. Moreover, stress concentration at the endpoints and inflection points of the faults led to the relatively developed structural fractures that were easily interconnected, facilitating the escape of shale gas. Hence, these areas were unfavorable for shale gas preservation. Furthermore, the deformation degree of the western Wulong syncline was more pronounced than that of the eastern Wulong syncline. The greater the deformation degree of the syncline, the larger the curvature of its core, making it more susceptible to the formation of ‘Λ’-type fractures. These fractures were conducive to the storage and preservation of shale gas. Therefore, the favorable preservation area for shale gas in the western Wulong syncline was superior to that in the eastern Wulong syncline. Studying the characteristics of the paleotectonic stress field lays the foundation for exploring the development degree of tectonic fractures and evaluating the effects of deep fracturing, and it is indeed a critical factor influencing high yields.
-
引言
边坡动力稳定性数值模拟中，有限元强度折减法逐渐成为解决边坡稳定性的热门方法（黄润秋，2007；涂义亮等，2018），此方法的关键在于选取一个合理的土坡失稳判据，由于土坡破坏机制复杂，使得土坡在极限破坏状态时的失稳判据尚未统一。
目前，有三类判据较为常用，分别是塑性区贯通（等效塑性应变）、数值模拟计算不收敛以及特征点的位移突变或位移不收敛（赵尚毅等，2002），通过上述判据来得到土坡的滑动面位置以及边坡强度储备安全系数。现在的多数学者认为，等效塑性应变贯通是边坡破坏的充分非必要条件（赵尚毅等，2005；吕庆等，2008；龙绪健等，2008），且塑性应变幅值的选取标准带有一定的非确定性等人为因素（裴利剑等，2010）。以有限元计算不收敛作为判据的问题是收敛标准受限于选取的软件，收敛误差也可以调整，作为边坡失稳的判据物理意义不明确，受人为因素的影响（郑宏等，2005；王飞阳，潘泓，2016）。特征点的位移突变或位移不收敛，通常特征点的选取都在坡脚、坡面中点和坡肩处（Zienkiewicz et al，1975；宋二祥，1997），但是滑动带的剪出口可能在坡脚上方，从而使得坡脚处的监测点失去意义，且这种情况只能反应边坡局部状态，边坡可能处于局部失稳，并非整体失稳。史卜涛（2016）则是提出了物质点强度折减法，采用坡顶点竖直方向的位移是否突变作为边坡失稳判据，可见从位移角度分析边坡稳定性的合理性。刘新荣等（2016）从能量角度出发，提出基于能量突变的强度折减法失稳判据。张友利等（2020）、崔笑等（2020）、李志平等（2016）、华成亚等（2016）提出塑性应变能判据应用在边坡动力稳定分析中，并用关键点的残余位移突变判据证明其在边坡动力稳定分析中的可行性和计算精度，在文章中。介玉新（2023）提出相对失稳加速度判别边坡稳定性的方法，指出最小安全系数对应的临界滑动面不一定是最先发生滑动的破坏面，失稳加速度临界滑动面可能最先滑动。刘金龙等（2005）在文献建议联合采用特征点处的位移是否突变和塑性区是否贯通作为边坡的失稳判据，动力作用下边坡失稳判据的选取相对更加复杂。
塑性区反映了土体的状态，当地震作用下边坡土体中出现连续贯通的塑性区时，说明边坡可能发生失稳，但是仅根据塑性区贯通判断边坡是否失稳可能过于保守。因为在实际情况中，即使塑性区贯通，边坡也可能通过内部的应力调整而保持稳定。位移云图是一种基于边坡土体位移变化的评价方法，可以直观地反映出地震作用后土坡的残余位移情况和破裂面形态，该方法能够反映出地震作用下边坡的动态响应。因此，通过塑性区贯通和位移云图的联合评价，能够从两个角度全面考虑边坡的应力应变状态和位移变化情况，从而更快捷准确地判断边坡是否处于失稳状态。本文综合上述判据的特点及差异，提出采用以塑性区贯通为前提，结合土坡呈现出的“彩虹状”位移云图，联合作为土坡动力作用下整体失稳的判据。
1. 彩虹状位移云图失稳判据提出
在地震诱发土坡滑坡的实际工程案例中，发现滑动面与圆弧滑动面相近，通常将其近似为圆弧面来考虑（任永强等，2011；邓东平等，2012；邢洋等，2021）。在理论分析中，各种条分法均是根据瑞典圆弧法（整体圆弧法）发展起来的，瑞典圆弧法原始的求解思想是将整个滑动土体作为一个整体，假设土体均质，滑动面为圆弧滑动面，土体呈刚性转动，在滑面上土体处于极限平衡状态，各力对圆心O的力矩平衡。假定土体单元为不变形刚体，最终建立平衡方程和圆弧滑面力矩平衡方程。当滑坡体沿圆弧滑动面发生相对滑动时，总是沿着所处位置与圆心的这一半径圆弧滑动，首先滑体发生滑动的原因是滑体的下滑力矩MS超过了抗滑力矩MR，如公式（1）、（2）所列。
$$ {M}_{S}＝Wd\text{，} $$ (1) $$ {M}_{\mathrm{R}}＝{\int }_{0}^{L}{\tau }_{f}dl\cdot R \text{，} $$ (2) 式中：W为滑体重力，d为滑坡体重心与圆心的距离，τf为抗剪强度，l为滑动面长度，R为圆弧滑动面半径。
在上述理论和实际工程案例的基础上，本文提出的“彩虹状”位移云图失稳判据是基于地震作用下土坡滑动面为圆弧面的假定。滑坡体以O点为圆心产生整体滑移或转动，如图1所示。
当滑坡体整体转动θ角度时，滑移面上的点C移动到C′的位置，其位移为：
$$ {l}_{cc{'}}＝\alpha R\text{，} $$ (3) 式中：lcc′为滑动面点的位移，α为在弧度制下所对的弧数，R为圆弧滑动面的半径。
在本研究的模拟中，考虑了不同的地震动特点，地震动可能会影响位移云图的数值大小和范围，但不会改变彩虹状位移云图的基本特征。基于上述理论推理和数值模拟结果，对结果进行分析后得出边坡失稳的特征，即当边坡整体失稳破坏时的位移云图应满足下面三个条件：
1） 滑坡体内的土体单元相对于边坡内部稳定区沿圆弧滑动面发生相对滑动。边坡内部稳定区与滑坡体在数值模拟的永久位移云图中应呈现出明显的圆弧形界限。
2） 滑坡体位移沿着顺坡向呈现出圆弧形的带状分布。分层明显，类似彩虹状，因此将其定义为彩虹状位移云图。
3） 滑坡体各层位移从坡面指向坡体内部有先增大再突变减小的现象，最大值的层带即为边坡滑动面。
例如，图2分别为相邻两个折减系数对应的土坡地震永久位移响应云图。由图可知，此时滑坡体内部土体单元的位移值小于0.01 m，位移值较小，且同一半径圆弧形条带土体单元的位移量差异较大，并未呈现出彩虹状，表明滑坡体没有沿圆弧滑动面发生整体滑动，因此土坡处于局部破坏状态。
![]()
图 2 边坡破坏位移云图（a）局部破坏位移云图；（b）整体破坏彩虹状位移云图Figure 2. Cloud map of slope failure displacement（a） Localized damage displacement map；（b） Overall damage rainbow displacement map将边坡继续折减到图2b，位移云图呈现出明显的圆弧形边界，满足上述三个条件（1），可认为图2b中土坡发生整体失稳破坏。
2. 算例分析
2.1 模型参数
本文采用FLAC3D软件进行数值模拟，为研究地震作用下土坡失稳判据，建立边坡模型前缘长40 m，高40 m，坡顶后缘长120 m，高60 m，边坡坡高为20 m，以坡角45°的均质土坡模型为例，同时在土坡模型的坡脚、坡面中点以及坡顶处布设监测点记为P1、P2、P3，网格划分如图3所示。
本文土坡模型材料参数选取四川地区滑坡体的土体类型及相关数据，土体类型为西南地区粘土，土体物理力学参数如表1所示（曲宏略，2015；工程地质手册，2018）。
表 1 边坡土体参数Table 1. Slope Soil Parameters参数 密度
/（kg·m−3）体积模量
/MPa剪切模量
/MPa抗拉强度
/kPa粘聚力
/kPa内摩擦角
/°数值 1 900 70 37 4 32 24 在模拟地震过程中，对于均质土坡这类简单模型，局部阻尼与实际情况接近（言志信等，2011），且局部阻尼不需要估计系统的自振频率，因此更为简单有效，故本文阻尼的形式为局部阻尼。对于岩土体材料，临界阻尼比取值范围一般为2%—5% （张兆鹏，2018）。本文的研究地区及土体类型与文献（曲宏略，2015）相同，且土体参数相近，因此同样采用5%的临界阻尼比来近似表征土体在地震波传播过程中的阻尼作用，通过式（4）计算可得阻尼系数为0.157 （言志信等，2010）。
$$ \xi ＝\text{π} {D}_{s} $$ (4) 式中：ξ为局部阻尼系数，Ds为临界阻尼比，$\text{π} $取3.14。
选取Trinidad地震动，时长21.41 s，峰值0.08 g，计算步数为2 141步，步长0.01 s，加速度、速度及位移时程曲线如图4所示。本文先对地震动进行了滤波处理和基线校正，然后从模型底部竖向输入水平向地震动，为了避免边界的地震波反射，模型周围施加自由场边界条件，底部采用静态边界条件，此时需要将加速度、速度时程转换成应力时程后，再施加到模型底部。在模型底部设置监测点，与输入地震动的加速度时程曲线吻合，说明地震波输入正确。
![]()
图 4 Trinidad地震动加速度、速度及位移时程曲线（a） 加速度时程曲线；（b） 速度时程曲线；（c） 位移时程曲线Figure 4. Trinidad Ground Motion Acceleration，Velocity，and Displacement Time History Curve（a） Acceleration time-course curves；（b） Velocity time-course curves；（c） Displacement time-course curves2.2 动力有限元强度折减法
本文采用FLAC显式差分法求解微分方程，首先将计算区域离散化为若干单元。在荷载作用下，通过时间步长的有限差分形式计算节点速度和相对位移，进而求出应变和应力。通过不断迭代，求解节点的不平衡力，直至达到平衡状态（陈育民，徐鼎平，2009）。
动力力学平衡方程为：
$$ \boldsymbol{M}\left\{\ddot{u}\right\} ＋ \boldsymbol{C}\left\{\dot{u}\right\} ＋ \boldsymbol{K}\left\{u\right\}＝\left\{F （ t ） \right\} $$ (5) 式中：M为结构的质量矩阵；C为结构的阻尼矩阵；K为结构的刚度矩阵；$ \left\{\ddot{u}\right\} $为结构的加速度列阵，$ \left\{\dot{u}\right\} $为结构的速度列阵，$ \left\{u\right\} $为结构的位移列阵，{F（t）}为结构的节点荷载列阵。
当动荷载为输入地震荷载时，基本力学运动方程可写为：
$$ \boldsymbol{M}\left\{\ddot{u}\right\} ＋ \boldsymbol{C}\left\{\dot{u}\right\} ＋ \boldsymbol{K}\left\{u\right\}＝-\boldsymbol{M}\left\{{\ddot{u}}_{g} （ t ） \right\} $$ (6) 式中，$ {\ddot{u}}_{g} （ t ） $为输入地震加速度。
地震是一种随时间变化的复杂荷载作用，边坡岩土体在地震作用下很快会进入弹塑性状态（张江伟，2016）。边坡的稳定性安全系数，即边坡的安全储备系数（李德生，徐颖，2012）。在动力作用下边坡的研究方法中，郑颖人等（2005）提出的有限元强度折减动力分析法，在不假定滑面的情况下，既能得出边坡安全系数，又能反映岩土体的动力特性。在不断试算的基础上人为判定边坡是否失稳。在数值分析有限元计算的过程中，强度折减法指通过逐渐提高折减系数F，相应地逐渐减小土体的抗剪强度指标，直至边坡处于极限平衡的临界状态。通过失稳判据来判断此时土坡所处的状态，如果土坡失稳破坏，则减小折减系数F，反之，增大折减系数F。
$$ {c}{{'}}＝\frac{c}{F}，$$ (7) $$ \mathrm{t}\mathrm{a}\mathrm{n}{\varphi }{{'}}＝\frac{\mathrm{t}\mathrm{a}\mathrm{n}\varphi }{F}，$$ (8) 摩尔—库伦屈服准则对边坡内部滑动面形成机理进行了较详细的说明，不论土体内部应力路径如何变化，只要岩土体试样某一斜截面上的法向正应力和切向剪应力满足摩尔—库伦强度破坏包线方程式（9）或满足主应力形式的摩尔—库伦破坏包线方程式（10），则该试样就会沿着此斜面发生剪切破坏（张坤勇等，2017）。
$$ \tau ＝c ＋ \sigma \mathrm{t}\mathrm{a}\mathrm{n}\varphi \text{，} $$ (9) 式中：τ为土体的剪切强度；c为黏聚强度；σ为主应力；φ为内摩擦角。
$$ {\sigma }_{1}－{\sigma }_{3}＝2c\,\, \mathrm{c}\mathrm{o}\mathrm{s}\varphi ＋ （ {\sigma }_{1} ＋ {\sigma }_{3} ） \mathrm{s}\mathrm{i}\mathrm{n}\varphi $$ (10) 式中：σ1为大主应力；σ3为小主应力。
图5为摩尔库伦破坏包线图。
$$ \chi ＝\frac{2c\,\, \mathrm{cos}\varphi ＋ （ {\sigma }_{1} ＋ {\sigma }_{3} ） \mathrm{sin}\varphi － （ {\sigma }_{1}－{\sigma }_{3} ） }{2} ，$$ (11) 式中：χ的实际物理意义为莫尔圆与强度包线之间的最短距离。当χ＞0 时，破坏包线与莫尔圆相离，单元未破坏；当χ≤0 时，破坏包线与莫尔圆相切或相交，单元发生破坏。当潜在滑动面上所有土体单元的应力差χ≤0 时，即为边坡的极限平衡状态。
2.3 土坡地震安全系数计算
通过对模型进行多次折减计算，当折减系数F＝1.25时，如图6a，最终时刻的位移云图并未出现彩虹状，而是边坡下部位移较大，上部位移量较小，处于局部失稳状态；当F＝1.26时，如图6b，最终时刻的位移云图呈现出彩虹状，说明此时边坡处于整体失稳状态，则边坡的强度折减安全系数可取为F＝1.25。
![]()
图 6 位移云图（a） F＝1.25时位移云图；（b） F＝1.26时位移云图Figure 6. Displacement Cloud Map（a） Displacement cloud at F＝1.25；（b） Displacement cloud at F＝1.263. 对比其他判据结果
为了验证本文所提出方法的准确性，对比计算了其他不同判据下得到的安全系数，并对其差异性进行阐述。其中，数值软件计算不收敛这一判据对于FLAC3D来说可行性较差，在模拟计算中很难出现不收敛现象，因此在文中并未对此判据进行计算、讨论。
3.1 塑性区贯通判据
图7是边坡模型计算后的塑性区云图，土体单元由于受到剪切或者张拉作用发生剪切破坏和张拉破坏，当破坏单元由坡脚贯通至坡顶时认为边坡失稳。从图7可以看到，当F＝1.23时塑性区未贯通，在F＝1.24时塑性区贯通，故强度折减安全系数F＝1.23。
![]()
图 7 塑性区云图（a） F＝1.23时塑性区云图；（b） F＝1.24时塑性区云图Figure 7. Cloud Map of Plastic Zone（a） Cloud map of the plastic zone at F＝1.23；（b） Cloud map of the plastic zone at F＝1.243.2 位移突变判据
位移突变判据通常取坡脚、坡面、坡顶的特征点的最终位移与折减系数的关系曲线变化来判别边坡是否失稳，当折减系数的增大到某一特定值时，该点的最终位移突变，则认为边坡发生失稳破坏，对应的折减系数即为边坡的稳定系数。在研究特征点位移量突变这一判据时，多数采用坡顶的水平位移突变作为特征点位移判据，本文取坡脚、坡面中点以及坡顶的监测点，同时监测特征点水平向与竖直向位移。由图8可以看出，初期随着折减系数的增加，P1，P2，P3的水平向位移和竖直向位移都呈增长趋势。当折减系数增大到1.25时，P2，P3水平和竖向位移同时开始明显增大；当折减系数1.26时，位移量同时发生陡增，位移突变在1.26且继续发展。故边坡的强度折减安全系数可判定1.24—1.26之间。
![]()
图 8 强度折减系数与特征点位移量关系曲线（a） 特征点水平x方向位移与强度折减系数关系曲线；（b） 特征点水平z方向位移与强度折减系数关系曲线Figure 8. Relation Curve between Strength reduction Coefficient and Displacement of Characteristic Points（a） Characteristic point horizontal x-direction displacement versus strength reduction factor curve； （b） Characteristic point horizontal z-direction displacement versus strength reduction factor curve3.3 位移时程曲线判据
图例中1、2、3分别对应P1，P2，P3，地震作用下边坡监测点水平方向的位移曲线，如图9所示。在折减系数F＝1.24时，监测点的水平向位移随着地震动的进行在0点附近规律性变化浮动，并出现一定永久位移，接着继续对土体参数折减，在折减系数F＝1.25下监测点的位移时程曲线可以看出，位移曲线已经发生了一定程度的偏移，在地震过程中不能再回到0点附近，地震结束后产生了一定的永久残余位移，此时边坡应处于极限平衡或临界失稳状态。当折减系数F＝1.26时，上述现象更加明显，监测点位移在原有位置上产生了较大的偏离，故认为边坡的安全系数在1. 24—1.26之间。
![]()
图 9 监测点位移曲线（a） F＝1.24时监测点位移曲线；（b） F＝1.25时监测点位移曲线；（c） F＝1.26时监测点位移曲线Figure 9. Displacement Curve of Monitoring Points（a） Displacement curve of monitoring point at F＝1.24；（b） Displacement curve of monitoring point at F＝1.25； （c） Displacement curve of monitoring point at F＝1.264. 讨论与结论
将四类失稳判据得到的稳定系数列于表2中。
表 2 不同判据下的安全系数Table 2. Safety Factors under Different Criteria判据
类别塑性区
贯通特征点
位移突变位移时程
曲线判据位移云图
彩虹状安全系数 1. 23 1. 24—1.26 1. 24—1.26 1. 25 通过表2中对比分析不同判据下边坡的安全系数可以发现，采用特征点位移突变、位移时程曲线和位移云图彩虹状判据得出的安全系数差距不大，说明了本文提出的判据与此前的三类判据具有一致性，也验证了其可靠性。郑颖人等提到的塑性区贯通是边坡土体破坏的必要非充分条件，本文所得结果也可以证明这点，以塑性区贯通为判据得出的安全系数相对较小，与其他判据相比误差为1.6%，出现这种差异的原因可能与网格划分的精密程度与计算精度有关。动力作用下的边坡失稳较为复杂，若采用特征点最终位移突变或者位移时程突变作为判据则需要多次折减系数计算，对于位移监测点是否陡增，更多地依赖于研究者主观上的判定，以此作为判据缺乏说服力。
在采用传统的边坡动力失稳识别方法时，往往存在主观性强、受模拟软件影响的缺陷。本文提出了“彩虹状位移云图”的概念，将边坡滑动面与位移云图相关联，实现了一种新颖且直观的边坡失稳识别方法。通过与传统判据的对比，证明了彩虹状位移云图判据求得边坡安全系数的可行性和准确性，且物理意义明确，操作简便。这使得复杂的数值模拟结果进行解析变得更为简单，提升了工程实践中的效率。
通过上述研究，可得到如下结论：
1） 采用塑性区贯通、特征点最终位移突变、位移时程曲线和彩虹状位移云图四种判据得出的安全系数差距不大，其中采用位移突变判据得到的安全系数具有主观差异性，可取范围标值。
2） 彩虹状位移云图的出现可作为地震作用下土坡整体失稳破坏的判据，物理意义明确，操作简便。同时，也可结合塑性区贯通等其他判据来确认安全系数的准确性。
3） 用特征点位移突变判据时，在选择特征点时应在坡面多选几处，坡脚处的监测点可能会因处于剪出口在其上方而导致结果不准确。
本文的研究对象为简单均质土坡，验证了本文提出的彩虹状位移判据的准确可靠性，对于岩质边坡及多级分层的非均质土坡等还有待进一步研究。
-
![]()
图 10 古构造应力场中燕山中期（上）、晚期（下）的最大主应力（a，c）和最小主应力（b，d）的数值模拟结果
Figure 10. Numerical simulation results of the maximum principal stress （a，c） and minimum principal stress （b，d） in the middle and late stages of the Middle Yanshanian
![]()
图 1 武隆地区区域位置图
（a） 武隆及邻区地质构造图；（b） 龙马溪组底界构造图；（c） 沿AA′测线的地震解释剖面
Figure 1. Regional location map of Wulong area
（a） Geological tectonic settings of Wulong and its adjacent areas；（b） Structural map of the bottom boundary of the Longmaxi Formation；（c） Seismic interpretation section along the seismic survey line AA′
![]()
![]()
图 3 武隆地区及周缘的背向斜轴迹走向（a）及构造应力方向玫瑰花图（b）
Figure 3. The strike of the anticlinal and synclinal axis traces （a） and rose diagram of the tectonic stress direction （b） of Wulong area and its periphery
![]()
图 4 武隆地区及周缘的野外实测点R1 （a），R2 （b），R3 （c），R4 （d），R5 （e）和R6 （f）处的共轭剪节理露头
Figure 4. Outcrops of conjugate shear joints at the field measurement points R1 （a），R2 （b），R3 （c），R4 （d），R5 （e） and R6 （f） in Wulong area and its surrounding areas
![]()
![]()
图 6 武隆地区志留系龙马溪组底界的中燕山中期（a）和晚期（b）的古断裂展布图
Figure 6. Distribution of paleo-faults in the middle （a） and late （b） stages of the Middle Yanshanian at the bottom boundary of the Silurian Longmaxi Formation in Wulong area
![]()
图 7 武隆地区志留系龙马溪组地层的弹性模量（a）和泊松比（b）分布图
Figure 7. Distribution of elastic modulus （a） and Poisson’s ratio （b） of Silurian Longmaxi Formation in Wulong area
![]()
图 8 中燕山中期（a）和晚期（b）有限元模型的网格单元划分
Figure 8. Mesh element division of finite element models in the middle （a） and late （b） stages of the Middle Yanshanian
![]()
图 9 中燕山中期（a）和晚期（b）地质模型的边界条件示意图
Figure 9. Schematic diagram of the boundary conditions of the geological models of the middle （a） and late （b） stages of the Middle Yanshanian
![]()
图 11 断裂带周缘裂缝发育模式图
Figure 11. Fracture development pattern at the periphery of the fault zone
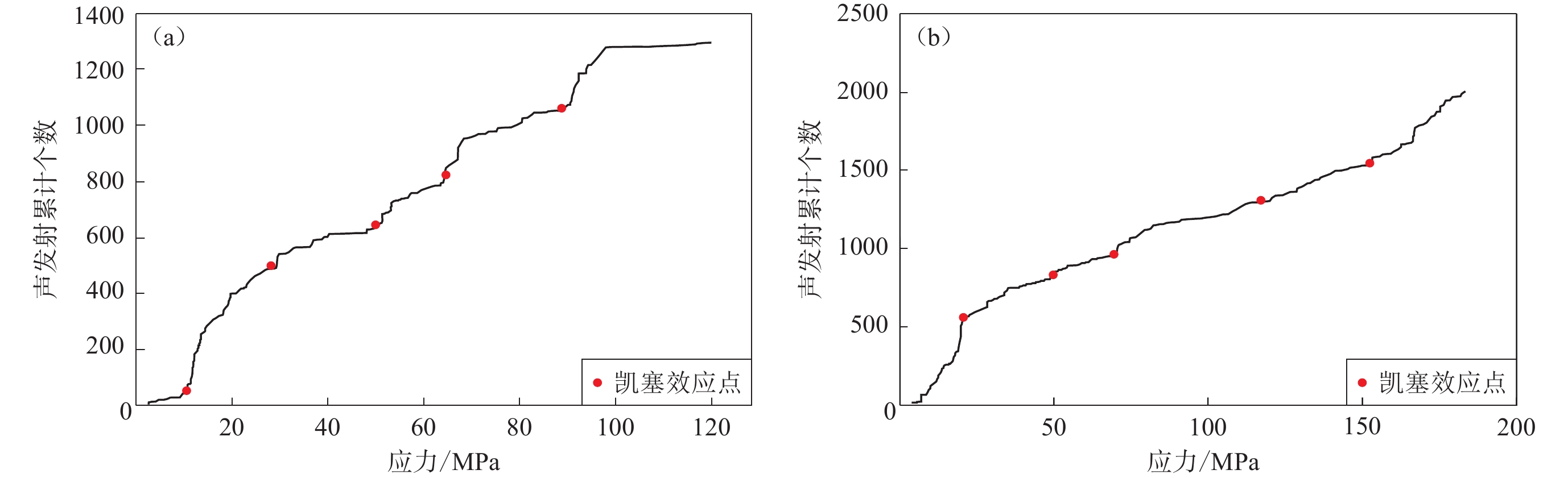
表 1 样品K1和K2的岩石声发射实验结果
Table 1 Results of rock acoustic emission experiments for the samples K1 and K2
样品
编号样品采集
层位早燕山期 中燕山早期 中燕山中期 中燕山晚期 喜山期 σ1/MPa σ3/MPa σ1/MPa σ3/MPa σ1/MPa σ3/MPa σ1/MPa σ3/MPa σ1/MPa σ3/MPa K1 T1j 15.12 7.71 33.53 22.68 150.18 73.14 120.55 40.94 71.70 48.65 K2 T1j 31.16 7.39 50.17 33.06 153.81 141.68 124.30 86.38 109.07 34.65 注：σ1和σ3为最大、最小主应力，T1j为下三叠统嘉陵江组地层。样品测试实验室：成都市成华区东方矿产开发技术研究所；测试者：韦力；测试仪器：美国物理公司生产的AE声发射检测系统。 
下载: 导出CSV
表 2 武隆地区多个钻井目的层岩心的岩石力学参数
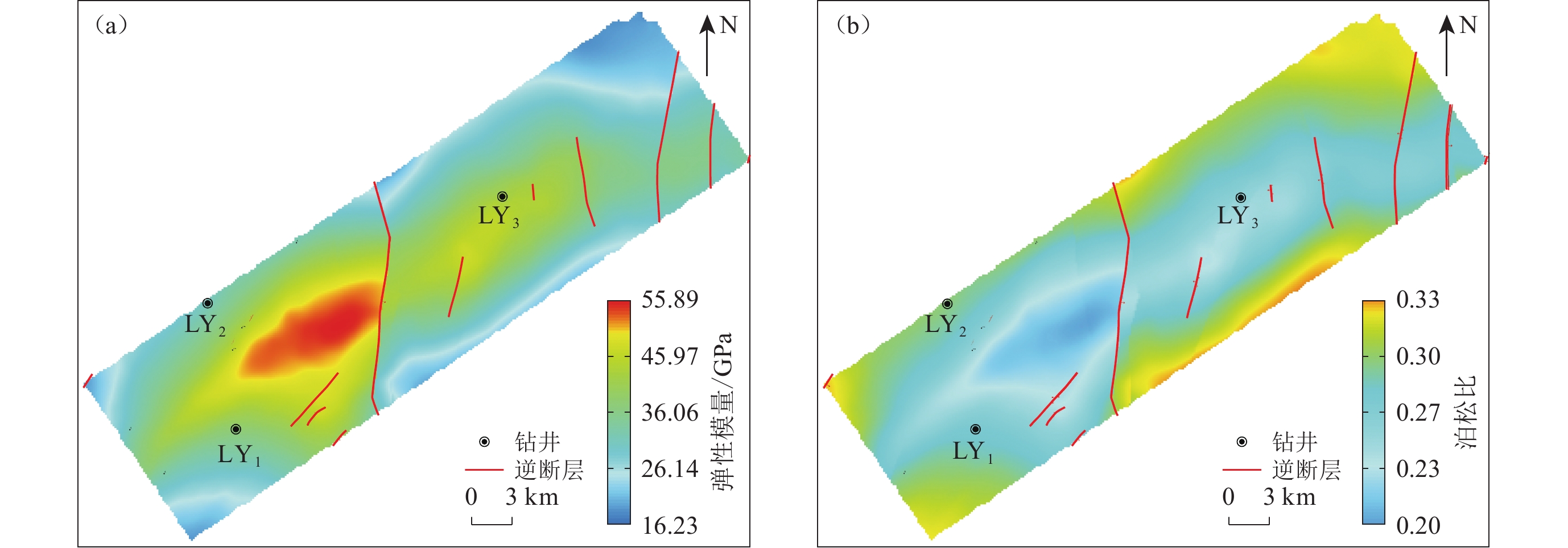
Table 2 Rock mechanical parameters of the target layer cores of several drills in Wulong area
样品编号 弹性模量/GPa 泊松比 LY1-1 37.14 0.266 LY1-2 36.70 0.276 LY2-1 31.34 0.292 LY2-2 32.57 0.279 LY3-1 43.22 0.241 LY3-2 43.35 0.232 注：样本测试实验室：成都市成华区东方矿产开发技术研究所；测试者：韦力；测试仪器：美国物理公司生产的TAW-1 000微机电液伺服控制岩石三轴应力试验机。
下载: 导出CSV
表 3 中燕山中期和晚期地质模型的岩石力学参数
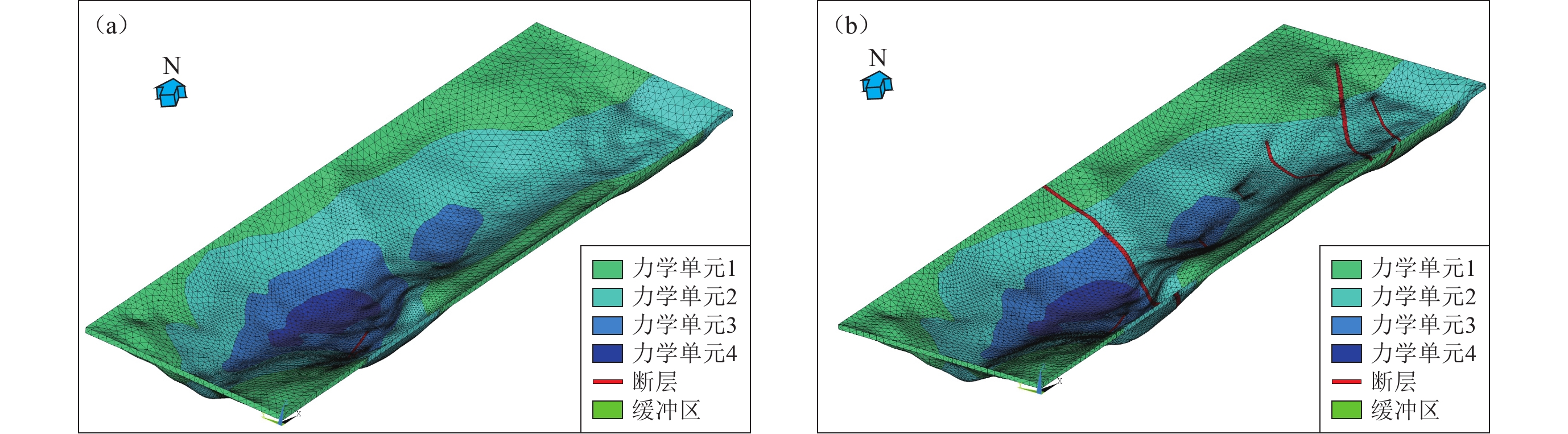
Table 3 The rock mechanical parameters of the geological models in the middle and late stages of the Middle Yanshanian
岩石物理参数 弹性模量/GPa 泊松比 力学单元1 43.21 0.23 力学单元2 39.53 0.26 力学单元3 32.51 0.30 力学单元4 28.52 0.32 断层 19.51 0.35 缓冲区 3.62 0.28
下载: 导出CSV
表 4 研究区古构造应力场数值模拟结果对比
Table 4 Comparison of numerical simulation results of paleotectonic stress field in the study area
时期 实测点 最大主应力 最小主应力 差应力 实验结果
/MPa模拟结果
/MPa差值
/MPa实验结果
/MPa模拟结果
/MPa差值
/MPa实验结果
/MPa模拟结果
/MPa差值
/MPa中燕山中期 R1 150.18 152.63 −2.45 73.14 75.71 −2.57 77.04 76.92 0.12 R2 153.81 152.69 1.12 124.30 120.23 4.07 29.51 32.46 −2.95 中燕山晚期 R1 120.55 118.46 2.09 40.94 42.17 −1.23 79.61 76.29 3.32 R2 141.68 139.98 1.70 86.38 83.97 2.41 55.30 56.01 −0.71
下载: 导出CSV
-
丁文龙,曾维特,王濡岳,久凯,王哲,孙雅雄,王兴华. 2016. 页岩储层构造应力场模拟与裂缝分布预测方法及应用[J]. 地学前缘,23(2):63–74. Ding W L,Zeng W T,Wang R Y,Jiu K,Wang Z,Sun Y X,Wang X H. 2016. Method and application of tectonic stress field simulation and fracture distribution prediction in shale reservoir[J]. Earth Science Frontiers,23(2):63–74 (in Chinese).
董敏,郭伟,张林炎,吴中海,马立成,董会,冯兴强,杨跃辉. 2022. 川南泸州地区五峰组—龙马溪组古构造应力场及裂缝特征[J]. 岩性油气藏,34(1):43–51. Dong M,Guo W,Zhang L Y,Wu Z H,Ma L C,Dong H,Feng X Q,Yang Y H. 2022. Characteristics of paleotectonic stress field and fractures of Wufeng-Longmaxi formations in Luzhou area,southern Sichuan Basin[J]. Lithologic Reservoirs,34(1):43–51 (in Chinese).
葛勋,汤济广,赵培荣,唐永,许启鲁. 2023. 渝东南彭水地区页岩储层构造应力场模拟解析[J]. 西南石油大学学报(自然科学版),45(5):27–42. Ge X,Tang J G,Zhao P R,Tang Y,Xu Q L. 2023. Simulation and analysis of tectonic stress field of shale reservoir in Pengshui area,southeast Chongqing[J]. Journal of Southwest Petroleum University (Science &Technology Edition),45(5):27–42 (in Chinese).
顾悦,陈世桢,贾小瑛,张竹如. 1983. 关于节理裂隙面产状随地层复平变化的一种简捷计算方法[J]. 贵州工学院学报,(3):24–28. Gu Y,Chen S Z,Jia X Y,Zhang Z R. 1983. A simple method for calculating the occurrence of joint fracture surface with the change of strata releveling[J]. Journal of Guizhou Institute of Technology,
(3):24–28 (in Chinese). 郭彤楼,何希鹏,曾萍,高玉巧,张培先,何贵松. 2020. 复杂构造区页岩气藏地质特征与效益开发建议:以四川盆地及其周缘五峰组—龙马溪组为例[J]. 石油学报,41(12):1490–1500. Guo T L,He X P,Zeng P,Gao Y Q,Zhang P X,He G S. 2020. Geological characteristics and beneficial development scheme of shale gas reservoirs in complex tectonic regions:A case study of Wufeng-Longmaxi Formations in Sichuan Basin and its periphery[J]. Acta Petrolei Sinica,41(12):1490–1500 (in Chinese).
何利,宋春彦,谭钦银,程锦翔,王瑞华,杨贵来. 2017. 川东南武隆地区五峰组—龙马溪组页岩气形成条件及富集区分析[J]. 海相油气地质,22(3):47–56. He L,Song C Y,Tan Q Y,Cheng J X,Wang R H,Yang G L. 2017. Accumulation conditions and enrichment zones of shale gas in Wufeng-Longmaxi Formation in Wulong area of southeast Sichuan Basin[J]. Marine Origin Petroleum Geology,22(3):47–56 (in Chinese).
何梅朋. 2023. 武隆地区五峰组—龙马溪组优质浅层常压页岩储层发育特征及含气性影响因素[J]. 非常规油气,10(3):64–73. He M P. 2023. Development characteristics and gas-bearing factors of high-quality shallow ordinary-pressure shale reservoirs in Wufeng-Longmaxi Formation in Wulong area[J]. Unconventional Oil &Gas,10(3):64–73 (in Chinese).
何希鹏,何贵松,高玉巧,张培先,卢双舫,万静雅. 2018. 渝东南盆缘转换带常压页岩气地质特征及富集高产规律[J]. 天然气工业,38(12):1–14. He X P,He G S,Gao Y Q,Zhang P X,Lu S F,Wan J Y. 2018. Geological characteristics and enrichment laws of normal-pressure shale gas in the basin margin transition zone of SE Chongqing[J]. Natural Gas Industry,38(12):1–14 (in Chinese).
何希鹏,高玉巧,马军,张培先,何贵松,周頔娜,刘娜娜,孙斌. 2022. 重庆市武隆区黄莺乡五峰组—龙马溪组剖面沉积特征及地质意义[J]. 油气藏评价与开发,12(1):95–106. He X P,Gao Y Q,Ma J,Zhang P X,He G S,Zhou D N,Liu N N,Sun B. 2022. Sedimentary characteristics and geological significance of outcrop in Wufeng-Longmaxi Formation,Huangying Town,Wulong District,Chongqing[J]. Petroleum Reservoir Evaluation and Development,12(1):95–106 (in Chinese).
李东东. 2017. 武陵山区中新生代古构造应力场分析[D]. 北京:中国地质大学(北京):33−34. Li D D. 2017. Analysis of the Characteristics of Paleotectonic Stress in Area of Wuling Mount[D]. Beijing:China University of Geosciences (Beijing):33−34 (in Chinese).
李萧,吴礼明,王丙贤,胡秋媛,董大伟. 2021. 渝东南地区龙马溪组构造应力场数值模拟及裂缝有利区预测[J]. 地质科技通报,40(6):24–31. Li X,Wu L M,Wang B X,Hu Q Y,Dong D W. 2021. Numerical simulation of tectonic stress field and prediction of fracture target in the Longmaxi Formation,southeastern Chongqing[J]. Bulletin of Geological Science and Technology,40(6):24–31 (in Chinese).
李艺豪. 2023. 川东—川鄂渝黔地块武隆地区地质构造与大型滑坡响应关系研究[D]. 北京:中国地质科学院:49−50. Li Y H. 2023. Study on the Relationship Between Geological Structure and Large Landslide in Wulong Area of the Eastern Sichuan Block-ChuanEYuQian Block[D]. Beijing:Chinese Academy of Geological Sciences:49−50 (in Chinese).
刘金华. 2009. 油气储层裂缝形成、分布及有效性研究[D]. 青岛:中国石油大学(华东):88−89. Liu J H. 2009. Study on the Formation,Distribution and Effectiveness of Reservoir Fractures With Oil and Gas[D]. Qingdao:China University of Petroleum (East China):88−89 (in Chinese).
马新华,谢军. 2018. 川南地区页岩气勘探开发进展及发展前景[J]. 石油勘探与开发,45(1):161–169. Ma X H,Xie J. 2018. The progress and prospects of shale gas exploration and exploitation in southern Sichuan Basin,NW China[J]. Petroleum Exploration and Development,45(1):161–169 (in Chinese).
梅廉夫,刘昭茜,汤济广,沈传波,凡元芳. 2010. 湘鄂西—川东中生代陆内递进扩展变形:来自裂变径迹和平衡剖面的证据[J]. 地球科学:中国地质大学学报,35(2):161–174. Mei L F,Liu Z Q,Tang J G,Shen C B,Fan Y F. 2010. Mesozoic intra-continental progressive deformation in western Hunan-Hubei-eastern Sichuan Provinces of China:Evidence from apatite fission track and balanced cross-section[J]. Earth Science:Journal of China University of Geosciences,35(2):161–174 (in Chinese).
聂海宽,包书景,高波,边瑞康,张培先,武晓玲,叶欣,陈新军. 2012. 四川盆地及其周缘下古生界页岩气保存条件研究[J]. 地学前缘,19(3):280–294. Nie H K,Bao S J,Gao B,Bian R K,Zhang P X,Wu X L,Ye X,Chen X J. 2012. A study of shale gas preservation conditions for the Lower Paleozoic in Sichuan Basin and its periphery[J]. Earth Science Frontiers,19(3):280–294 (in Chinese).
彭瑞,孟祥瑞,赵光明,董春亮,左超. 2015. 不同岩性岩石声发射地应力测试及其应用[J]. 中南大学学报(自然科学版),46(9):3377–3384. Peng R,Meng X R,Zhao G M,Dong C L,Zuo C. 2015. Acoustic emission insitu stress testing of different lithology rock and its application[J]. Journal of Central South University (Science and Technology),46(9):3377–3384 (in Chinese).
任浩林,刘成林,刘文平,杨熙雅,李文研. 2020. 四川盆地富顺—永川地区五峰组—龙马溪组应力场模拟及裂缝发育区预测[J]. 地质力学学报,26(1):74–83. Ren H L,Liu C L,Liu W P,Yang X Y,Li W Y. 2020. Stress field simulation and fracture development prediction of the Wufeng Formation-Longmaxi Formation in the Fushun-Yongchuan block,Sichuan Basin[J]. Journal of Geomechanics,26(1):74–83 (in Chinese).
苏文博,李志明,Ettensohn F R,Johnson M E,Huff W D,王巍,马超,李录,张磊,赵慧静. 2007. 华南五峰组—龙马溪组黑色岩系时空展布的主控因素及其启示[J]. 地球科学:中国地质大学学报,32(6):819–827 Su W B,Li Z M,Ettensohn F R,Johnson M E,Huff W D,Wang W,Ma C,Li L,Zhang L,Zhao H J. 2007. Distribution of black shale in the Wufeng-Longmaxi Formations (Ordovician-Silurian),South China:Major controlling factors and implications[J]. Earth Science:Journal of China University of Geosciences,32(6):819–827 (in Chinese).
孙斌,鞠玮,杨敏芳,杨兆彪,刘洪林,王胜宇. 2021. 滇东北地区煤储层现今地应力分布特征及渗透性预测[J]. 中国煤炭地质,33(4):44–50. Sun B,Ju W,Yang M F,Yang Z B,Liu H L,Wang S Y. 2021. Distribution of present-day in-situ stresses and permeability prediction within coal reservoirs of northeastern Yunnan region[J]. Coal Geology of China,33(4):44–50 (in Chinese).
孙晓庆. 2008. 古构造应力场有限元数值模拟的应用及展望[J]. 断块油气田,15(3):31–33. Sun X Q. 2008. Present situation and prospect of application for finite element numerical simulation of paleotectonic stress fields[J]. Fault-Block Oil and Gas Fields,15(3):31–33 (in Chinese).
孙玥. 2020. 四川盆地龙马溪组页岩气富集的控制因素及评价[D]. 北京:中国石油大学(北京):45−46. Sun Y. 2020. Comparative Analysis and Periphery of Shale Gas Enrichment and Depletion Factors in the Wufeng-Longmaxi Formation in the Sichuan Basin[D]. Beijing:China University of Petroleum (Beijing):45−46 (in Chinese).
王珂,戴俊生,冯阵东,解艳雪,樊阳,王媛,赵力彬. 2013. 砂泥岩间互地层等效岩石力学参数计算模型及其应用[J]. 地质力学学报,19(2):143–151. Wang K,Dai J S,Feng Z D,Xie Y X,Pan Y,Wang Y,Zhao L B. 2013. Calculation model of equivalent rock mechanical parameters of sand-mud interbed and its application[J]. Journal of Geomechanics,19(2):143–151 (in Chinese).
王珂,戴俊生,冯建伟,王俊鹏,李青. 2014. 塔里木盆地克深前陆冲断带储层岩石力学参数研究[J]. 中国石油大学学报(自然科学版),38(5):25–33. Wang K,Dai J S,Feng J W,Wang J P,Li Q. 2014. Research on reservoir rock mechanical parameters of Keshen foreland thrust belt in Tarim Basin[J]. Journal of China University of Petroleum (Edition of Natural Science),38(5):25–33 (in Chinese).
王琳. 2020. 渝东南地区重点层系页岩气富集条件及可采资源[D]. 北京:中国地质大学(北京):63−64. Wang L. 2020. Enrichment Conditions and Recoverable Resources of Shale Gas of the Focus Layers in Southeast Chongqing[D]. Beijing:China University of Geosciences (Beijing):63−64 (in Chinese).
吴航. 2019. 川东地区中-新生代构造隆升过程研究[D]. 北京:中国石油大学(北京):126−127. Wu H. 2019. Meso-Cenozoic Tectonic Uplift Process of the Eastern Sichuan Basin[D]. Beijing:China University of Petroleum (Beijing):126−127 (in Chinese).
吴聿元,张培先,何希鹏,高玉巧,何贵松,孙斌,万静雅,高全芳,周頔娜. 2020. 渝东南地区五峰组—龙马溪组页岩岩石相及与页岩气富集关系[J]. 海相油气地质,25(4):335–343. Wu Y Y,Zhang P X,He X P,Gao Y Q,He G S,Sun B,Wan J Y,Gao Q F,Zhou D N. 2020. Lithofacies and shale gas enrichment of Wufeng Formation-Longmaxi Formation in southeastern Chongqing[J]. Marine Oil and Gas Geology,25(4):335–343 (in Chinese).
谢渊,丘东洲,王剑,汪正江,刘建清,余谦,李嵘,杨平,李旭兵. 2012. 雪峰山西侧盆山过渡带震旦系—下古生界油气远景区预测与评价[J]. 地质通报,31(11):1769–1780. Xie Y,Qiu D Z,Wang J,Wang Z J,Liu J Q,Yu Q,Li R,Yang P,Li X B. 2012. Prediction and evaluation of the Sinian-Lower Paleozoic oil-gas prospective areas in the basin-mountain transitional region on the western side of the Xuefeng Mountain[J]. Geological Bulletin of China,31(11):1769–1780 (in Chinese).
徐政语,梁兴,王维旭,张介辉,王希有,舒红林,黄程,王高成,鲁慧丽,刘臣,张朝,李庆飞,徐鹤. 2016. 上扬子区页岩气甜点分布控制因素探讨:以上奥陶统五峰组—下志留统龙马溪组为例[J]. 天然气工业,36(9):35–43. Xu Z Y,Liang X,Wang W X,Zhang J H,Wang X Y,Shu H L,Huang C,Wang G C,Lu H L,Liu C,Zhang C,Li Q F,Xu H. 2016. Controlling factors for shale gas sweet spots distribution in the Upper Yangtze region:A case study of the Upper Ordovician Wufeng Fm−Lower Silurian Longmaxi Fm,Sichuan Basin[J]. Natural Gas Industry,36(9):35–43 (in Chinese).
薛亚东,高德利. 2000. 声发射地应力测量中凯塞点的确定[J]. 石油大学学报(自然科学版),24(5):1–3. Xue Y D,Gao D L. 2000. Determination of Kaiser point in measurement of geo-stress with acoustic emission[J]. Journal of the University of Petroleum,China,24(5):1–3 (in Chinese).
云露,高玉巧,高全芳. 2023. 渝东南地区常压页岩气勘探开发进展及下步攻关方向[J]. 石油实验地质,45(6):1078–1088. Yun L,Gao Y Q,Gao Q F. 2023. Progress and research direction of normal-pressure shale gas exploration and development in southeastern Chongqing[J]. Petroleum Geology &Experiment,45(6):1078–1088 (in Chinese).
曾维特. 2014. 渝东南地区下古生界页岩储层裂缝发育特征与分布预测研究[D]. 北京:中国地质大学(北京):37−38. Zeng W T. 2014. The Characteristics and Distribution Prediction of Fractures in Lower Paleozoic Shale,Southeast of Chongqing Area[D]. Beijing:China University of Geosciences (Beijing):37−38 (in Chinese).
张斗中,陈孔全,汤济广,庹秀松,马帅. 2024. 鄂西荆门地区志留系龙马溪组古构造应力场研究及裂缝预测[J]. 地球学报,45(2):217–231. Zhang D Z,Chen K Q,Tang J G,Tuo X S,Ma S. 2024. Paleotectonic stress field and fracture prediction of Silurian Longmaxi Formation in Jingmen area,western Hubei[J]. Acta Geoscientica Sinica,45(2):217–231 (in Chinese).
张海涛,张颖,何希鹏,高玉巧,张培先. 2018. 渝东南武隆地区构造作用对页岩气形成与保存的影响[J]. 中国石油勘探,23(5):47–56. Zhang H T,Zhang Y,He X P,Gao Y Q,Zhang P X. 2018. The effect of tectonism on shale gas formation and preservation in Wulong area,southeastern Chongqing[J]. China Petroleum Exploration,23(5):47–56 (in Chinese).
张胜利. 2010. 构造应力场模拟:有限元理论、方法和研究进展[J]. 西北地震学报,32(4):405–410. Zhang S L. 2010. Modeling of tectonic stress field:The theory,method and related research progress of the finite element method[J]. Northwestern Seismological Journal,32(4):405–410 (in Chinese).
张晓明,石万忠,徐清海,王任,徐壮,王健,王超,袁琪. 2015. 四川盆地焦石坝地区页岩气储层特征及控制因素[J]. 石油学报,36(8):926–939. Zhang X M,Shi W Z,Xu Q H,Wang R,Xu Z,Wang J,Wang C,Yuan Q. 2015. Reservoir characteristics and controlling factors of shale gas in Jiaoshiba area,Sichuan Basin[J]. Acta Petrolei Sinica,36(8):926–939 (in Chinese).
张颖,张海涛,何希鹏,高玉巧,张培先. 2018. 渝东南武隆地区龙马溪组页岩孔裂隙特征研究[J]. 西南石油大学学报(自然科学版),40(4):29–39. Zhang Y,Zhang H T,He X P,Gao Y Q,Zhang P X. 2018. Shale pore characteristics of Longmaxi Formation in Wulong area,southeastern Chongqing[J]. Journal of Southwest Petroleum University (Science &Technology Edition),40(4):29–39 (in Chinese).
赵奎,项威斌,曾鹏,杨道学,伍文凯,龚囱,杨贤达. 2021. 岩石声发射Kaiser效应研究现状及展望[J]. 金属矿山,(1):94–105. Zhao K,Xiang W B,Zeng P,Yang D X,Wu W K,Gong C,Yang X D. 2021. Research status and prospect of Kaiser effect in rock acoustic emission[J]. Metal Mine,(1):94−105 (in Chinese).
钟城,秦启荣,魏志红,李虎,邓毅,何威. 2018. 酒店垭—桑木场构造须家河组节理发育特征与应力场解析[J]. 现代地质,32(2):279–288. Zhong C,Qin Q R,Wei Z H,Li H,Deng Y,He W. 2018. Characteristics and tectonic stress field of joints in Xujiahe Formation of Jiudianya-Sangmuchang structure[J]. Geoscience, 32 (2):279−288 (in Chinese).
祖克威,曾大乾,程秀申,卓色强,李松峰. 2017. 普光地区嘉二段构造裂缝形成机理及预测[J]. 新疆石油地质,38(4):407–413. Zu K W,Zeng D Q,Cheng X S,Zhuo S Q,Li S F. 2017. Forming mechanism and prediction of structural fractures in the second member of Jialingjiang Formation in Puguang area[J]. Xinjiang Petroleum Geology,38(4):407–413 (in Chinese).
Camac B A,Hunt S P. 2009. Predicting the regional distribution of fracture networks using the distinct element numerical method[J]. AAPG Bulletin,93(11):1571–1583. doi: 10.1306/07230909040
Ding W L,Fan T L,Yu B S,Huang X B,Liu C. 2012. Ordovician carbonate reservoir fracture characteristics and fracture distribution forecasting in the Tazhong area of Tarim Basin,Northwest China[J]. J Petrol Sci Eng, 86 − 87 (3):62–70.
Ju W,Wang J L,Fang H H,Gong Y P,Zhang S J. 2017. Paleostress reconstructions and stress regimes in the Nanchuan region of Sichuan Basin,South China:Implications for hydrocarbon exploration[J]. Geosci J, 21 (4):553–564.
Kaiser P K,Moss A. 2022. Deformation-based support design for highly stressed ground with a focus on rockburst damage mitigation[J]. J Rock Mech Geotech Eng,14(1):50–66.
Li J J,Qin Q R,Li H,Zhao S X. 2022. Paleotectonic stress field modeling and fracture prediction of the Longmaxi Formation in the N216 well block,southern Sichuan basin,China[J]. Arab J Geosci,15(4):347.
Liu J S,Ding W L,Wang R Y,Yin S,Yang H M,Gu Y. 2017. Simulation of paleotectonic stress fields and quantitative prediction of multi-period fractures in shale reservoirs:A case study of the Niutitang Formation in the Lower Cambrian in the Cen’gong block,South China[J]. Mar Petrol Geol,84:289–310.
Tang Y,Yang F,Lü Q Q,Tang W J,Wang H K. 2018. Analysis of the tectonic stress field of SE Sichuan and its impact on the preservation of shale gas in Lower Silurian Longmaxi Formation of the Dingshan region,China[J]. J Geol Soc India,92(1):92–100. doi: 10.1007/s12594-018-0957-z
Zhang D Z,Tang J G,Chen K Q,Wang K M,Zhang P X,He G S,Tuo X S. 2022. Simulation of tectonic stress field and prediction of tectonic fracture distribution in Longmaxi Formation in Lintanchang area of eastern Sichuan Basin[J]. Front Earth Sci,10:1024748.
计量
- 文章访问数: 150
- HTML全文浏览量: 12
- PDF下载量: 60
