Study on the seismic damage mechanism of buildings on the river terrace in Wenchuan earthquake
-
摘要:
基于2008年汶川MS8.0地震现场的震害调查资料,揭示了河流阶地地形对建筑物震害分布的影响规律。阶地前缘冲洪积层较厚区域的建筑物遭受了严重的破坏,而阶地后缘坡积层较薄区域则相对受损较轻,总体上呈现出前缘震害显著大于后缘的特征。为研究该震害特征的机制,利用FLAC3D有限差分软件构建三维阶地模型,模拟分析其在脉冲荷载作用下的地震动响应。计算结果表明:各级阶地前缘的地震动水平均要显著高于后缘,同时随上覆土层增厚,加速度峰值、相对持时值、反应谱平台值以及放大系数均呈现上升趋势。而随阶地级数的减小,峰值、持时和反应谱特征周期却呈下降趋势,且级数的增加还会显著增强地震动低频成分的放大效应。因此阶地级数与上覆土层厚度是影响地震动响应的关键因素,高阶地和厚覆盖土层区域对地震动的放大效应更强,从而导致建筑物破坏严重。
Abstract:The seismic ground motion topographic effect, as an important research content in the field of seismic engineering, the study of the mechanism of complex terrain on the ground motion characteristics can provide basis for engineering seismic defense.A large number of post-earthquake site investigations have shown that the complexity of local terrain has a significant impact on the distribution of seismic damage, especially the irregular terrain can change the intensity and spectral characteristics of ground motion. As a type of local irregular topography widely found in nature, the unique geometric shape of river terraces can cause complex scattering and diffraction of seismic waves, resulting in differences of ground motion in its local areas, and then affecting the seismic damage degree of surrounding buildings.
Based on the on-site seismic damage investigation data of the river terraces in Wenchuan MS8.0 earthquake in 2008, it was found that buildings in the area with thicker alluvial at the front edge of the terrace suffered serious damage, while those in the area with thinner slope deposit at the rear edge of the terrace suffered relatively less damage. In general, the seismic damage at the front edge was significantly greater than that at the rear edge. At the same time, in order to study the mechanism of this seismic damage feature, the river terraces were selected as the research object, and the FLAC3D finite difference software was used to establish three-dimensional river terrace analysis models with different thicknesses of overburden soil layers, simulating and calculating the ground motion response under impulse loading, further revealing the influence law and internal mechanism of river terrace topography on ground motion characteristics and the distribution of seismic damage to buildings.
For the same level terrace, the peak values of the horizontal and vertical acceleration and the 90% energy duration all show an upward trend with the increase of the overlying soil thickness, reaching the maximum value at the front edge of the terrace and the transition point with the steep slope. Meanwhile, the ground motion level at the front edge of each terrace is significantly higher than that at the rear edge. As the terrace grade decreases, the peak values of the horizontal and vertical acceleration and the 90% energy duration at the corresponding area also gradually decrease. Similarly, the trend of the Fourier spectrum amplitude and ratio at different monitoring points on the same level terrace is basically consistent, but the amplitude and ratio increase gradually as the monitoring point approaches the edge of the terrace facing the steep slope and the front edge of the area with increased soil thickness.As the terrace grade increases (the terrain height rises), the natural frequency of the structure increases accordingly, thereby significantly enhancing the amplification effect of low-frequency ground motion. The characteristic period value, platform value, and platform amplification coefficient in the standard response spectrum of seismic acceleration for different monitoring points are all affected by the terrace grade and overlying soil thickness. The Tg value decreases gradually as the terrace grade decreases; the platform value increases as the overlying soil thickness at the front edge of the terrace increases; however, the platform amplification coefficient β value decreases as the terrace grade increases, and the amplification coefficient at the front edge of the terrace is significantly larger than that at the rear edge.
River terraces have a significant impact on the propagation of ground motion and the degree of seismic damage to buildings. The change of the thickness of the overlying soil layer leads to different distributions of building damage by affecting the amplification of ground motion, while the number of terrace grades exacerbates or mitigates the seismic damage by affecting the spectral characteristics and overall level of ground motion.Therefore, the number of terrace grades and overlying soil thickness are the key factors affecting ground motion response, and the amplification effect of ground motion is stronger in high terrace and thick covering soil layer, which leads to serious damage of buildings.
-
引言
地震动地形效应属于地震工程领域的重要研究内容,复杂地形对地震动特性的影响机制研究可以为工程抗震设防提供依据。二十世纪七十年代以来,国外学者Boore (1972)、Davis和West (1973)就已经开始系统地研究地形条件对地震动的影响。众多的震后现场调查表明,局部地形的复杂性对震害分布特征有显著影响,尤其是不规则地形能改变地震动的强度和频谱特性(刘晶波,1996;Bouckovalas,Papadimitriou,2005;Peng et al,2009)。河谷作为自然界中广泛存在的局部不规则地形,其独特的地形几何形态能够引发地震波复杂的散射和衍射现象,导致地震动在局部区域内产生差异,进而影响周边建筑物的震害程度(高玉峰等,2021)。历史上多次强震事件均有力地证明了河谷地形对震害的重要影响。例如,2005年巴基斯坦东部巴控克什米尔地区的MS7.8强烈地震中,位于Kaghan河谷谷地附近的巴拉考特镇遭受了毁灭性打击,超过90%的建筑物倒塌,造成了大量人员伤亡(曲国胜等,2008)。2008年中国四川攀枝花市仁和区与凉山州会理县交界的MS6.1地震中,沿河谷地带的震害严重、地形效应显著(肖文海,2009);汶川MS8.0特大地震中,位于流沙河阶地之上的汉源县城出现了罕见的远震高烈度异常现象(李平等,2016);此外,平武县南坝镇涪江、彭州小鱼洞镇湔江、什邡市石亭江等河流阶地上不同位置的震害情况呈现出显著差异(王伟,2011)。
河谷地形的地震动效应研究方法主要包括强震动观测、理论解析和数值模拟等。强震动观测方法是基于实际地震动记录开展研究(李平等,2016),观测对象为真实的自然地形,具有很好的直观性,但其应用受限于观测资料的获取难度和数量。理论解析则是基于波动理论,利用数学和力学的方法求解地震波在河谷地形下的响应,揭示地震波波形、入射角和入射频率以及地形几何参数对地震动特性的重要影响(李伟华,赵成刚,2003;董俊,赵成刚,2005;梁建文等,2005;韩铮,2006;高玉峰等,2022)。然而,理论解析方法的简化模型与实际情况存在差异,以及对计算能力的高要求,限制了其广泛应用。相比之下,数值模拟的灵活性和高精度,使其逐渐成为研究河谷地形效应的主流方法。国内外学者采用有限差分法(Boore,1972;Tessmer,Kosloff,1994;王铭锋等,2017)、有限元法(Kurita et al,2005;周国良等,2012;章小龙等,2017;孙纬宁等,2019;沈欣茹等,2023)及边界元法(林皋,关飞,1990;Liu et al,2018;何颖等,2019),深入研究了地震动在河谷场地下的传播与响应,揭示了河谷场地深宽比、覆盖层厚度、坡角以及输入地震动强度和入射角对地表地震动的影响规律。
河流阶地由于经历了地质演化作用过程,具有复杂的几何结构和厚度不均匀的沉积地层特征,目前这两种因素对地震动的耦合效应影响机制研究较少。本文基于2008年汶川特大地震河流阶地的现场震害调查资料,选取河流阶地作为研究对象,利用FLAC3D有限差分软件建立具有不同厚度覆盖土层的三维河流阶地分析模型,模拟计算其在脉冲荷载作用下的地震动响应,揭示了河流阶地地形对地震动特性的影响规律,进一步探讨了其对建筑物震害分布影响的内在机制。
1. 河流阶地的现场震害调查
在2008年汶川特大地震之后,对河流阶地上的建筑物震害情况进行了全面调查,收集了大量宝贵的现场资料。分析发现河流阶地不同位置处的覆盖层厚度存在显著差异,与相应位置建筑物的震害程度密切相关。具体而言,在冲洪积层较厚的阶地前缘区域,建筑物遭受了非常严重的破坏;相反,在阶地后缘与山体坡脚连接的洪积层较薄区域,建筑物的受损程度则相对较轻。总体上,阶地前缘的建筑物震害程度显著高于阶地后缘。
以平武县南坝镇为例,该镇坐落于涪江顺流右侧的河谷盆地之中,与断层的直线距离仅为4.2 km,处于地震烈度高达Ⅺ度的区域。在涪江的南岸,存在一处高于江面30 m左右的Ⅲ级冲洪积阶地,其底部由厚度超过20 m的冲洪积卵砾层和漂石层构成,地表为相对较薄的黏土层。调查结果显示,在阶地前缘,即靠近涪江一侧的建筑物,倒塌与破坏极为严重,如图1所示;而阶地后缘上覆层为较薄坡残积碎石层,该区域的建筑物大多保持完好或仅受轻微损害,如图2所示。
什邡市的蓥华镇则位于石亭江次流分支——竹溪河的右岸,处于Ⅸ度烈度区,且与主断层的直线距离约为20.2 km。沿着河流的走向,对该镇上的各类建筑物进行了详尽的现场调查,主要涵盖了村庄的民房、工厂的厂房、工人宿舍等80多个建筑物调查点,其不同区域建筑物的震害程度分布示于图3。可以看到,在靠近河流的阶地前缘区域建筑物破坏程度严重,而远离河流的山前阶地后缘区域建筑物震害则相对较轻。具体表现为阶地后缘的建筑物大多保持了较好的完整性或只遭受了轻微的损害,如图4所示;而阶地前缘的建筑物却受到了严重的破坏,尤其是恰好位于阶地前缘与坡地交汇处的蓥峰实业工厂,其地下是厚度约30—50 m的坡洪积漂卵石层,地震发生后该工厂的车间、厂房普遍倒塌破坏,如图5所示。
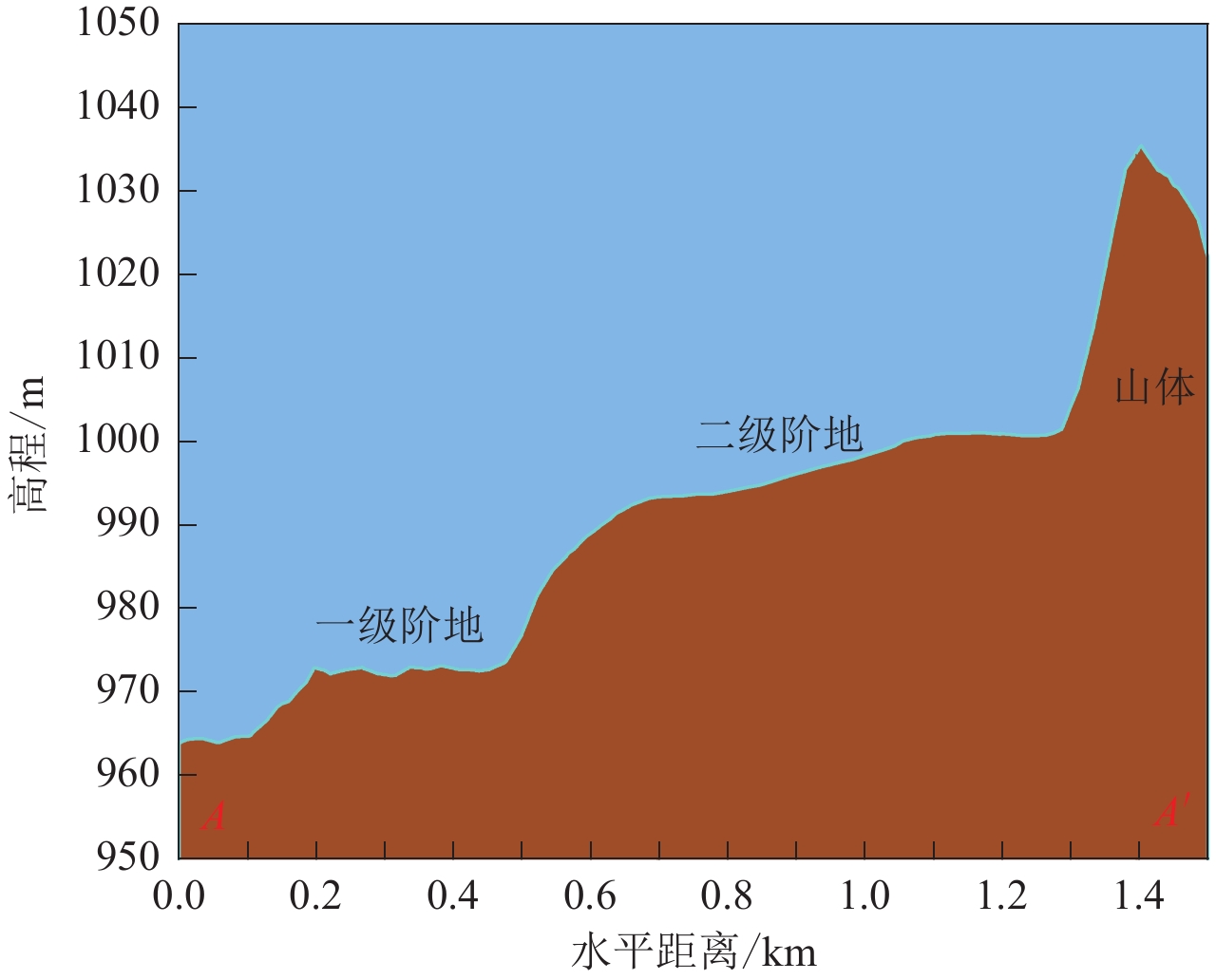
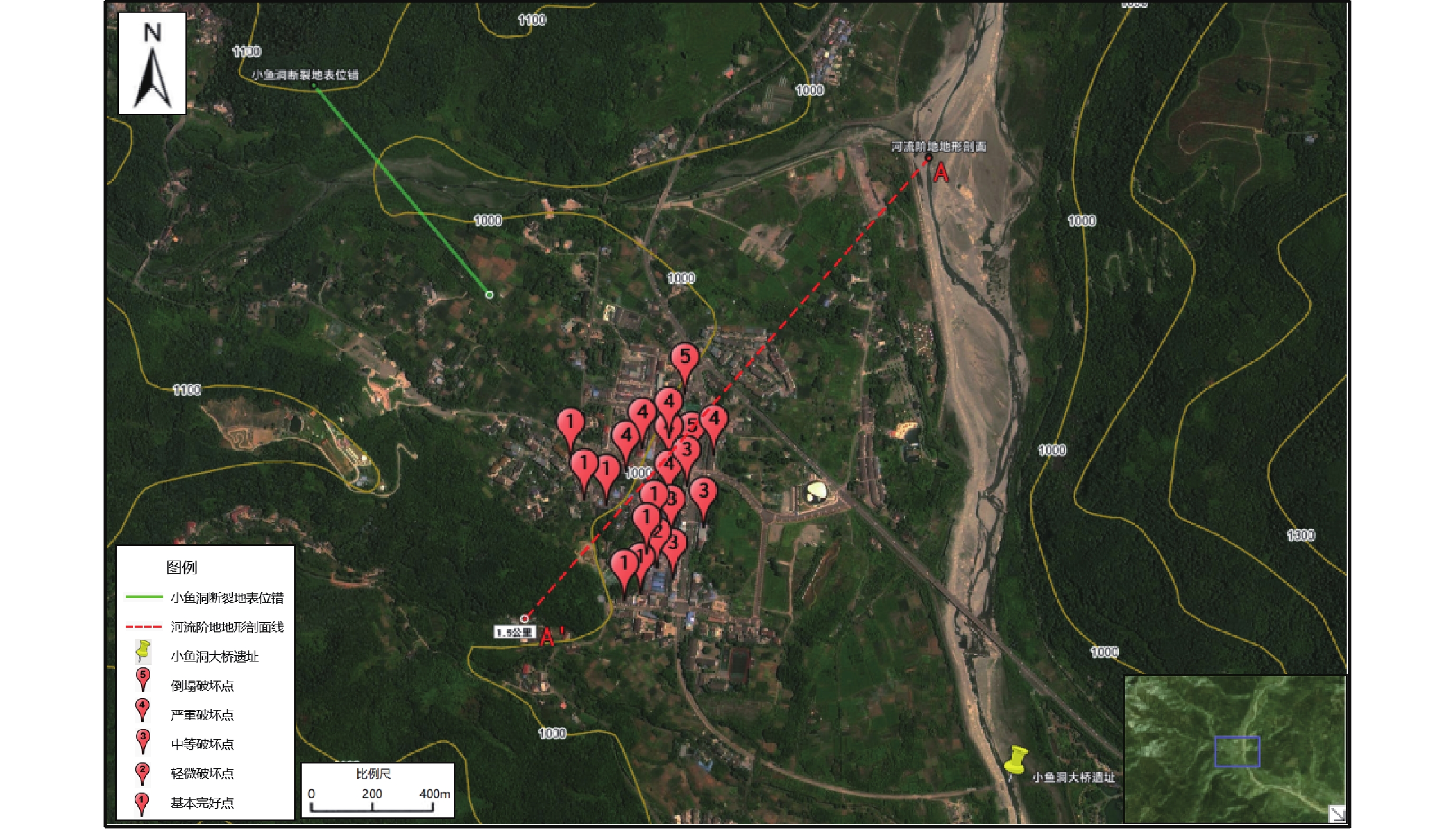
彭州小鱼洞镇坐落于湔江顺流右岸Ⅱ级阶地与山前洪积扇交汇处,位于Ⅹ度地震烈度区,与主断层直线距离为12.7 km。该镇地势自西向东逐渐降低,形成了明显的Ⅱ级河流阶地地形,如图6所示。在西南方向,山脚处的基岩局部裸露,其覆盖层以坡残积为主;而沿着东北方向靠近湔江,逐渐变为以坡洪积和冲洪积为主的覆盖层,厚度也由最初的3—5 m逐渐增加到20—30 m左右。在离湔江较近的Ⅱ级阶地前缘区域,存在厚度超过20 m的卵砾石层和漂卵石层,阶地表层则覆盖着厚度不足1 m的黏土层。小鱼洞镇建筑物震害分布示于图7,其中调查的建筑物结构基本以单层砌体结构和多层框架结构为主,部分工厂的厂房则是钢结构,而房屋的基础类型多为条形基础,埋深较浅,仅有1.0—2.0 m左右。同时结合Ⅱ级河流阶地地形剖面图,可以清晰地观察到从阶地前缘至后缘位置,建筑物的破坏程度呈现出明显的减轻趋势。在阶地前缘(水平距离约为0.8—0.9 km),房屋倒塌和严重破坏的现象频繁发生,如图8所示;而在山前较缓的阶地后缘(水平距离约为1.2 km),房屋则大多保持完好或受到轻微损害,如图9所示。值得注意的是,小鱼洞断裂的地表破裂在图7中以NW40°方向展布,向东南方向延伸至草坝。破裂性质表现为左旋逆冲,西南盘相对上升,东北盘相对下降,观测到的最大竖向位移达2.7 m,最大水平位移为1.4 m,地表破裂带的宽度为2.3—20.0 m。作者和赵纪生等2008年在地震现场科考发现,小鱼洞地表破裂穿越罗阳村复兴路西侧建筑物倒塌情况,竖向位错1.0 m,水平位错不明显,地表破裂带的宽度为21.0 m;建筑物完全倒塌宽度为26.0 m,上盘比下盘破坏较严重(赵纪生等,2009)。由此可见,小鱼洞镇阶地前缘的400.0 m左右的严重破坏和倒塌破坏分布范围远大于断裂控制的26.0 m,故断裂应该不是小鱼洞大面积震害的主要控制因素。
2. 河流阶地地震动数值模拟
2.1 建立阶地模型
基于汶川MS8.0地震现场的震害调查与野外勘查资料,选取河流一侧具有代表性的阶地地形作为研究对象,通过构建三维模型来分析其地震动响应特性。此过程中,首先利用软件的插值拟合技术生成连续平滑的地层界面;随后通过精细的离散化处理将地层界面转化为FLAC3D软件能高效读取的表格数据;最后利用FLAC3D中的topography命令,对初始网格进行拉伸与变形,构建出具有复杂地形特征的几何模型。
为确保能够全面反映阶地地形的特性,对模型尺寸进行了设计。在水平方向上,x轴长度设定为70 m,充分覆盖了阶地地形的主要横截面特征,y轴则延伸至200 m。竖直方向上,以计算基底为准,设定了各级阶地及河流底部的高程,Ⅲ级阶地52 m、Ⅱ级阶地32 m、Ⅰ级阶地17 m以及河流底部7 m。此外,考虑到河流阶地的地质演化过程,模型建立时体现了阶地上覆土层的厚度变化,即由阶地后缘向前缘覆盖土层厚度逐渐增加,如图10所示。
在边界条件设置方面,为真实地模拟地震动在无限域或半无限域中的传播环境,模型的四周采用了自由边界条件。同时,鉴于模型底部为模量较大的基岩条件,故直接在其底部施加加速度时程,以确保地震动的有效输入。选取了瑞利阻尼作为模型的阻尼类型,设定临界阻尼比0.05,中心频率6.0 Hz。依据岩土体的物理力学参数经验值,对模型中不同岩土体设定了合理的参数见表1,以确保数值分析的可靠性。
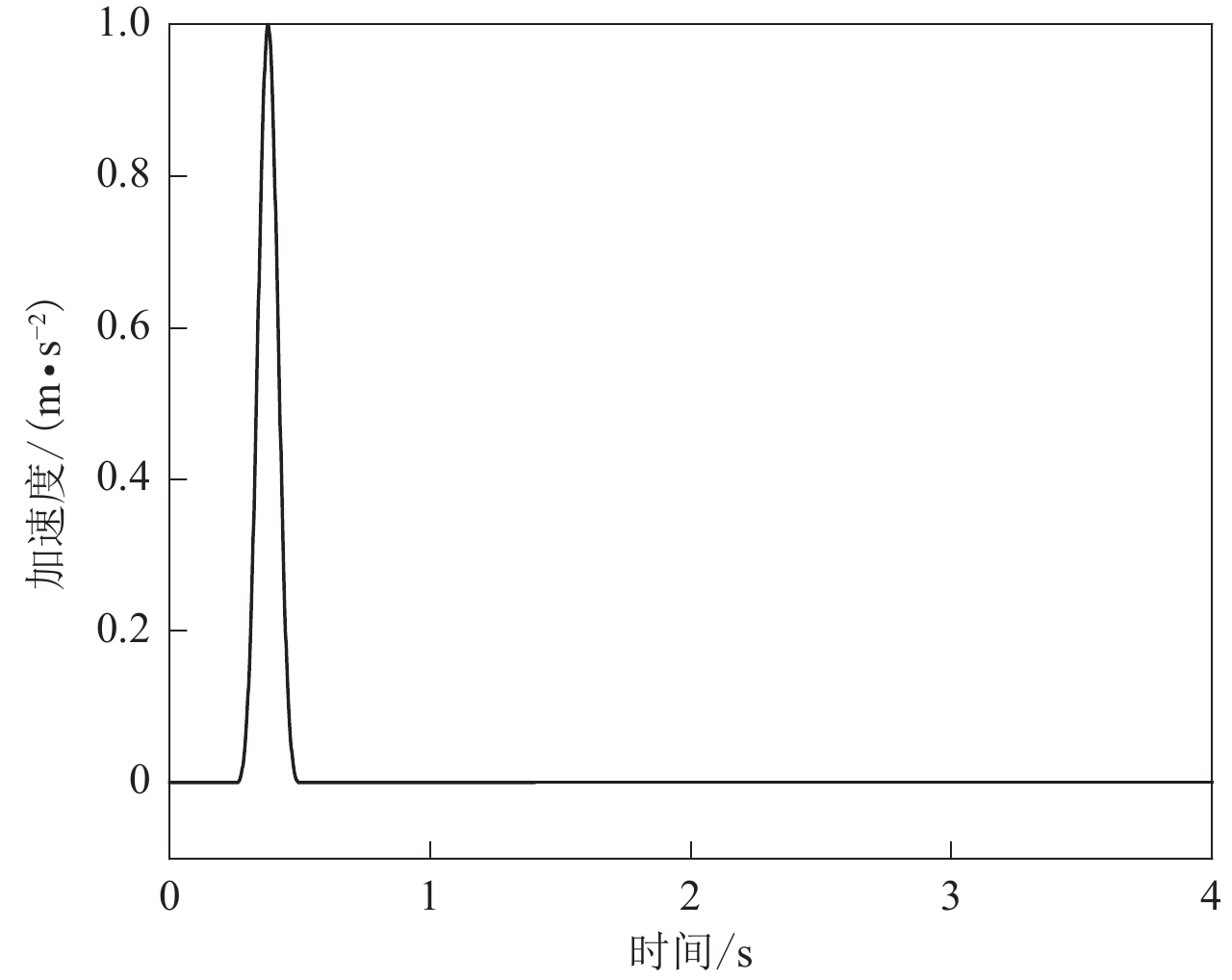
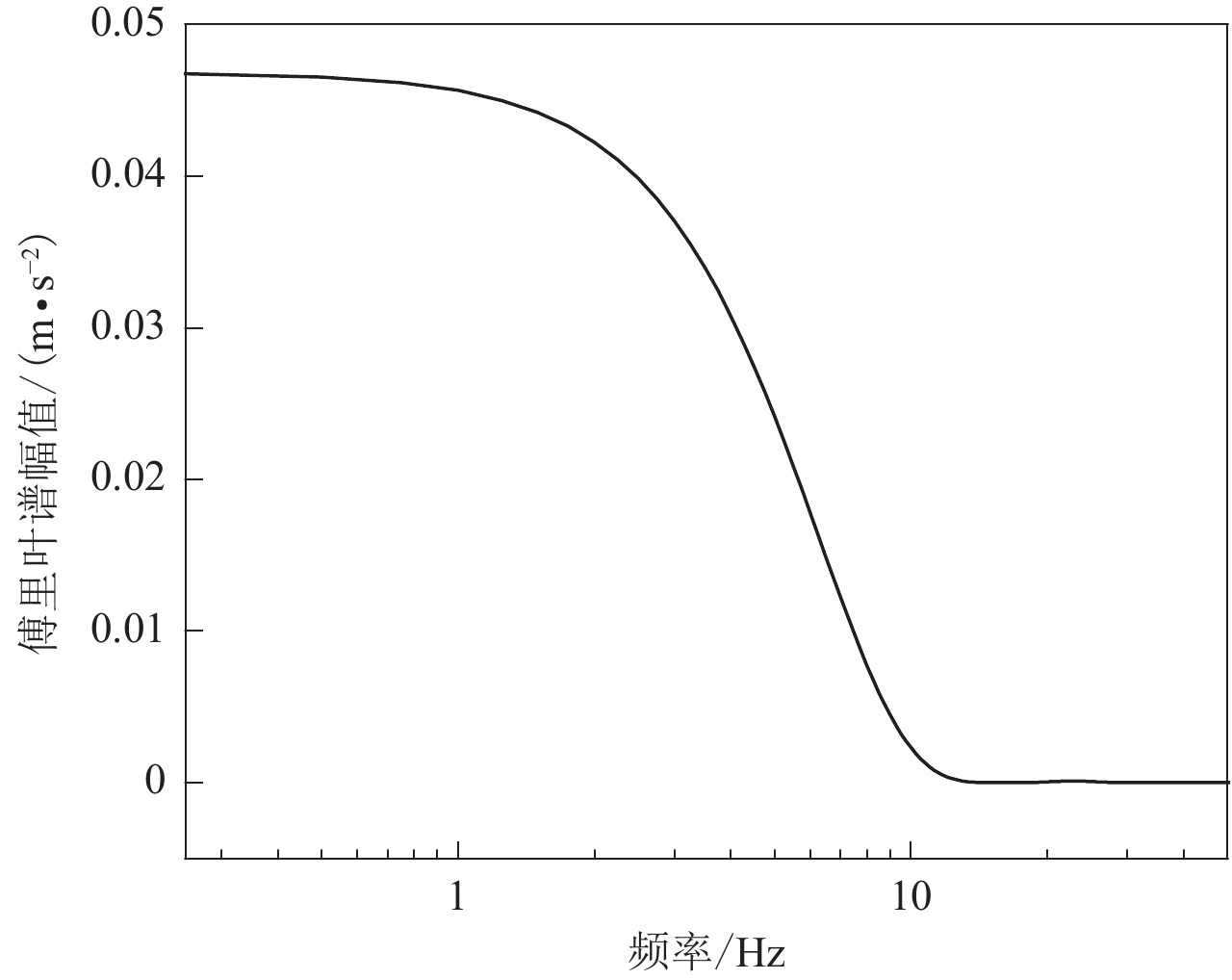
表 1 计算模型岩土体物理力学参数Table 1. Physical and mechanical parameters of rock and soil mass for the calculation model岩土类型 密度(kg/m3) 体积模量(GPa) 剪切模量(GPa) 粘聚力(MPa) 内摩擦角(°) 粉质黏土 1850 0.160 0.074 0.023 25 砂岩 2487 5.970 6.010 2.060 40 在计算过程中,沿模型底部x、y、z三个方向同时输入脉冲函数形式的动荷载,其宽度为0.25 s,时间步距为0.002 s,如图11所示,相应的傅里叶谱特征示于图12,可知动荷载的频率成分截止到10.0 Hz。在模型地表布置了42个加速度时程监测点,具体位置见图10所示。
2.2 数值模拟结果分析
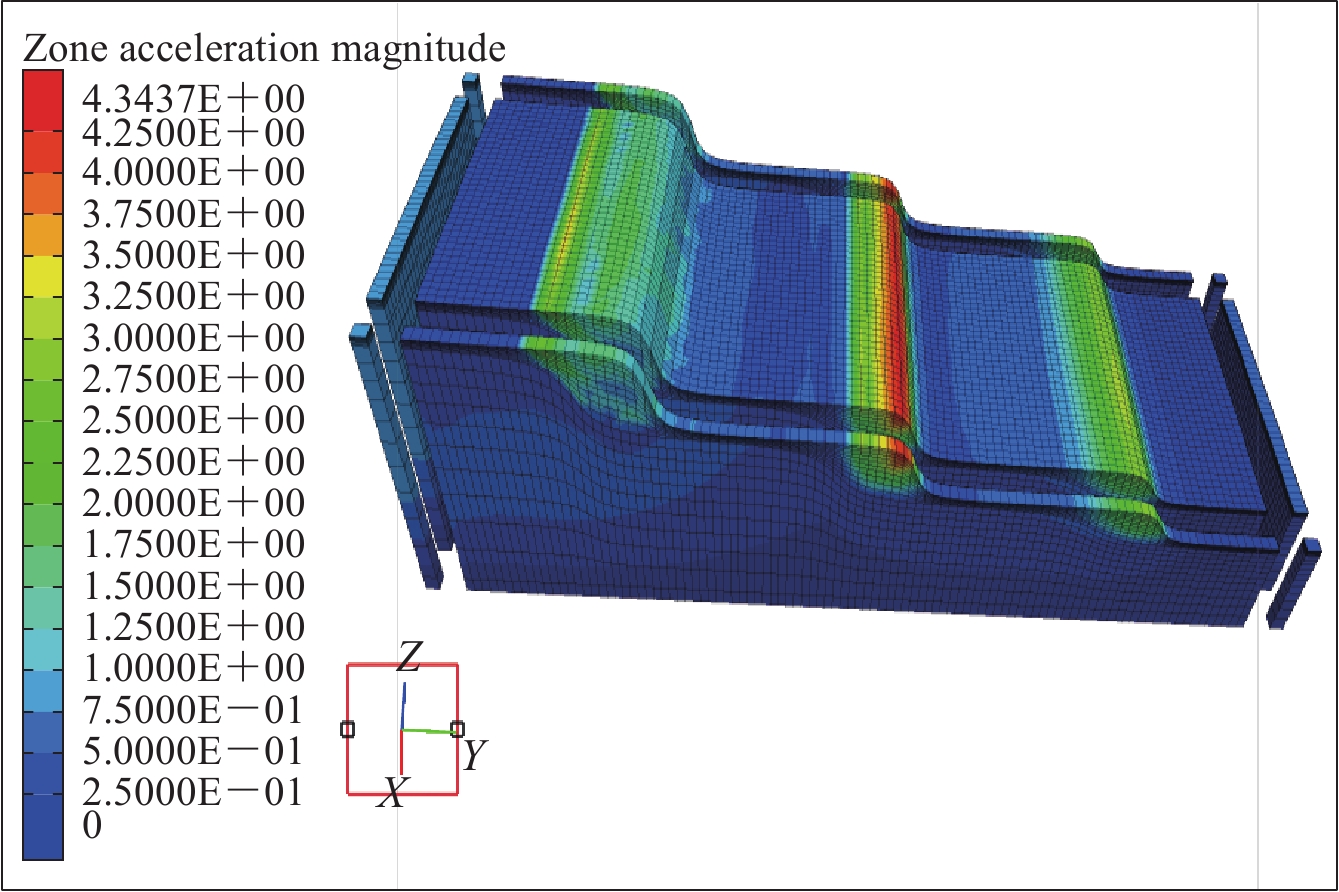
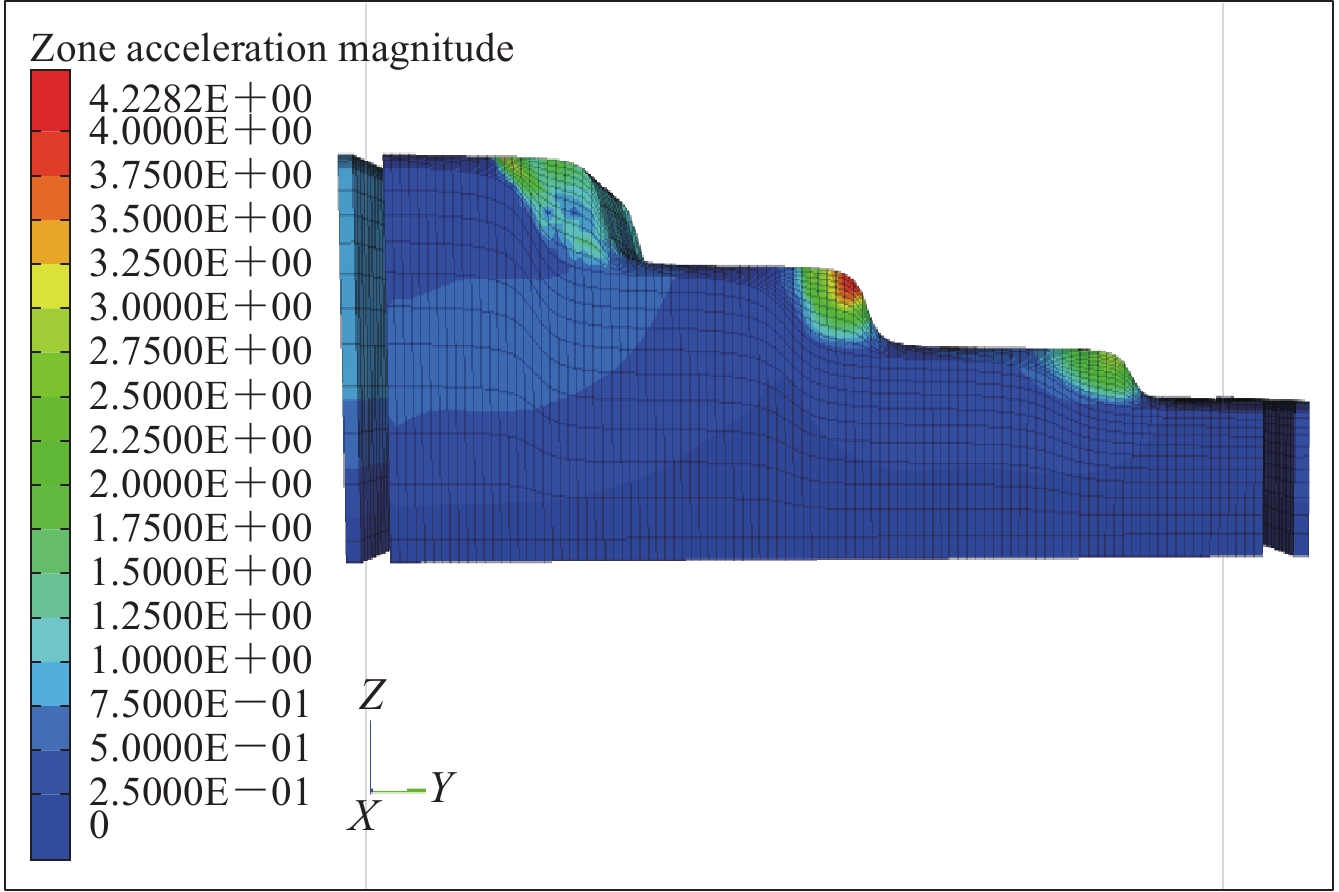
通过对阶地模型底部x、y、z三个方向同时施加脉冲荷载,模拟其在三向地震作用下的动力响应,获得了阶地模型加速度等值线云图与地表监测点剖面加速度分布云图,分别示于图13和图14。图中清晰地揭示了阶地地形对地震动水平空间差异分布的影响,各级阶地加速度较高的区域主要集中在阶地前缘,而后缘的加速度水平则相对较低。
2.2.1 加速度峰值分析
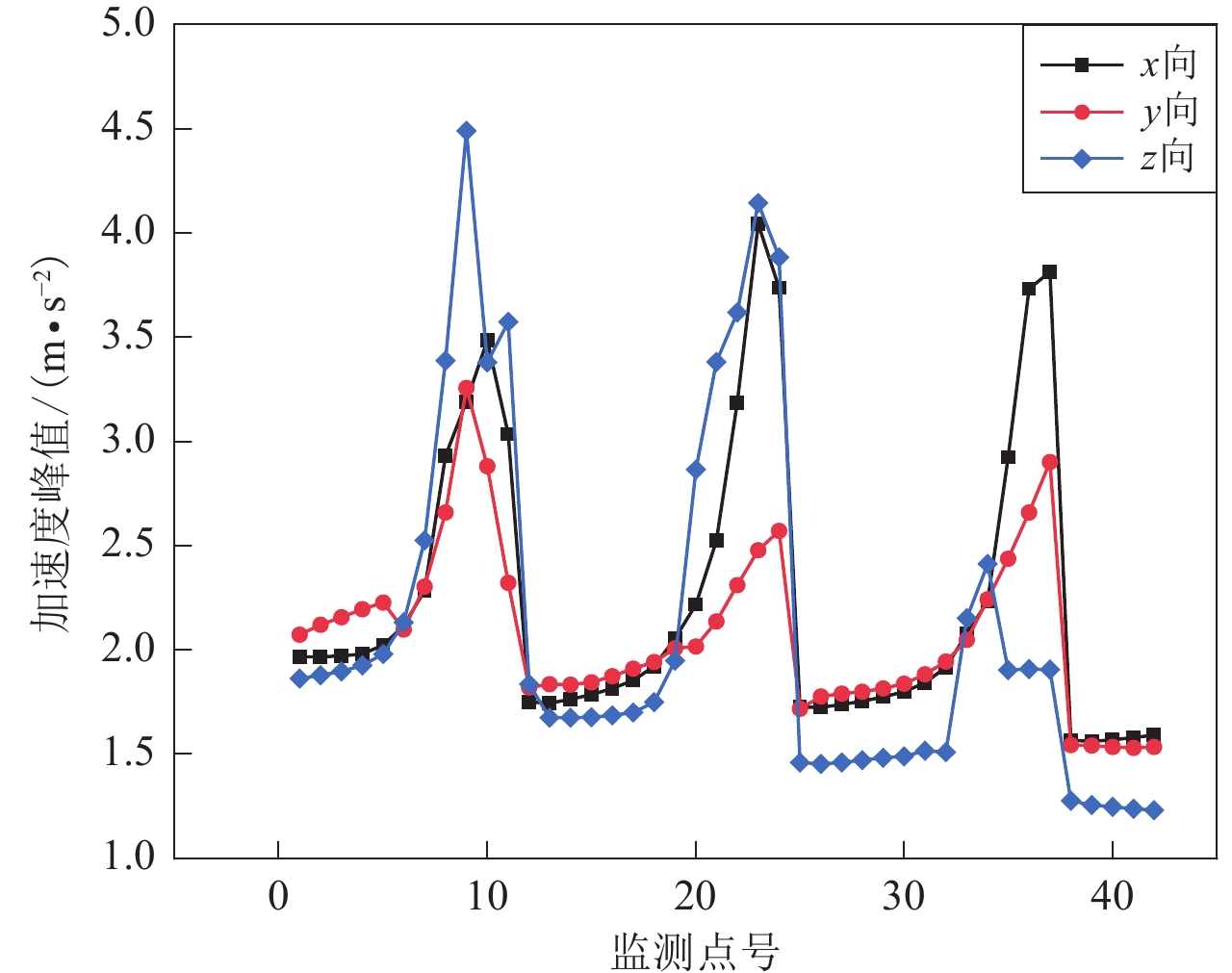
为进一步量化该分布特征,绘制了各级阶地地表监测点x、y、z三个方向上的加速度峰值变化趋势,如图15所示。可以看出,三个方向上的加速度峰值变化趋势基本一致,均随阶地地形的起伏和上覆土层厚度的变化而变化。具体而言,加速度峰值在各级阶地的前缘区域都出现了极大值,随阶地级数的减小,阶地对应区域的地表加速度峰值也逐渐下降。以竖直z向为例,Ⅲ级阶地后缘测点#1—5的加速度峰值主要在1.90 m/s2左右,Ⅱ级阶后缘测点#13—18的加速度峰值则在1.68 m/s2左右,而Ⅰ级阶后缘测点#26—31的加速度峰值却处于更低的1.50 m/s2附近。此外对同一级别阶地,随着上覆土层厚度的增加,各方向上的加速度峰值呈现上升趋势。特别是在阶地前缘与陡坎转折处,由于地形的突变和波速的变化,加速度显著偏大。在Ⅲ级阶地上,随上覆土层厚度的增加(测点#6—10),三个方向的加速度峰值均明显增大;而位于阶地前缘与陡坎转折处的测点#11,其x、y和z方向加速度峰值分别则为3.03 m/s2、2.32 m/s2和3.57 m/s2,显著高于其他位置。
2.2.2 加速度持时分析
由于结构地震反应与破坏程度与输入地震动的能量有关,故以给定比例的能量为准则确定地震动的持续时间。按照公式1计算能量函数En(t)随时间的变化:
$$ {E_n} ( t ) =\int_0^t {{a^2} ( t ) } dt/\int_0^{{T_0}} {{a^2} ( t ) dt} $$ (1) 式中,a(t)为加速度时程,T0为总持续时间。En(t)的物理意义则可视为单位质量的单自由度体系在地震动a(t)作用下,t时刻的能量与总能量之比。选取比值的上限和下限分别为95%和5%,则达到上限时刻t2和达到下限时刻t1之间的时间间隔为强地震动的能量持时,它表示此时段内输入结构的能量占总能量的90%,也称90%能量持时。
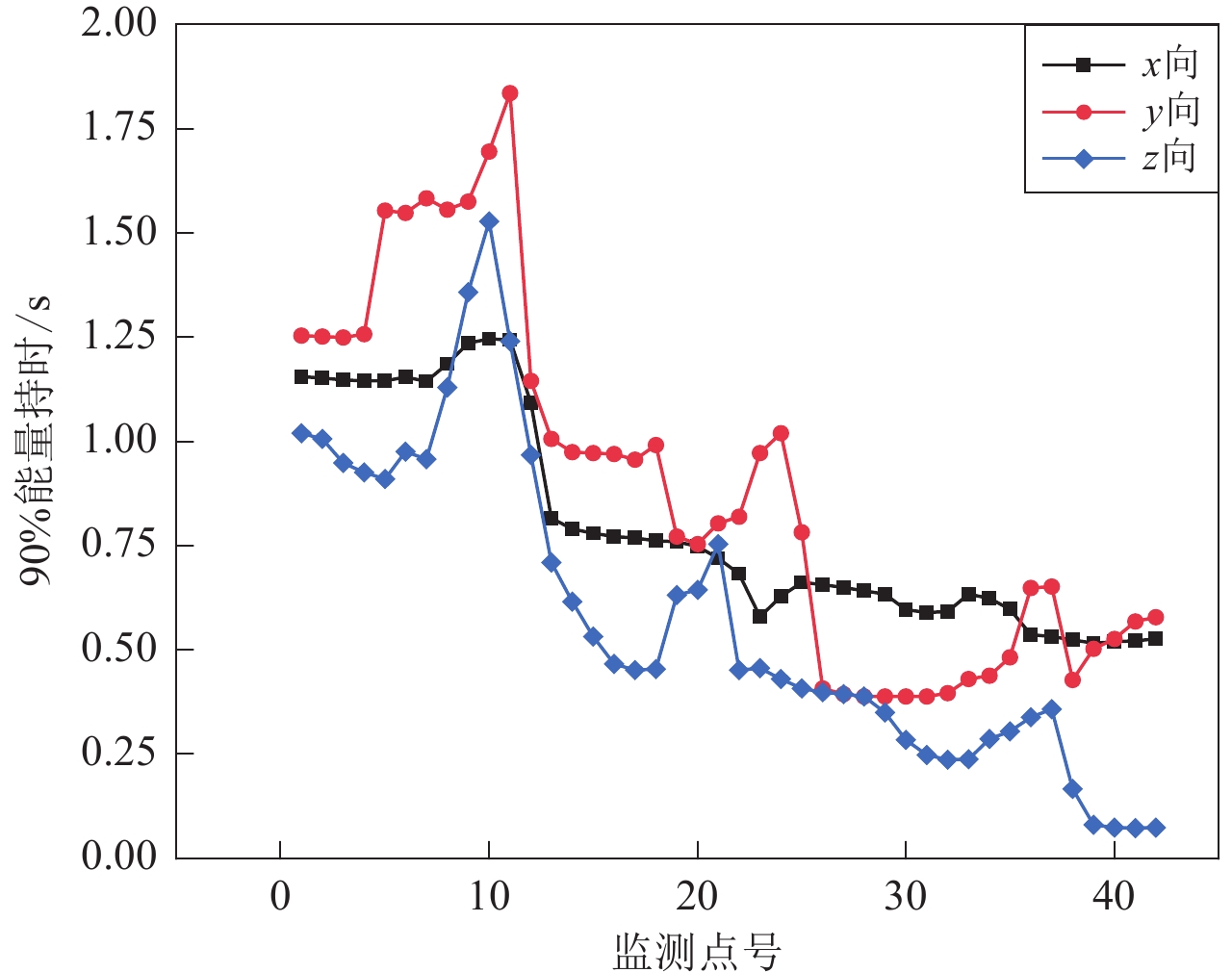
各级阶地地表监测点加速度的90%能量持时变化示于图16。可以看出,三个方向的相对持时变化趋势大体相似,均随阶地级数的减小而波动下降;而对同一级别阶地,前缘的监测点持时水平还是明显大于后缘,并且前缘区域受到上覆土层厚度和阶地几何形状的耦合影响,出现了持时极大值。在Ⅲ级阶地上,随上覆土层厚度的增加,测点#6—10在三个方向上的相对持时也呈现上升趋势;并在阶地前缘与陡坎转折处的测点#11达到最大值,其x、y和z方向上的持时值分别为1.24 s、1.83 s和1.24 s,这与前面加速度峰值的变化规律相一致。
综合对比各级阶地地表监测点的加速度峰值和相对持时,可以发现两者的变化趋势有一定相似性。第一,随着阶地级数的减小,地表加速度峰值和相对持时值均下降。这说明阶地级数越高,地震动在地形中的放大效应越显著。第二,对同一级别阶地上覆土层厚度变化明显的区域,加速度峰值和相对持时值都显著变化。随着上覆土层厚度的增加,加速度峰值和持时均呈现上升趋势。第三,在阶地前缘与陡坎转折处,加速度峰值和相对持时值均达到了最大。这表明地形几何形状的突变和厚覆盖土层对地震动的放大有耦合效应。第四,在同一级别阶地上,前缘区域的监测点加速度和持时水平明显高于后缘,也进一步证明了阶地地形对地震动响应的空间分布差异具有显著影响。
2.2.3 加速度频谱分析
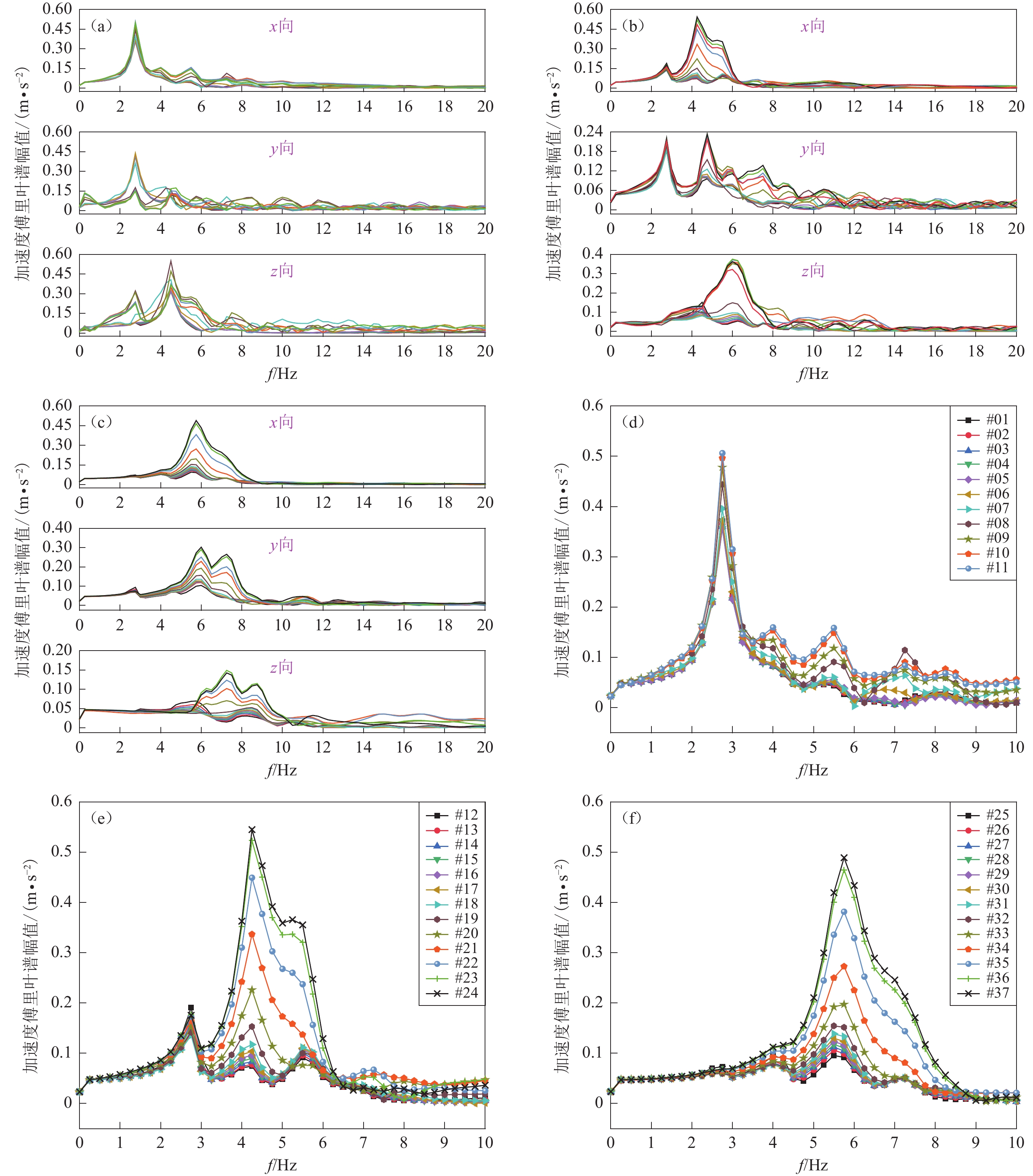
为深入分析各级阶地地表不同监测点的地震动频谱特性,对监测点的加速度时程进行傅里叶变换,得到傅里叶谱。随后,将各测点的傅里叶谱与计算基底输入的脉冲荷载傅里叶谱进行谱比分析,以确定阶地不同位置对脉冲荷载各种频率成分的放大效应。此外,还对监测点的加速度反应谱进行规准,探讨了其特征周期、平台值以及放大系数的变化规律。
首先,从图17加速度傅里叶谱可以看出,各级阶地之间的幅值变化存在显著差异。这主要归结于阶地地形几何构造特征、土层厚度以及相对高度的差异影响。同一级别阶地,尽管各方向上的幅值谱有所不同,但在相同方向上的幅值谱形状却基本保持一致。对于Ⅲ级阶地在水平x和y向上,幅值谱的峰值均集中在2.8 Hz左右的频率处,表明在水平方向上的地震动能量主要集中在该频率附近。竖直z向的谱峰值则出现在4.5 Hz左右,说明竖向上地震动能量集中分布的频段高于水平向。在Ⅱ级阶地的水平x向和竖直z向上,最大谱值分别集中于4.2 Hz和6 Hz附近,表明随阶地级数的减小(即地形高度的降低),地震动能量的集中频率段有所升高。在水平y向上,幅值谱在2.8 Hz和4.6 Hz两个频率处均出现较大的值,这可能与该方向上的地形复杂性或覆盖层不均匀性有关。而在Ⅰ级阶地的水平x向上,幅值谱峰值出现在5.8 Hz左右,水平y向出现在6 Hz和7.2 Hz的频率处,进一步验证了随阶地级数减小,地震动能量集中频率上升的趋势。竖直z向的较大谱值则出现在7.2 Hz和8.8 Hz的频率附近。这说明在更低阶的阶地上,地震动频谱特性更为复杂,多频成分共存。
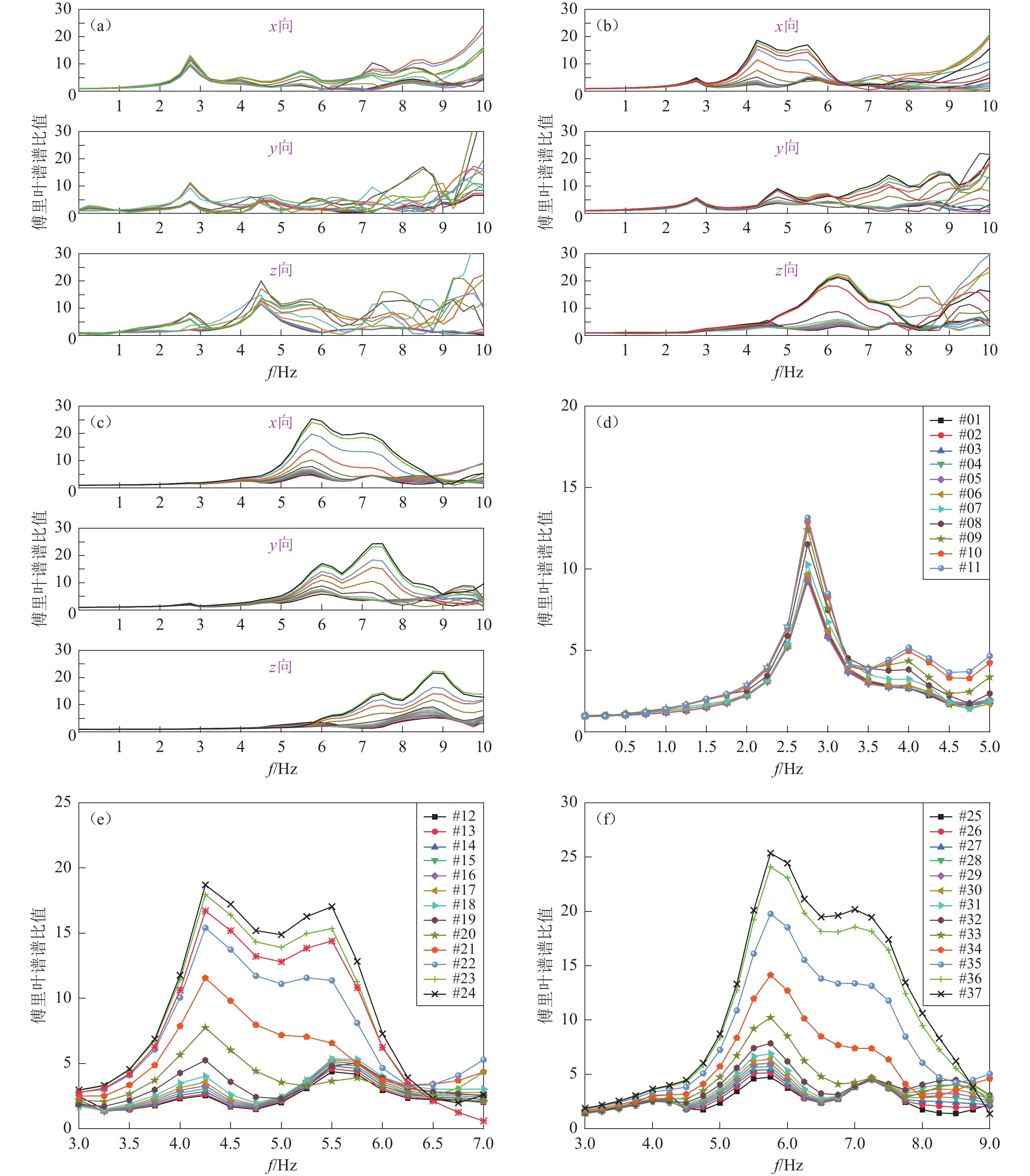
各级阶地不同测点的傅里叶谱比示于图18,可以看出不同级别阶地对地震动频率成分的放大效应存在显著差异。比如水平x方向上,Ⅲ级阶地在2.7 Hz左右频率处出现较大的谱比值,而Ⅱ级和Ⅰ级阶地则分别在更高频段4.0—6.0 Hz和5.0—8.0 Hz范围内达到谱比峰值。随着阶地级数的增加,其对地震动低频成分的放大效应也显著的增强。这主要是因为阶地级数的增加(即地形高度上升),其自振周期也相应增大,从而在低频段内产生共振效应。由图18中的d、e和f可知,同一级别阶地上不同监测点的谱比值变化趋势虽然一致,但谱比值的大小差异显著。阶地后缘区域的测点谱比曲线较为一致,随着测点向阶地前缘区域靠近,谱比值逐渐增加,其原因一方面是由于测点位置逐渐靠近阶地陡坎临空面,该处测点振动所受周边岩土体的介质约束较小,另一方面则是上覆土层厚度的增加,对地震动有显著放大作用,因此阶地的几何形状与上覆土层对地震动的放大有耦合效应。
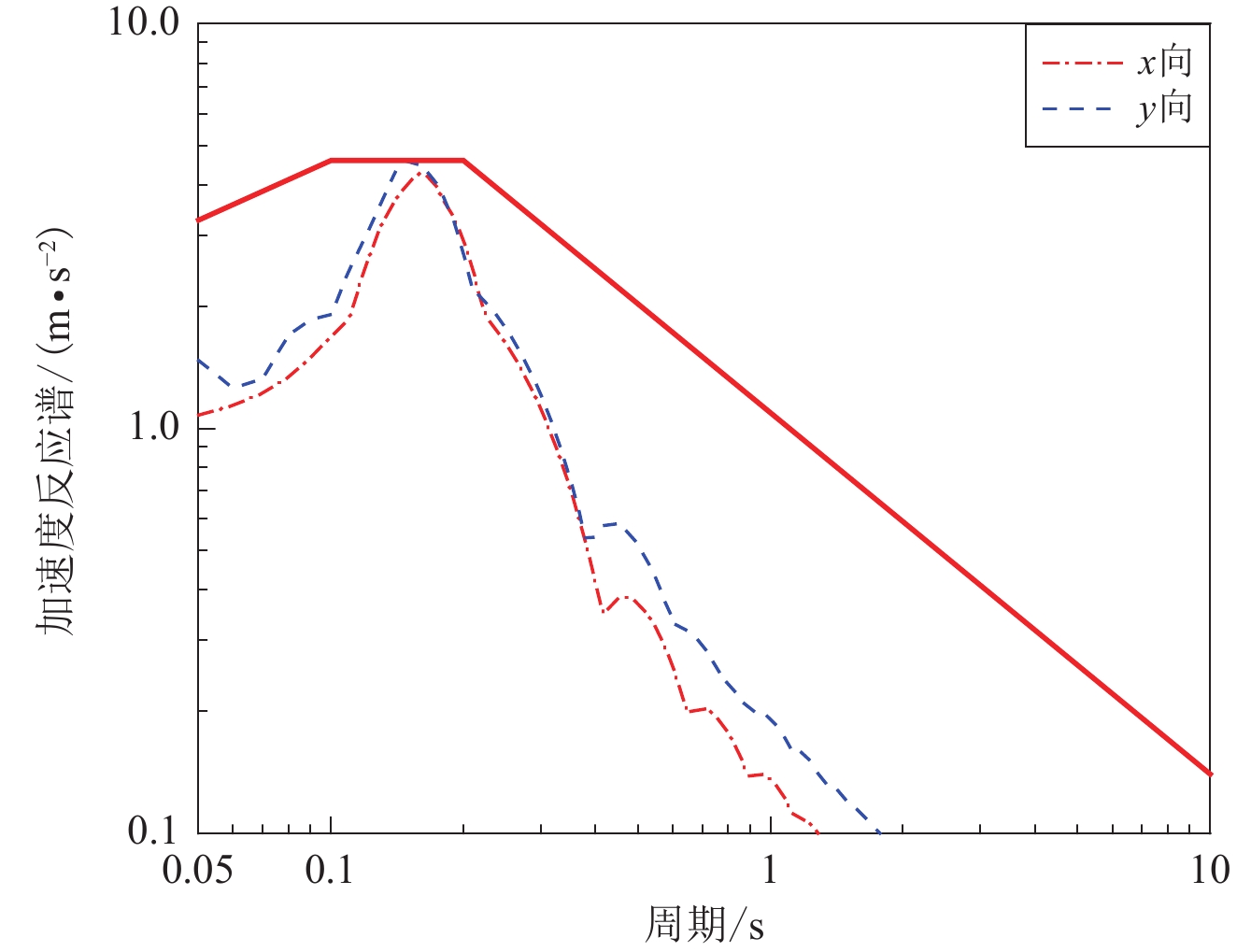
对阶地模型地表监测点水平x和y向的加速度反应谱进行规准化处理,以得到地震动加速度规准反应谱。其中归准化参数包括地震动的峰值加速度amax、反应谱平台值放大系数βm、第一拐点周期T0(通常取0.1 s)、第二拐点周期(特征周期)Tg、周期值范围上限Tm以及下降段下降速度控制参数α(如衰减指数取0.9)。具体计算时,使用公式2和公式3进行,同时列举了测点#36的规准反应谱曲线,如图19所示。
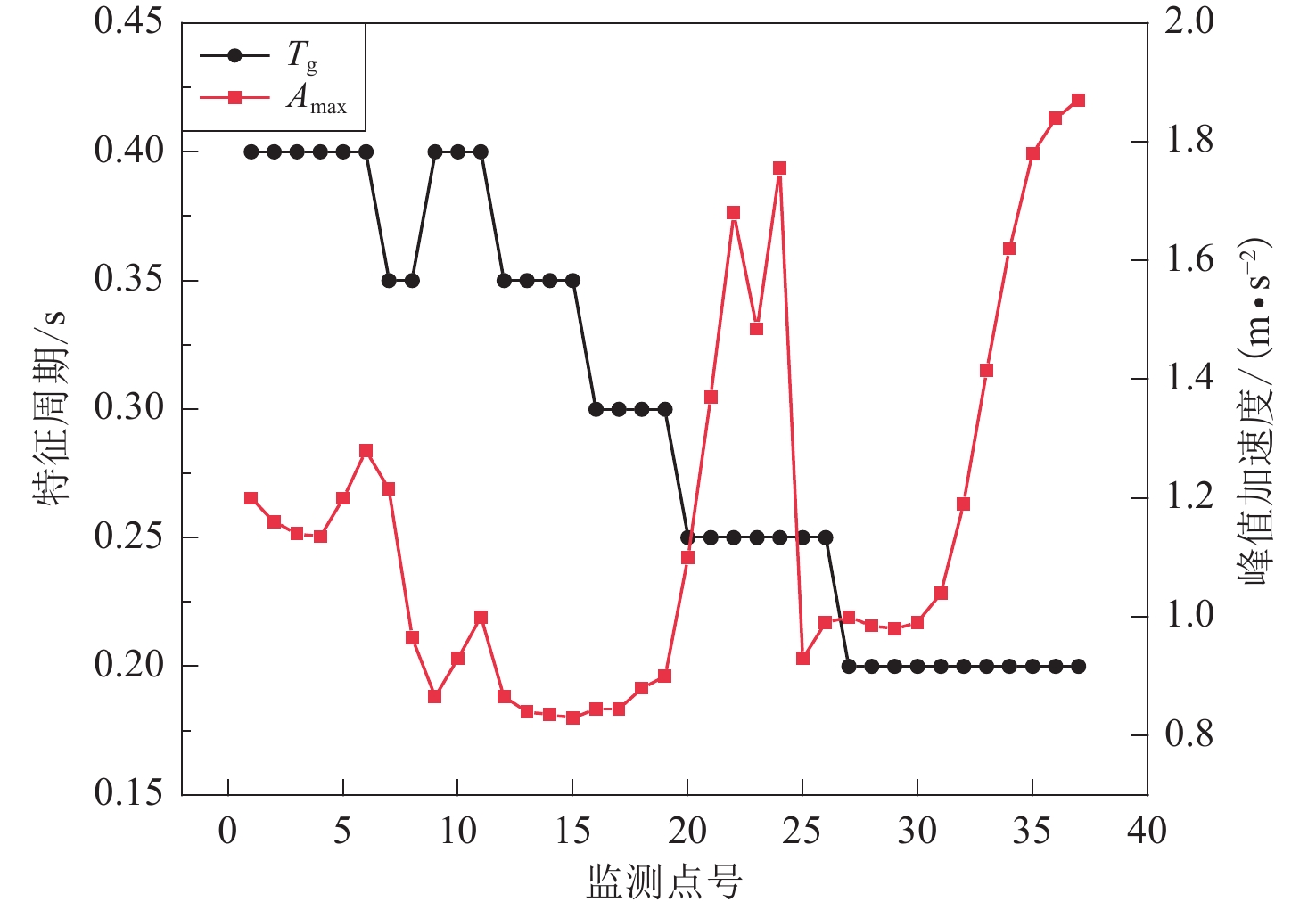
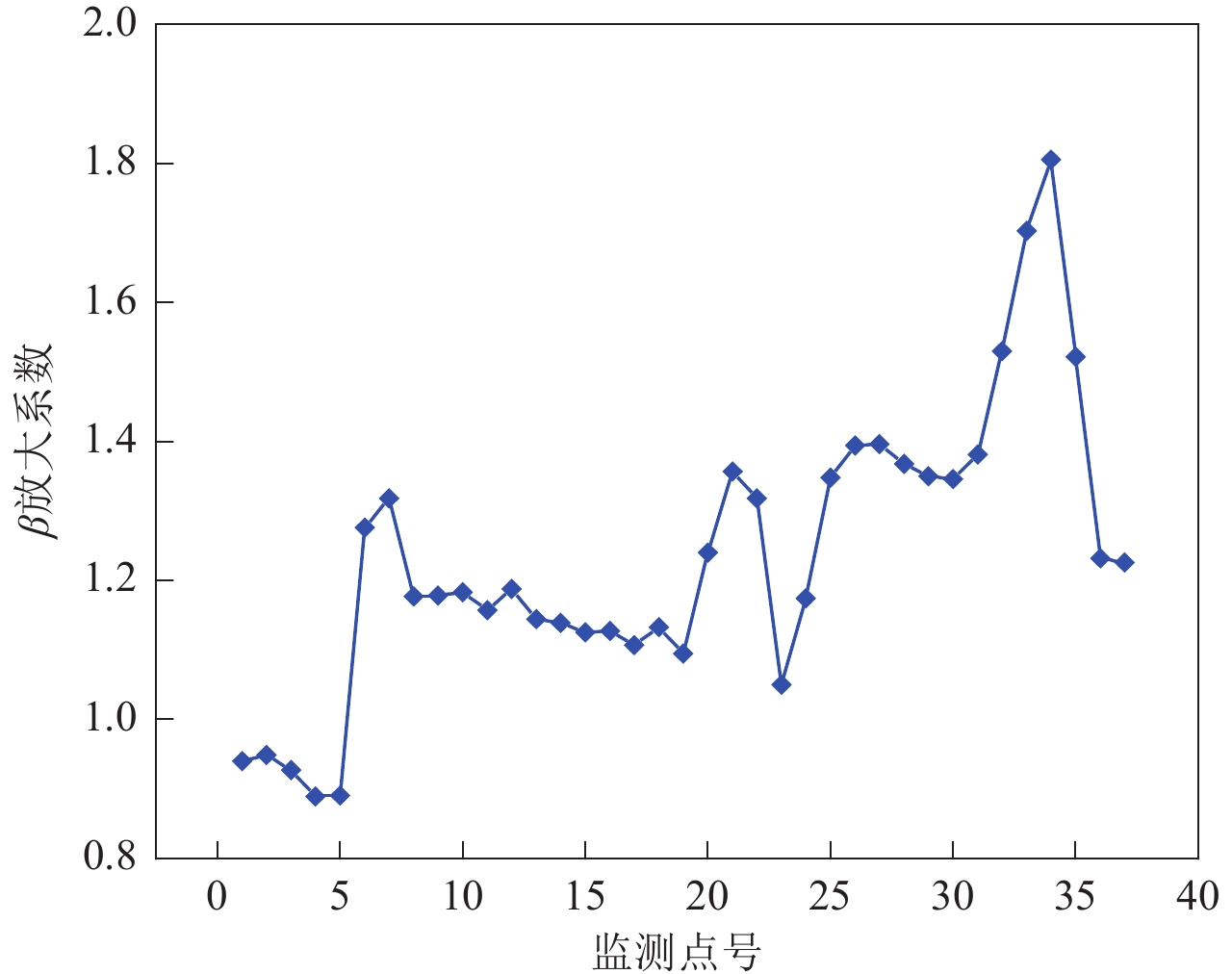
$$ {S_a} ( T ) ={a_{\max }}\beta ( T ) $$ (2) $$ \beta ( T ) =\left\{ \begin{gathered} 1 + ( {{\beta _m} - 1.0} ) \frac{T}{{{T_0}}}\text{,} 0.0 {\text{<}} T {\text{≤}} {T_0} \\ {a_{\max }}{\beta _m}\text{,} {T_0} {\text{<}} T {\text{≤}} {T_g} \\ {\beta _m}{\left( {\frac{{{T_g}}}{T}} \right)^\alpha }\text{,} {T_g} {\text{<}} T {\text{≤}} {T_m} \\ \end{gathered} \right. $$ (3) 阶地模型地表监测点水平向规准反应谱具体参数见表2,根据表中参数绘制各级阶地不同监测点反应谱的特征周期、平台值以及平台放大系数的变化趋势分别示于图20和图21。其中特征周期Tg值受阶地级数影响显著,随级数的减小,特征周期也逐渐减小。Ⅲ级阶地的Tg值基本在0.4 s左右,Ⅱ级阶地则在0.25—0.35 s范围内,而Ⅰ级阶地却处于更低的0.2 s左右。说明高级阶地(地势较高)对地震动中的低频成分能量有显著的放大效应,这与阶地的几何尺寸和入射地震波波长有关。反应谱的平台值Sa则主要受到上覆土层厚度与地形几何形状的影响。同一级别阶地上前缘区域的平台值显著高于后缘,这与阶地前缘的几何形状有关,即前缘部分临空面对地震动没有约束作用;同时随着前缘区域上覆土层厚度的增加,对地震动的放大效应增强,反应谱平台值也逐渐增大。此外,平台放大系数β值同样受阶地级数与几何条件的影响,随阶地级数的增加,放大系数却呈下降趋势,这是因为高级阶地对高频地震动有滤波作用,导致高频成分减少,而β放大系数的大小却与地震动输入中的高频成分直接相关。在同一级别阶地上,前缘区域的放大系数明显大于后缘,这也进一步说明阶地几何形状对地震动有显著的影响。
表 2 阶地模型地表监测点规准反应谱参数Table 2. Standard response spectrum parameters of surface monitoring points in the terrace model监测点号 T0(s) Tg(s) Sa(m/s2) β 监测点号 T0(s) Tg(s) Sa(m/s2) β 1 0.10 0.40 1.963 0.940 20 0.10 0.25 2.750 1.240 2 0.10 0.40 2.013 0.949 21 0.10 0.25 3.425 1.357 3 0.10 0.40 2.050 0.927 22 0.10 0.25 4.200 1.318 4 0.10 0.40 2.125 0.889 23 0.10 0.25 4.250 1.050 5 0.10 0.40 2.288 0.890 24 0.10 0.25 4.388 1.174 6 0.10 0.40 2.700 1.276 25 0.10 0.25 2.325 1.348 7 0.10 0.40 3.038 1.318 26 0.10 0.25 2.475 1.394 8 0.10 0.40 3.450 1.177 27 0.10 0.20 2.500 1.396 9 0.10 0.40 3.838 1.178 28 0.10 0.20 2.463 1.368 10 0.10 0.40 4.125 1.183 29 0.10 0.20 2.450 1.350 11 0.10 0.40 3.513 1.157 30 0.10 0.20 2.475 1.346 12 0.10 0.35 2.163 1.188 31 0.10 0.20 2.600 1.381 13 0.10 0.35 2.100 1.144 32 0.10 0.20 2.975 1.530 14 0.10 0.35 2.088 1.139 33 0.10 0.20 3.538 1.703 15 0.10 0.35 2.075 1.125 34 0.10 0.20 4.050 1.806 16 0.10 0.30 2.113 1.127 35 0.10 0.20 4.450 1.522 17 0.10 0.30 2.113 1.107 36 0.10 0.20 4.600 1.232 18 0.10 0.30 2.200 1.133 37 0.10 0.20 4.675 1.226 19 0.10 0.30 2.250 1.095 3. 讨论与结论
本文通过对汶川地震中典型河流阶地的现场震害调查,结合阶地地形的三维数值模拟结果,深入探讨了河流阶地地形对建筑物震害特征及其产生机理的影响。
现场调查发现阶地前缘建筑物震害程度显著高于后缘,这是由于前缘的厚冲洪积层放大地震动而导致震害严重,后缘则因薄坡积层对地震动的衰减使震害较轻。数值结果也显示,各级阶地前缘的地震动水平普遍高于后缘,且随上覆土层增厚,地表加速度峰值、相对持时、反应谱平台值以及放大系数均呈上升趋势。研究还发现,阶地级数对地震动的空间分布和总体水平也有显著影响。随阶地级数的减小,加速度峰值、相对持时和反应谱特征周期都呈现下降趋势;而阶地级数的增加则会增强对地震动低频成分的放大效应。阶地前缘与陡坎衔接处因地形几何条件与厚上覆土层对地震动放大的耦合作用,震害尤其严重。且该处数值结果中的加速度峰值、相对持时和反应谱平台值均达到极大值,同样揭示了其震害严重的原因。
综上分析,本研究得出以下结论:(1)河流阶地对地震动的传播和建筑物震害程度有显著影响,其中阶地上覆土层厚度变化是导致震害程度分布不同的主要原因之一。(2)阶地级数通过影响地震动的频谱特性和总体水平来加剧或减轻震害。其中低级别阶地对地震动具有较强的衰减作用,而高级别阶地则会放大地震动的低频成分。(3)特殊地形条件如阶地前缘与陡坎转折位置在地震中可能遭受更为严重的破坏,这为重大工程的抗震设计提供参考。
-
表 1 计算模型岩土体物理力学参数
Table 1 Physical and mechanical parameters of rock and soil mass for the calculation model
岩土类型 密度(kg/m3) 体积模量(GPa) 剪切模量(GPa) 粘聚力(MPa) 内摩擦角(°) 粉质黏土 1850 0.160 0.074 0.023 25 砂岩 2487 5.970 6.010 2.060 40 表 2 阶地模型地表监测点规准反应谱参数
Table 2 Standard response spectrum parameters of surface monitoring points in the terrace model
监测点号 T0(s) Tg(s) Sa(m/s2) β 监测点号 T0(s) Tg(s) Sa(m/s2) β 1 0.10 0.40 1.963 0.940 20 0.10 0.25 2.750 1.240 2 0.10 0.40 2.013 0.949 21 0.10 0.25 3.425 1.357 3 0.10 0.40 2.050 0.927 22 0.10 0.25 4.200 1.318 4 0.10 0.40 2.125 0.889 23 0.10 0.25 4.250 1.050 5 0.10 0.40 2.288 0.890 24 0.10 0.25 4.388 1.174 6 0.10 0.40 2.700 1.276 25 0.10 0.25 2.325 1.348 7 0.10 0.40 3.038 1.318 26 0.10 0.25 2.475 1.394 8 0.10 0.40 3.450 1.177 27 0.10 0.20 2.500 1.396 9 0.10 0.40 3.838 1.178 28 0.10 0.20 2.463 1.368 10 0.10 0.40 4.125 1.183 29 0.10 0.20 2.450 1.350 11 0.10 0.40 3.513 1.157 30 0.10 0.20 2.475 1.346 12 0.10 0.35 2.163 1.188 31 0.10 0.20 2.600 1.381 13 0.10 0.35 2.100 1.144 32 0.10 0.20 2.975 1.530 14 0.10 0.35 2.088 1.139 33 0.10 0.20 3.538 1.703 15 0.10 0.35 2.075 1.125 34 0.10 0.20 4.050 1.806 16 0.10 0.30 2.113 1.127 35 0.10 0.20 4.450 1.522 17 0.10 0.30 2.113 1.107 36 0.10 0.20 4.600 1.232 18 0.10 0.30 2.200 1.133 37 0.10 0.20 4.675 1.226 19 0.10 0.30 2.250 1.095 -
董俊,赵成刚. 2005. 三维半球形凹陷饱和土场地对平面P波散射问题的解析解[J]. 地球物理学报,48(3):680–688. doi: 10.3321/j.issn:0001-5733.2005.03.028 Dong J,Zhao C G. 2005. An analytic solution for the diffraction of plane P-wave by three-dimensional hemispherical canyons in a fluid-saturated porous media half space[J]. Chinese Journal of Geophysics,48(3):680–688. (in Chinese).
高玉峰,代登辉,张宁. 2021. 河谷地形地震放大效应研究进展与展望[J]. 防灾减灾工程学报,41(4):734–752. Gao Y F,Dai D H,Zhang N. 2021. Progress and prospect of topographic amplification effects of seismic wave in canyon sites[J]. Journal of Disaster Prevention and Mitigation Engineering,41(4):734–752. (in Chinese).
高玉峰,代登辉,张宁. 2022. 翡翠河谷地震动地形效应解析分析[J]. 地震学报,44(1):40–49. Gao Y F,Dai D H,Zhang N. 2022. Analytical study on the topographic effect on ground motion of Feitsui[J]. Acta Seismologica Sinica,41(4):734–752. (in Chinese).
韩铮. 2006. 半球形凹陷谷场地对Rayleigh波的三维散射[J]. 山西建筑,32(3):52–53. doi: 10.3969/j.issn.1009-6825.2006.03.032 Han Z. 2006. Three-dimensional scattering of Rayleigh waves in hemispherical canyon[J]. Shanxi Architecture,32(3):52–53. (in Chinese).
何颖,于琴,刘中宪. 2019. 考虑散射效应沉积河谷空间相关多点地震动模拟[J]. 岩土力学,40(7):2739–2747. He Y,Yu Q,Liu Z X. 2019. Simulation of multi-point spatially correlated earthquake ground motions of sedimentary valleys considering scattering effect[J]. Rock and Soil Mechanics,40(7):2739–2747. (in Chinese).
梁建文,李方杰,顾晓鲁. 2005. Rayleigh波在浅圆凹陷地形附近的散射:高频解答[J]. 地震工程与工程振动,25(5):26–31. Liang J W,Li F J,Gu X L. 2005. Scattering of Rayleigh waves by a shallow circular canyon:high-frequency solution[J]. Earthquake Engineering and Engineering Vibration,25(5):26–31. (in Chinese).
林皋,关飞. 1990. 用边界元研究地震波在不规则地形处的散射问题[J]. 大连理工大学学报,30(2):145–152. Lin G,Guan F. 1990. Scattering of seismic waves at irregular topographies by boundary element method[J]. Journal of Dalian University of Technology,30(2):145–152. (in Chinese).
刘晶波. 1996. 局部不规则地形对地震地面运动的影响[J]. 地震学报,18(2):239–245. Liu J B. 1996. The impact of local irregular topography on seismic ground motion[J]. Acta Seismologica Sinica,18(2):239–245. (in Chinese).
李伟华,赵成刚. 2003. 圆弧形凹陷饱和土场地对平面P波散射问题的解析解[J]. 地球物理学报,46(4):539–546. doi: 10.3321/j.issn:0001-5733.2003.04.017 LI W H,Zhao C G. 2003. An analytical solution for the diffraction of plane P-waves by circular cylindrical canyons in a fluid-saturated porous media half space[J]. Chinese Journal of Geophysics,46(4):539–546. (in Chinese).
李平,刘红帅,薄景山,李孝波,于晓辉. 2016. 汶川MS8.0地震河谷地形对汉源县城高烈度异常的影响[J]. 地球物理学报,59(1):174–184. Li P,Liu H S,Bo J S,Li X B,Yu X H. 2016. Effects of river valley topography on anomalously high intensity in the Hanyuan town during the Wenchuan MS8.0 earthquake[J]. Chinese Journal of Geophysics,59(1):174–184. (in Chinese).
李平,薄景山,李孝波,肖瑞杰. 2016. 安宁河河谷及邛海地区土层场地对地震动的放大作用[J]. 岩土工程学报,38(2):362–369. Li P,Bo J S,Li X B,Xiao R J. 2016. Amplification effect of soil sites on ground motion in Anning River valley and Qionghai Lake area[J]. Chinese Journal of Geotechnical Engineering,38(2):362–369. (in Chinese).
曲国胜,黄建发,李小军,张晓东,宁宝坤,李亦纲. 2008. 南亚(巴基斯坦)地震灾害分布及成因分析[J]. 震灾防御技术,3(1):85–94. doi: 10.3969/j.issn.1673-5722.2008.01.011 Qu G S,Huang J F,Li X J,Zhang X D,Ning B K,Li Y G. 2008. The Hazard Assessment and Analysis of Pakistan Earthquake in 2005[J]. Technology for Earthquake Disaster Prevention,3(1):85–94. (in Chinese).
沈欣茹,郝冰,李远东,陈珍,周正华. 2023. 河谷地形对地震动的影响分析[J]. 地震学报,45(4):706–16. Shen X R,Hao B,Li Y D,Chen Z,Zhou Z H. 2023. The influence of valley topography on ground motion[J]. Chinese Journal of Geophysics,45(4):706–16. (in Chinese).
孙纬宇,汪精河,严松宏,欧尔峰,梁庆国. 2019. SV波斜入射下河谷地形地震动分布特征分析[J]. 振动与冲击,8(20):237–243+265. Sun W Y,Wang J H,Yan S H,Ou E F,Liang G Q. 2019. Characteristic analysis of ground motions of a canyon topography under obliquely incident SV waves[J]. Journal of Vibration and Shock,8(20):237–243+265. (in Chinese).
王铭锋,郑傲,章文波. 2017. 局部山体地形对强地面运动的影响研究[J]. 地球物理学报,60(12):4655–4670. doi: 10.6038/cjg20171210 Wang M F,Zheng A,Zhang W B. 2017. Effect of local mountain topography on strong ground motion[J]. Chinese Journal of Geophysics,60(12):4655–4670. (in Chinese).
王伟. 2011. 地震动的山体地形效应[D]. 哈尔滨:中国地震局工程力学研究所,10−14. Wang W. 2011. Effect of Hill Topography on Ground Motion[D]. Harbin:Institute Of Engineering Mechanics,China Earthquake Administration,10−14. (in Chinese).
肖文海. 2009. 大型河谷场地地震动特征研究[D]. 哈尔滨:中国地震局工程力学研究所,1. Xiao W H. 2009. Research on Ground Motion Characteristic at the Site of Large-scale ValleyD]. Harbin:Institute Of Engineering Mechanics,China Earthquake Administration,1. (in Chinese).
章小龙,李小军,周正华,陈国兴,彭小波. 2017. 三维复杂山谷地形SV波垂直入射地震反应分析[J]. 地球物理学报,60(7):2779–2790. doi: 10.6038/cjg20170723 Zhang X L,Li X J,Zhou Z H,Chen G X,Peng X B. 2017. The seismic response analysis of three-dimensional Canyon comples topography under incident SV seismic waves[J]. Chinese Journal of Geophysics,60(7):2779–2790. (in Chinese).
赵纪生,吴景发,师黎静,王伟. 2009. 汶川地震地表破裂周围建筑物重建的避让距离[J]. 地震工程与工程振动,29(6):96–101. Zhao J S,Wu J F,Shi L J,Wang W. 2009. Setback distance determination in reconstruction along the trace of surface rupture caused by MS8.0 Wenchuan earthquake[J]. Earthquake Engineering and Engineering Vibration,29(6):96–101. (in Chinese).
周国良,李小军,侯春林,李铁萍. 2012. SV波入射下河谷地形地震动分布特征分析[J]. 岩土力学,33(4):1161–1166. doi: 10.3969/j.issn.1000-7598.2012.04.029 Zhou G L,Li X J,Hou C L,Li T P. 2012. Characteristic analysis of ground motions of canyon topography under incident SV seismic waves[J]. Rock and Soil Mecanics,33(4):1161–1166. (in Chinese).
Boore D M. 1972. A note on the effect of simple topography on seismic SH waves[J]. Bulletin of the seismological Society of America,62(1):275–284. doi: 10.1785/BSSA0620010275
Bouckovalas G D,Papadimitriou A G. 2005. Numerical evaluation of slope topography effects on seismic ground motion[J]. Soil Dynamics and Earthquake Engineering,25(7):547–558.
Frischknecht C,Wagner JJ. 2004. Seismic soil effect in an embanked deep Alpine Valley:A numerical investigation of two-dimensional resonance[J]. Bulletin of the Seismological Society of America,94(1):171–186. doi: 10.1785/0120020158
Davis L L,West L R. 1973. Observed effects of topography on ground motion[J]. Bulletin of the Seismological Society of America,63(1):283–298. doi: 10.1785/BSSA0630010283
Gao Y,Zhang N,Li D,Liu H,Cai Y,Wu Y. 2012. Effects of topographic amplification induced by a U-shaped canyon on seismic waves[J]. Bulletin of the Seismological Society of America,102(4):1748–1763.
Kurita T,Annaka T,Takahashi S,Shimada M,Suehiro T. 2005. Effect of irregular topography on strong ground motion amplification[J]. Journal of Japan Association for Earthquake Engineering,5(3):1–11. doi: 10.5610/jaee.5.3_1
Liu Z X,Wang D,Liang J W,Wu C Q. 2018. The fast multi-pole indirect BEM for solving high-frequency seismic wave scattering by three-dimensional superficial irregularities[J]. Eng Anal Boun Elem,90:86–99.
Peng W F,Wang C L,Chen S T,Lee S T. 2009. Incorporating the effects of topographic amplification and sliding areas in the modeling of earthquake-induced landslide hazards,using the cumulative displacement method[J]. Computers &Geosciences,35(5):946–966.
Tessmer E D,Kosloff D. 1994. 3-D elastic modeling with surface topography by a Chebychev spectral method[J]. Geophysics,59(3):464–473.





 下载:
下载: