Geodynamical simulation of the effects of ridge subduction on the scale of the seismogenic zone south of Chile Triple Junction
-
摘要: 智利三联点以北地震较多，智利三联点以南地震很少且热异常显著。为探究洋脊俯冲对由温度定义的孕震区的影响，以智利三联点区域的地质背景为基础建立二维有限元数值模型，对洋脊俯冲过程进行数值模拟，并将俯冲角度和汇聚速率等因素对孕震区的影响进行了对比。结果表明，洋脊俯冲过程中孕震区宽度减小，导致发生在智利三联点以南的地震远少于智利三联点以北。剖面附近的观测数据与数值模拟结果的对比表明，数值模拟可以大致反映智利三联点区域板块间的孕震区宽度和地表热流特征。当俯冲汇聚量相同时，板块间的汇聚速率越大，洋脊俯冲过程中孕震区则越宽且其下边界越深，海沟附近的地表热流越高。与汇聚速率相比，俯冲角度等因素对地表热流的影响较小。俯冲角度越大，洋脊俯冲过程中孕震区越窄。当数值模型包含剪切生热时，洋脊俯冲过程中孕震区宽度大约可减小至15 km，且孕震区很浅。这可导致智利三联点以南部分区域难以发生地震，出现观测不到和达-贝尼奥夫带的现象。Abstract: Earthquakes are much more frequent to the north of Chile Triple Junction than to the south, where the thermal anomaly is also more significant. To study the effects of ridge subduction on the thermally defined seismogenic zone, two-dimensional finite element models were established based on the geology of Chile Triple Junction, the process of ridge subduction was simulated, and the effects of the initial slab dip and the convergence rate on the seismogenic zone were compared. The results show that the width of the seismogenic zone decreases during the ridge subduction, inducing earthquakes to occur much less to the south than to the north of Chile Triple Junction. By comparing the observed data in the vicinity of the profiles with the numerical simulation results, we find that the numerical simulation can roughly reflect the width of the interplate seismogenic zone and the surface heat flow in the area of Chile Triple Junction. At the same convergence distance, a larger convergence rate comes with a wider seismogenic zone and the deeper the downdip limit of the seismogenic zone, the higher the surface heat flow in the vicinity of the trench. Compared with the convergence rate, factors such as slab dip have little effect on the surface heat flow. In the process of ridge subduction, larger the slab dips leads to narrower seismogenic zones. When the effect of shear heating is included in the simulation, the width of the seismogenic zone in the process of ridge subduction can shrink to about 15 km and the depth of the seismogenic zone is small. Such a narrow and shallow seismogenic zone makes it hard for earthquakes to occur and for the Wadati-Benioff plane to be observed in some areas south of Chile Triple Junction.
-
Keywords:
- Chile Triple Junction /
- ridge subduction /
- seismogenic zone /
- numerical simulation
-
引言
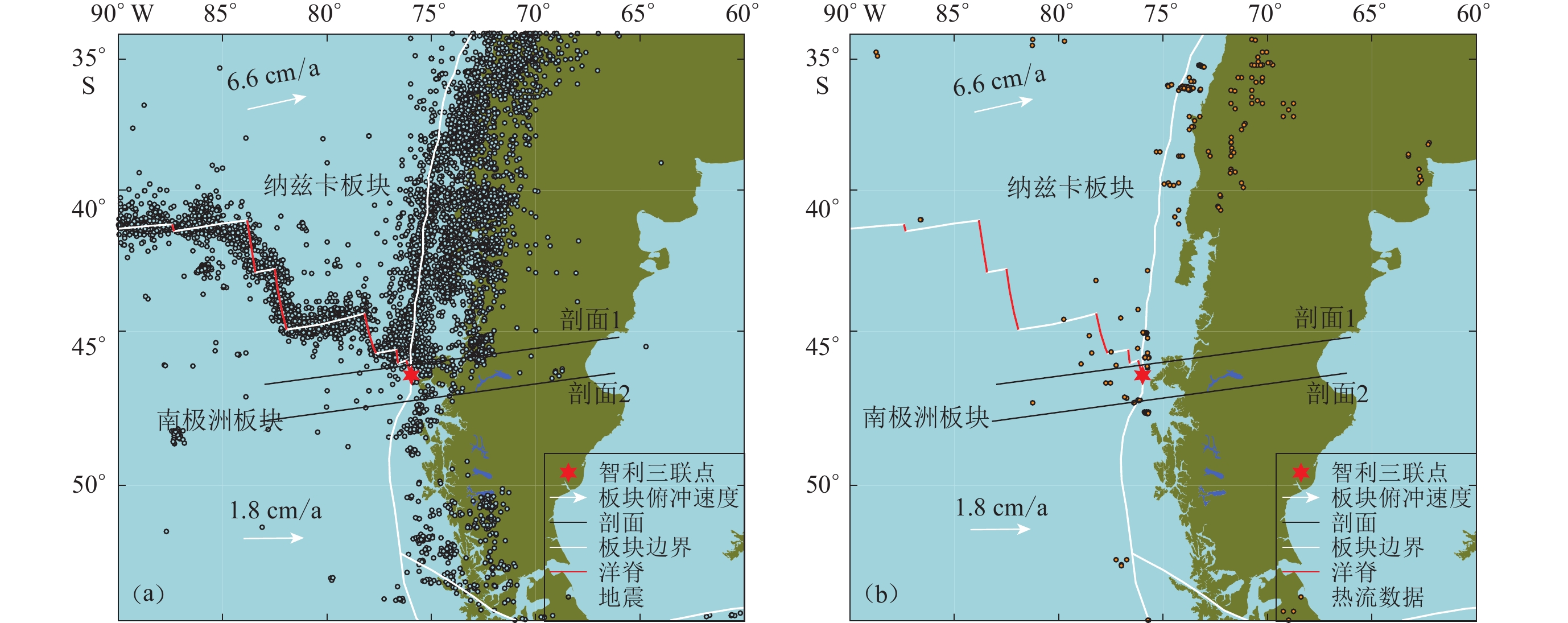
洋脊与俯冲带相互作用导致洋脊消亡是常见的地球动力学过程，对应于威尔逊旋回中的大洋闭合阶段（沈晓明等，2010）。当扩张洋脊俯冲时便形成了洋脊-海沟-海沟（ridge-trench-trench，缩写为RTT）型三联点。智利三联点（Chile Triple Junction，缩写为CTJ）（约46°30′S，75°45′W），是典型的RTT型三联点，位于纳兹卡板块、南极洲板块和南美板块的交界处（王振山，魏东平，2018）。
智利洋脊大致平行于智利海沟（图1），一系列转换断层将其分割为长度不同的洋脊区段（Tebbens et al，1997）。从14 Ma至今，洋脊区段和转换断层交替俯冲至南美板块之下，CTJ的类型也随之转变（贾鸿瑞，魏东平，2021）：当转换断层俯冲时，CTJ的三个边界分别为转换断层-海沟-海沟（fault-trench-trench，缩写为FTT）型三联点；当洋脊区段俯冲时，CTJ为RTT型三联点。洋脊区段俯冲至大陆板块之下继续扩张，大洋板块边界会被热的地幔包围，导致板块边界停止生长，板片窗在深部发育（Bourgois，Michaud，2002）。巴塔哥尼亚板片窗是智利洋脊长期俯冲的结果之一。Breitsprecher和Thorkelson （2009）基于运动学假设给出了巴塔哥尼亚板片窗的演化过程，并推断现今巴塔哥尼亚板片窗的南、北边界分别位于里奥穆塔（Rio Murta）和赛罗哈德孙（Cerro Hudson volcanic centers）地区。
![]()
图 1 智利三联点区域构造背景及观测数据（a） 智利三联点区域1906—2021年地震分布，数据来自国际地震中心（Bondár，Storchak，2011；Storchak et al，2017，2020）；（b） 智利三联点区域热流数据分布（Lucazeau，2019）Figure 1. Geotectonic background of the Chile Triple Junction and the observed data（a） Earthquake distribution in the area of CTJ from 1906 to 2021，the seismicity data are from the International SeismologicalCenter （Bondár，Storchak，2011；Storchak et al，2017，2020）；（b） The heat flow data in the area of CTJ （Lucazeau，2019）受洋脊俯冲等因素影响，CTJ以南的南美大陆热异常比较显著（Hamza et al，2005；Goddard，Fosdick，2019）。Lagabrielle等（2000）基于Taitao半岛的钻孔资料和地质背景推测，在CTJ以南50 km内，由于该区域在6 Ma以来接连受到两次洋脊俯冲影响，部分区域在较浅处就可能达到850—950℃的高温。此外，俯冲带的热异常通常与多种因素相关，但与板块年龄、板块之间的汇聚速率、俯冲角度、地幔楔的性质、上覆板块厚度等因素相比，洋脊俯冲的影响可能占主导地位（Guo et al，2021）。
近年来对地震各向异性的研究表明，三联点区域的地形、岩石层热结构及地震活动性十分复杂（Eakin et al，2010）。例如，CTJ以南地震很少，CTJ以北地震相对较多（Murdie et al，1993；Bohm et al，2002）（图1a）。在43°S以南，震源深度普遍较浅，且未观测到明显的贝尼奥夫（Wadati-Benioff）带。据推测，上述现象可能与该地区温度较高且洋壳年龄很小，难以孕育深源地震有关（Kirby et al，1996；Lange et al，2007；Agurto-Detzel et al，2014）。在43°S以北，贝尼奥夫带较为明显（Agurto-Detzel et al，2014）。
板块间地震活动性与板块边界的运动情况紧密相关（Wei，Seno，1998）。在CTJ以北，纳兹卡板块向南美板块俯冲，速率约为6.6 cm/a；在CTJ以南，南极洲板块向南美板块俯冲，速率约为1.8 cm/a （Maksymowicz et al，2012）。尽管影响板块间地震分布的因素较多，例如，俯冲带的应力状态及流体压强、沉积层的俯冲、俯冲板块与上覆板块的耦合程度等（张克亮，魏东平，2011，Völker et al，2011；Zhang，Wei，2012），但相关研究表明，控制板块间地震活动性分布的首要因素是温度（Tichelaar，Ruff，1991；Oleskevich et al，1999；Völker et al，2011）。Klotz等（2006）根据板块间接触面的温度定义了孕震区宽度，且通过该定义计算的孕震区宽度在诸多俯冲带与地震活动性数据相符，包括墨西哥俯冲带、阿拉斯加俯冲带、智利俯冲带等（Tichelaar，Ruff，1991；Oleskevich et al，1999；Völker et al，2011）。Guo等（2021）将CTJ以南南极洲板片的俯冲定义为楔俯冲（wedge subduction），即年龄较小且较薄的板片区段先俯冲，年龄较大且较厚的板片区段后俯冲的俯冲模式，并讨论了楔俯冲对CTJ以南岩石层热结构及孕震区的影响。此外，作者基于楔俯冲对孕震区宽度的影响推测，洋脊俯冲可能是造成CTJ以南部分区域观测不到明显贝尼奥夫带的主要原因，但缺少直接证据。目前，洋脊俯冲对CTJ区域地震活动性分布的影响多基于理论推测，相关数值模拟和实验很少，亟待进一步开展研究。
本文拟以CTJ区域的地质背景为基础，以地表热流、地震活动性等观测资料为约束，采用有限元软件ASPECT建立二维简化数值模型，对伴随洋脊的大洋板块向大陆板块的俯冲过程进行数值模拟，讨论洋脊俯冲及汇聚速率、俯冲角度等控制因素对CTJ区域孕震区尺度的影响，以期对洋脊俯冲过程及洋脊俯冲区域的地震活动分布有进一步的认识。
1. 数值模型与参数设置
采用有限元数值算法“ASPECT”建立二维简化数值模型（Kronbichler et al，2012；Heister et al，2017；Rose et al，2017；Fraters et al，2019；Bangerth et al，2020）。“ASPECT”算法主要求解动量守恒、质量守恒和热量守恒方程，具体可参见刘梦雪等（2019）。
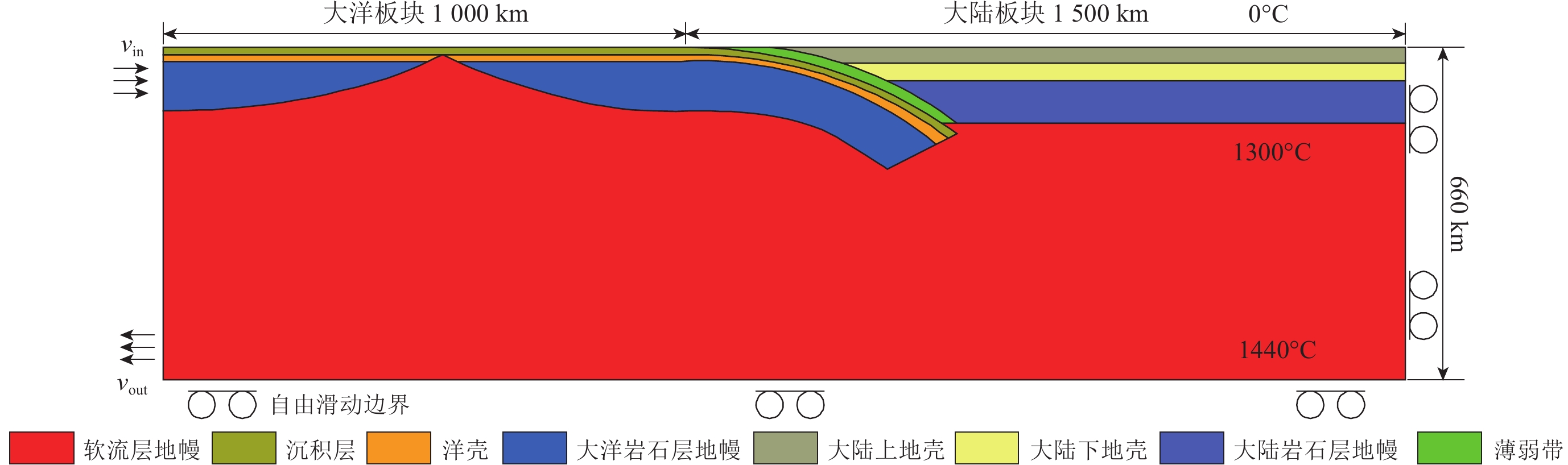
以智利三联点（CTJ）区域的地质背景为基础建立一组包含洋脊的大洋板块向大陆板块俯冲的二维地球动力学数值模型。模型的平面尺度均为2 500 km×660 km，其中大陆板块的上表面长度为1 500 km，大洋板块的上表面长度为1 000 km。根据CTJ区域的层析成像及前人在CTJ区域建立的相关数值模型（van der Hilst，Hoop，2005；Scherwath et al，2006，2009；Simmons et al，2010；Völker et al，2011；Maksymowicz et al，2012；徐佳静等，2019），将参考模型中板片的初始俯冲角度设置如下：在海沟处为0°，随深度逐渐增大，至岩石层底部附近增至约30°。
参考模型的边界条件设置为：在模型的左边界上部施加与大洋板块等厚度的速度边界条件，初始时刻速度设置为6.6 cm/a，以模拟板块的持续汇聚作用；左边界下部施加相同大小的流出速度（刘梦雪等，2019）；模型的上边界为自由表面；右、下边界均为自由滑动边界（图2）。初始温度场设置为：大洋板块及大陆板块的上边界温度为0℃，下边界均设为1 300℃ （Tetreault，Buiter，2012；Shi et al，2018；史亚男等，2019）。大洋板块的初始温度模型采用板块冷却模型（plate model），将其最大厚度设为95 km，大洋板块的岩石层厚度及初始温度场T（x，y）根据板块年龄计算得到（Stein，Stein，1992；Richards et al，2018）：
![]()
图 2 参考模型初始设置及边界条件这些参数由干橄榄岩（Hirth，Kohlstedt，2004）的位错蠕变和扩散蠕变的流变实验获得Figure 2. Initial setups and boundary conditions of the reference modelAll these parameters come from the rheological experiments on dislocation creep and diffusion creep of dry olivine （Hirth，Kohlstedt，2004）$$ T （ x，y ） ＝{T}_{\mathrm{m}}\left[\frac{y}{a}＋\sum _{n＝1}^{\mathrm{\infty }}\frac{2}{n\mathrm{\pi }}\mathrm{exp}\left(-\frac{{\beta }_{n}x}{a}\right)\mathrm{s}\mathrm{i}\mathrm{n}\left(\frac{n\mathrm{\pi }y}{a}\right)\right]， $$ (1) 式中：x和y为横、纵坐标；a为板块的最大厚度；Tm为板块下边界温度；$\;{\beta _n} ＝ \sqrt { {{R^2} ＋ {n^2}{\pi ^2}} } － R$，R＝va/（2κ），κ为热扩散系数，v为洋脊的半扩张速率。将大陆板块的岩石层厚度设为100 km，其中上、下地壳的厚度均设为20 km （Assumpção et al，2013；Bagherbandi et al，2017； 刘梦雪等，2019）。大陆板块的初始温度场采用线性插值的方法（李忠海，石耀霖，2016），将莫霍面温度设置为600℃。地幔中采用线性温度梯度0.25℃/km （Tetreault，Buiter，2012），通过计算可得模型底部温度约为1440℃。各层的力学参数参考相关研究进行选取，具体信息列于表1 （Völker et al，2011，刘梦雪等，2019；徐佳静等，2019）。
表 1 数值模型的相关参数Table 1. Relevant parameters of numerical model物质名称 厚度
/km热扩散率κ
/10−6 （m2·s−1）比热容Cp
/（J·kg−1· K−1）密度ρ
/（kg·m−3）热膨胀系数
α/10−5 K−1内摩擦角
φ/°黏聚力
C/106 Pa生热率A
/（W·m−3）流变
准则①晶粒大小
指数m地幔 660 0.989 750 3 300 2 20 20 干橄榄岩 3 大陆上地壳 20 1.21 750 2 800 2 20 20 10−6 湿石英 1 大陆下地壳 20 1.15 750 2 900 2 20 20 4×10−7 湿钙长石 1 岩石层地幔 0—87 9.87 750 3 300 2 20 20 干橄榄岩 3 沉积层 4 1.21 750 3 000 2 5 10 辉长岩 1 大洋地壳 8 1.15 750 3 100 2 20 10 辉长岩 1 薄弱带 1.21 750 3 300 2 0.03 1 辉长岩 1 物质名称 前因子② （Pa−n·m−p·s−1） 应力指数n 活化能E/103 （J·mol−1） 活化体积V/10−6 （m3·mol−1） 扩散蠕变 位错蠕变 扩散蠕变 位错蠕变 扩散蠕变 位错蠕变 扩散蠕变 位错蠕变 地幔 2.37×10−15 6.52×10−16 1 3.5 375 530 4 13 大陆上地壳 1×10−50 8.57×10−28 1 4.0 0 223 0 0 大陆下地壳 1×10−50 7.13×10−18 1 3.0 0 345 0 0 岩石层地幔 2.37×10−15 6.52×10−16 1 3.5 375 530 4 18 沉积层 1×10−50 1.12×10−10 1 3.4 0 497 0 0 大洋地壳 1×10−50 1.12×10−10 1 3.4 0 497 0 0 薄弱带 1×10−50 1.12×10−10 1 3.4 0 497 0 0 注：① 流变准则：干橄榄岩来自Hirth和Kohlstedt （2004）；湿石英来自Gleason和Tullis （1995）；湿钙长石来自Rybacki （2006）；辉长岩来自Wilks和Carter （1990）。② 黏滞系数的前因子来自平面应变单轴试验结果（Naliboff，Buiter，2015；刘梦雪等，2019）. 2. 数值模拟结果
2.1 参考模型
图3为包含洋脊的大洋板块向大陆板块的俯冲过程。由于初始模型中存在板片（图2），故根据洋脊的半扩张速率2.4 cm/a及板片的长度对板片加热，通过计算得到俯冲板片的初始温度场，将计算完成时刻计为0 Ma （图3a）。随后，根据纳兹卡板块和南极洲板块向南美板块的汇聚速率设置参考模型的汇聚速率：在0—3 Ma （图3 t＝0 Ma），洋脊向海沟方向汇聚，将模型中板块的汇聚速率设置为纳兹卡板块向南美板块的汇聚速率，即6.6 cm/a；模型运行至3 Ma时洋脊已临近海沟，在3—19.5 Ma （图3a），洋脊开始向南美板块下方俯冲，将汇聚速率设置为南极洲板块向南美板块的汇聚速率，即1.8 cm/a。参考模型未考虑剪切生热，在6—10 Ma，洋脊与海沟首次接触（表2）。
![]()
图 3 参考模型成分场（a）和黏滞系数η场的演化（b）图中橙色实线为等温线，最低为100 ℃，最高为1 250 ℃Figure 3. Evolutions of the material field （a） and the viscosity coefficient η field （b） of the reference modelOrange solid lines are isotherms，with a minimum of 100 ℃ and a maximum of 1 250 ℃表 2 参考模型孕震区宽度演化Table 2. Evolution of the width of the seismogenic zone of the reference model时间/Ma 闭锁区宽度/km
x（350℃）－x（100℃）过渡带宽度/km
x（450℃）－x（350℃）孕震区宽度/km
x（350℃）－x（100℃） ＋0.5× ［ x（450℃） －x（350℃） ］洋脊与海沟横
坐标差/km0 86 36 104 −164 3 70 31 86 −60 6 89 34 106 −13 10 68 44 90 32 15 52 39 72 78 19.5 49 34 66 112 有理论表明，板块间的孕震区主要受温度控制（Tichelaar，Ruff，1991；Oleskevich et al，1999；Völker et al，2011）。孕震区的宽度与板块间地震活动性分布紧密相关。智利区域（Tichelaar，Ruff，1991；Oleskevich et al，1999）及CTJ以北的纳兹卡俯冲带（Völker et al，2011）的地震分布与该理论吻合较好。在俯冲板片与上覆板块的接触面上，Klotz等（2006）根据温度作如下定义：① 100—350℃是地震的闭锁区；② 350—450℃是闭锁区到滑动区的过渡带；③ 闭锁区的宽度加上过渡带宽度的一半是孕震区（Völker et al，2011）。
根据图3中100℃，350℃和450℃等温线与板片上边界（沉积层上表面）交点的横坐标，表2统计了参考模型在洋脊俯冲过程中闭锁区、过渡带和孕震区宽度的演化。可以发现，在洋脊俯冲过程中岩石层地幔底部黏度有所减小（图3b），大洋板块和上覆大陆板块间孕震区的宽度持续减小，且其宽度小于洋脊俯冲之前（表2）。在0 Ma，孕震区宽度约为104 km；在洋脊向海沟方向汇聚的过程中，孕震区宽度先减小后增大，至6 Ma时其宽度约为106 km；至19.5 Ma洋脊距海沟的水平距离约为112 km，孕震区宽度约减小至66 km，比0 Ma时约减小了38 km。这表明，洋脊俯冲导致了孕震区宽度减小，影响了CTJ区域的地震分布。因此，在CTJ以南，受智利洋脊俯冲影响，该区域地震必然少于CTJ以北。CTJ区域的地震分布（图1a）明显可见上述差异。
2.2 汇聚速率对洋脊俯冲过程中孕震区宽度的影响
智利洋脊俯冲至南美板块之下会继续扩张，发育为巴塔哥尼亚板片窗。由于俯冲至南美板块下方的洋脊扩张速率难以确定，因此建立了不同汇聚速率的洋脊俯冲模型，以探究汇聚速率对洋脊俯冲过程中板块间孕震区宽度的影响。在洋脊俯冲过程中，参考模型的汇聚速率为1.8 cm/a，为慢速汇聚模型。在此基础上，分别建立了汇聚速率为6.6 cm/a的快速汇聚模型和介于二者之间汇聚速率为4.2 cm/a的模型。
图4为不同汇聚速率模型在俯冲汇聚量相同时的成分场和等效黏滞系数场。可以看出，当俯冲汇聚量相同时，即汇聚速率为1.8，4.2和6.6 cm/a的三个模型分别在19.5 Ma，10 Ma和7.5 Ma时，三者的孕震区宽度分别约为66，101和113 km。这表明，汇聚速率越大，板块间的孕震区越宽。此外，不同汇聚速率模型的孕震区宽度在洋脊俯冲过程中均呈减小趋势。
![]()
图 4 不同汇聚速率模型在俯冲汇聚量相同时的成分场（a）和黏滞系数场η（b）Figure 4. The material field （a） and the viscosity field η （b） of different convergence rate models when the convergence distances are the same2.3 俯冲角度对洋脊俯冲过程中孕震区宽度的影响
CTJ以北的层析成像证据表明，纳兹卡板块的俯冲角度向北呈增大趋势（van der Hilst，Hoop，2005；Scherwath et al，2006，2009；Contreras-Reyes et al，2010；Maksymowicz et al，2012）。CTJ以南层析成像的证据很少，南极洲板块俯冲角度尚不明确。Breitsprecher和Thorkelson（2009）基于运动学假设推测CTJ附近南极洲板片的俯冲角度很小，约为10—13°。参考模型的俯冲角度为30°，在此基础上建立了初始俯冲角度分别为15°和45°的数值模型，探究俯冲角度对洋脊俯冲过程中孕震区宽度的影响。
图5为不同初始俯冲角度模型在19.5 Ma时的成分场和等效黏滞系数场。表3给出了当俯冲角度分别为15°，30°和45°时洋脊俯冲过程中孕震区宽度的演化。可以发现，在6 Ma时，俯冲角度为45°模型的洋脊已经开始俯冲，其余两个模型的洋脊尚未与海沟接触。这表明，当汇聚速率相同时，俯冲角度越大洋脊越早开始俯冲。此外，在6 Ma时，俯冲角度为15°，30°和45°模型的孕震区宽度分别约为85 km，106 km和74 km。其中俯冲角度为45°模型的孕震区宽度最小，这是由于6 Ma时该模型的洋脊已经开始俯冲，其距海沟的水平距离约为8 km，孕震区宽度随着洋脊俯冲有所减小。在19.5 Ma时，俯冲角度为15°，30°和45°模型中孕震区宽度分别约为80 km，66 km和52 km。这表明，当俯冲时间相同时，俯冲角度越大，洋脊俯冲过程中孕震区的宽度越小。
![]()
图 5 不同初始俯冲角度模型在19.5 Ma时的成分场（a）和黏滞系数场η（b）Figure 5. The material field （a） and the viscosity field η （b） of models of different initial slab dips at 19.5 Ma表 3 不同初始俯冲角度模型孕震区宽度演化Table 3. Evolution of the width of the seismogenic zone of models of different initial slab dips时间/Ma 孕震区宽度/km 洋脊与海沟横坐标差/km 15° 30° 45° 15° 30° 45° 0 111 104 66 −145 −164 −158 3 96 86 76 −67 −60 −36 6 85 106 74 −33 −13 8 10 94 90 60 14 32 36 15 90 72 51 46 78 67 19.5 80 66 52 77 112 92 2.4 洋壳参数及剪切生热对洋脊俯冲过程中孕震区宽度的影响
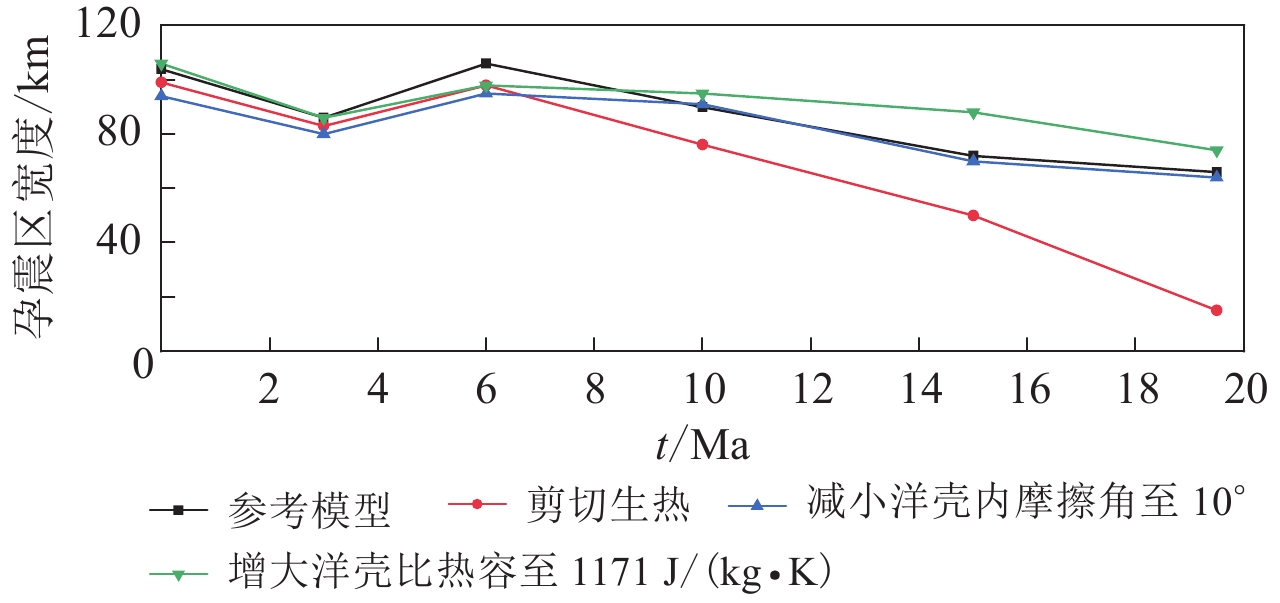
在参考模型的基础上，分别建立洋壳比热容为1 171 J/（kg·K）和洋壳内摩擦角为10°的两个对比模型，探究洋壳参数对洋脊俯冲过程中孕震区宽度的影响。此外还建立了包含剪切生热的对比模型。图6给出了上述三个模型在洋脊俯冲过程中孕震区宽度随时间的演化。可以发现，上述三者中剪切生热对孕震区宽度的影响最大。在19.5 Ma时，包含剪切生热模型的孕震区宽度在洋脊俯冲的过程中由约99 km减小至约15 km；参考模型由约104 km减小至约66 km。这表明，剪切生热对洋脊俯冲过程中孕震区宽度的影响不可忽略。实际上，数值模型中未考虑洋脊区域的地幔热物质上涌，且板块间接触面温度与实际相比偏低，因此数值结果中孕震区的宽度可能偏大。尽管如此，考虑剪切生热的模拟结果中孕震区宽度已减小至约15 km，可以认为洋脊俯冲导致CTJ以南部分区域的地震难以发生，即出现不能观测到贝尼奥夫带的现象。
![]()
图 6 对比模型孕震区宽度的演化Figure 6. Evolution of the width of the seismogenic zone of contrast models由图6可以发现，增大洋壳比热容或内摩擦角均会导致孕震区宽度增大。当洋壳内摩擦角由10°增大至20°时，孕震区宽度在6 Ma时由约95 km增大至约106 km，在19.5 Ma时由约64 km增大至约66 km；当洋壳的比热容由750 J/（kg·K）增大至1 171 J/（kg·K）时，孕震区宽度在10 Ma时由约90 km增大至约95 km，在19.5 Ma时由约66 km增大至约74 km。由此可见，在19.5 Ma时，增大洋壳比热容后模拟的孕震区宽度比参考模型宽8 km；减小洋壳内摩擦角后模拟的孕震区宽度比参考模型窄2 km，可忽略不计。这表明，洋脊俯冲后洋壳内摩擦角和比热容两种参数对孕震区宽度的影响较小。
3. 讨论
3.1 热流数据与模拟结果
对比数值模拟结果与观测数据以测试模型能否反映洋脊俯冲不同阶段的地表热流特征。采用ASPECT软件的后处理功能获得了各模型的地表热流（Gresho et al，1987；Bangerth et al，2020）。选取了剖面附近50 km以内的热流数据投影至剖面与模拟结果进行对比（图1），热流数据来自Lucazeau （2019）。CTJ以南热流数据很少（图1b），根据热流数据的分布，我们选取了CTJ附近的两个剖面，分别为CTJ以北的剖面1 （46°13′S）和CTJ以南的剖面2 （47°35′S）。当洋脊临近海沟时，各模型的板片年龄与剖面1的大致相同；当模型的汇聚速率为1.8 cm/a，t＝19.5 Ma时，模型中俯冲板片的年龄与剖面2的大致相同，但模型的洋脊位置位于实际的板片窗边界附近，模型中洋脊与海沟的距离小于实际距离。这是由于数值模型的两个俯冲板块采用了相同的汇聚速率。
图7a和7b为剖面1附近的地表热流和各模型的热流结果。对比不同汇聚速率模型的地表热流结果与观测数据可以发现（图7a），与热流观测数据最相符的模型既不是以南极洲板块汇聚速率俯冲的慢速俯冲模型（1.8 cm/a），也不是以纳兹卡板块汇聚速率俯冲的快速俯冲模型（6.6 cm/a），而是汇聚速率介于二者之间的模型（4.2 cm/a）。这是由于剖面1位于CTJ附近，剖面上海沟与洋脊的位置基本重合，且剖面附近的热流观测数据多位于智利洋脊附近，但海沟西、东两侧的板块汇聚速率不同，分别为南极洲板块和纳兹卡板片的汇聚速率，而数值模型采用了相同的汇聚速率，因此，采用二者的平均汇聚速率（4.2 cm/a）得到的热流结果与海沟附近的热流观测数据较为一致，该速率约为洋脊中心的俯冲速率。此外，由图7a可以看出，与汇聚速率相比，其它因素对地表热流的影响相对较小，且汇聚速率越大，海沟附近的地表热流越高。
![]()
图 7 海沟附近地表热流分布（a） 剖面1各模型热流结果；（b） 剖面1不同初始俯冲角度模型热流结果（汇聚速率＝4.2 cm/a）；（c） 剖面2不同初始俯冲角度模型热流结果（汇聚速率＝1.8 cm/a）Figure 7. Surface heat flow in the vicinity of the trench（a） Heat flow results of different models of profile 1；（b） Heat flow results of models with different initial slab dips of profile 1 （convergence rate＝4.2 cm/a）；（c） Heat flow results of models with different initial slab dips of profile 2 （convergence rate＝1.8 cm/a）图7b给出了剖面1附近汇聚速率为4.2 cm/a、不同俯冲角度数值模型的地表热流结果；图7c给出了剖面2附近汇聚速率为1.8 cm/a、不同俯冲角度数值模型的地表热流结果，模型的初始俯冲角度分别为15°，30°和45°。可以看出，与汇聚速率相比，俯冲角度对地表热流的影响相对较小且较为复杂，难以一概而论。在图7b中，在海沟附近热流数据较密集处，俯冲角度为45°模型的地表热流略大于其它两个模型的热流数据。此外，尽管图7c中海沟前方的热流结果低于观测数据，但总体上看，本文的数值模拟可以大致反映CTJ区域的地表热流特征。
3.2 地震活动性数据与模拟结果
图8为剖面1和剖面2的深部构造（Simmons et al，2010）。需要指出，由于CTJ以南地震数据很少，该区域的不同层析成像模型相差很大。Maksymowicz等（2012）的层析成像结果表明，在海沟前方150 km内，剖面2附近的俯冲角度约为10°。Breitsprecher和Thorkelson （2009）基于运动学假设推测，CTJ附近南极洲板片的俯冲角度约为10°—13°。上述证据表明，CTJ附近南极洲板片的俯冲角度较小。
![]()
图 8 剖面1（左）和剖面2（右）的P波速度扰动（Simmons et al，2010）Figure 8. Vertical cross sections of P wave velocity perturbations （Simmons et al，2010） along the profile 1 （left） and the profile 2 （right）对比数值模拟结果与地震活动性数据以测试模型能否反映洋脊俯冲不同阶段的孕震区宽度。地震活动性数据（1906—2021）来自ISC （Bondár，Storchak，2011；Storchak et al，2017，2020）。我们选取了海沟前方、剖面1和剖面2附近50 km以内的地震活动性数据与模拟结果进行对比（图9）。根据热流对比结果，剖面1选取了不同俯冲角度、汇聚速率为4.2 cm/a的三个数值模型（图9a）。
![]()
图 9 剖面1 （a）和剖面2 （b）的地震分布及孕震区白色圆点为地震活动性数据。图a汇聚速率为4.2 cm/a，图b汇聚速率为1.8 cm/aFigure 9. Earthquake distributions and seismogenic zones of the profile 1 （a） and the profile 2 （b）Seismicity data are indicated by the white dots. The convergence rate of fig.（a） is 4.2 cm/a and 4.2 cm/a for fig.（b）孕震区宽度的定义仅与板块间地震分布有关（Völker et al，2011），不涉及其它位置。在图9中，剖面1和剖面2附近的地震活动性分布不仅在板块接触面附近较为密集，在孕震区的范围之外也大量存在，其中存在大量火山地震、矿震等（Agurto-Detzel et al，2014）。对比板块间的地震活动性数据与模拟结果表明，与剖面2相比，数值模型的孕震区结果与剖面1附近的地震分布相对一致。对比地震数据与模拟结果表明，在初始俯冲角度分别为15°，30°和45°三个模型中，各模型的孕震区与地震活动性分布差异不大，初始俯冲角度为15°模型（图9a）的孕震区及板片位置与剖面1的地震活动性分布相对一致。剖面2附近的地震相对较少（图9b）。数值模拟结果表明，这是由于洋脊俯冲导致了孕震区变窄。与剖面2附近的地震活动性分布相对一致的仍是初始俯冲角度为15°的模型，初始俯冲角度为30°和45°模型孕震区内的地震相对较少。对比结果表明，本文的数值模拟可以大致反映CTJ区域板块间的孕震区宽度特征，且支持CTJ以南的层析成像结果和南极洲板片以小角度俯冲至南美板块下方的推论（Breitsprecher，Thorkelson，2009；Maksymowicz et al，2012）。
3.3 洋脊俯冲与楔俯冲对孕震区宽度的影响
Guo等（2021）讨论了楔俯冲对CTJ以南孕震区宽度的影响，并推测洋脊俯冲可能是造成CTJ以南部分区域观测不到明显贝尼奥夫带的主要原因。与初始条件相同的板片俯冲模型相比，楔俯冲模型的孕震区宽度比其窄4 km，楔俯冲模型的孕震区宽度约为83 km。当楔俯冲模型的汇聚速率由6.6 cm/a减小至1.8 cm/a时，其孕震区宽度减小至约72 km。除洋脊位置及板块年龄外，本文的参考模型与Guo等（2021）部分板片俯冲模型的初始条件大致相同。本文的模拟结果表明，洋脊俯冲过程中孕震区宽度减小。在6 Ma时，参考模型的孕震区宽度约为106 km，此后洋脊俯冲至上覆板块下方。在19.5 Ma时，参考模型的孕震区宽度减小至约66 km；剪切生热模型的孕震区宽度减小至约15 km。这表明，洋脊俯冲对孕震区宽度的影响较大，可能出现由于孕震区过窄而难以观测到贝尼奥夫带的现象。
3.4 洋脊俯冲对孕震区深度的影响
表4给出了洋脊俯冲过程中部分模型孕震区上、下边界深度的演化。可以发现，在洋脊俯冲过程中（6—19.5 Ma），孕震区下边界深度呈减小趋势。在参考模型中，孕震区下边界深度的变化较小，在6 Ma时，其深度约为74 km，至19.5 Ma时仅减小了4 km。与参考模型相比，剪切生热模型的孕震区上边界深度的变化相对较小，下边界深度在洋脊俯冲过程中大幅度减小，其深度由约73 km （6 Ma）减小至约26 km （19.5 Ma）。此时，孕震区的横向宽度为15 km，纵向宽度仅7 km，即孕震区的横、纵向宽度都很窄，可造成难以观测到贝尼奥夫带的现象。
表 4 部分模型孕震区深度演化Table 4. Evolution of the depth of the seismogenic zone for some models时间/Ma 参考模型 汇聚速率＝6.6 cm/a 俯冲角度＝45° 剪切生热 孕震区上边界
深度/km孕震区下边界
深度/km孕震区上边界
深度/km孕震区下边界
深度/km孕震区上边界
深度/km孕震区下边界
深度/km孕震区上边界
深度/km孕震区下边界
深度/km0 7 47 7 47 8 39 8 43 3 23 70 23 70 12 74 24 67 6 14 74 18 94 7 71 11 73 10 17 71 17 91 10 60 11 66 15 17 69 − − 5 51 9 51 19.5 19 70 − − 1 47 19 26 与参考模型相比，当模型的初始俯冲角度增大至45°时，孕震区下边界深度在洋脊俯冲过程中明显减小，在19.5 Ma时其深度可减小至约47 km。与参考模型相比，该模型的孕震区上、下边界深度均有所减小，即俯冲角度越大，洋脊俯冲过程中孕震区越浅。此外，当俯冲汇聚量相同时，汇聚速率越大，孕震区下边界则越深，孕震区的纵向宽度越宽。与参考模型相比，当汇聚速率增大至6.6 cm/a时，孕震区上边界的变化较小，下边界深度明显增大，在6 Ma和10 Ma时其下边界深度均增大了约20 km。这与Guo等（2021）的结果基本一致，即楔俯冲的汇聚速率越大，孕震区越深。
3.5 局限性
洋脊俯冲涉及多种物理过程和地质过程，包括岩浆作用、变质作用、盆地演化等（Sisson et al，2003），是复杂的三维问题。CTJ是典型的洋脊俯冲带，且洋脊被一系列转换断层分割为长度不同的洋脊区段，构造背景尤其复杂，涉及板片窗的演化、转换断层俯冲以及侵蚀等过程。本研究建立了二维数值模型探究洋脊俯冲对孕震区造成的影响，并以CTJ区域的地质背景为例进行探讨，并不能完全反应空间上该区域孕震区分布的复杂性，也未能综合考虑洋脊俯冲过程中的上述多种过程，仅能表明洋脊俯冲及部分控制因素对孕震区的影响。
孕震区的定义（Klotz et al，2006）主要涉及板片边界附近的构造地震，而CTJ附近的地震不仅涉及构造地震，还包括矿震、火山地震等多种形态（Agurto-Detzel et al，2014）。在图9中，数值模拟得到的板片边界附近的孕震区结果和地震分布存在一定的差异，其中不仅存在数值模拟与实际俯冲过程的差异，还包括火山地震等对结果的影响。此外，孕震区的定义与真实的地震机理必然存在一定的差异，孕震区的定义表明，温度是控制孕震区的首要因素，但具体的孕震机理及适用范围仍需要进一步开展研究。
4. 结论
本文以地表热流、地震活动性等观测资料为约束，基于智利三联点区域的构造特征建立了二维有限元模型，对洋脊俯冲过程进行了数值模拟，得到了洋脊俯冲过程中孕震区宽度的演化，讨论了汇聚速率、俯冲角度等因素的影响，主要结论如下：
1） 洋脊俯冲导致孕震区宽度减小，影响了智利三联点区域的地震分布，造成智利三联点以南的地震远少于智利三联点以北。洋壳内摩擦角和比热容对孕震区宽度的影响相对较小，剪切生热对孕震区宽度的影响较大。当考虑剪切生热时，洋脊俯冲过程中孕震区宽度可减小至约15 km，且孕震区很浅。此时，洋脊俯冲已经导致CTJ以南部分区域的地震难以发生，出现部分区域观测不到贝尼奥夫带的现象。
2） 对比数值模拟结果与选取的剖面附近的观测数据表明，本文的数值模拟可以大致反映智利三联点区域板块间的孕震区宽度和地表热流特征。当俯冲角度为15°时，模拟结果中孕震区宽度及板片位置与地震活动性数据相对一致。
3） 当俯冲汇聚量相同时，板块间的汇聚速率越大，则孕震区越宽且孕震区下边界越深。地表热流受汇聚速率的影响较大，受俯冲角度等因素的影响较小。板片的初始俯冲角度越大，洋脊俯冲过程中孕震区越窄。
本文的研究结果为洋脊俯冲区域的地震活动性分布特征提供了一定的数值模拟依据。
-
![]()
图 1 智利三联点区域构造背景及观测数据
（a） 智利三联点区域1906—2021年地震分布，数据来自国际地震中心（Bondár，Storchak，2011；Storchak et al，2017，2020）；（b） 智利三联点区域热流数据分布（Lucazeau，2019）
Figure 1. Geotectonic background of the Chile Triple Junction and the observed data
（a） Earthquake distribution in the area of CTJ from 1906 to 2021，the seismicity data are from the International SeismologicalCenter （Bondár，Storchak，2011；Storchak et al，2017，2020）；（b） The heat flow data in the area of CTJ （Lucazeau，2019）
![]()
图 2 参考模型初始设置及边界条件
这些参数由干橄榄岩（Hirth，Kohlstedt，2004）的位错蠕变和扩散蠕变的流变实验获得
Figure 2. Initial setups and boundary conditions of the reference model
All these parameters come from the rheological experiments on dislocation creep and diffusion creep of dry olivine （Hirth，Kohlstedt，2004）
![]()
图 3 参考模型成分场（a）和黏滞系数η场的演化（b）
图中橙色实线为等温线，最低为100 ℃，最高为1 250 ℃
Figure 3. Evolutions of the material field （a） and the viscosity coefficient η field （b） of the reference model
Orange solid lines are isotherms，with a minimum of 100 ℃ and a maximum of 1 250 ℃
![]()
图 4 不同汇聚速率模型在俯冲汇聚量相同时的成分场（a）和黏滞系数场η（b）
Figure 4. The material field （a） and the viscosity field η （b） of different convergence rate models when the convergence distances are the same
![]()
图 5 不同初始俯冲角度模型在19.5 Ma时的成分场（a）和黏滞系数场η（b）
Figure 5. The material field （a） and the viscosity field η （b） of models of different initial slab dips at 19.5 Ma
![]()
图 6 对比模型孕震区宽度的演化
Figure 6. Evolution of the width of the seismogenic zone of contrast models
![]()
图 7 海沟附近地表热流分布
（a） 剖面1各模型热流结果；（b） 剖面1不同初始俯冲角度模型热流结果（汇聚速率＝4.2 cm/a）；（c） 剖面2不同初始俯冲角度模型热流结果（汇聚速率＝1.8 cm/a）
Figure 7. Surface heat flow in the vicinity of the trench
（a） Heat flow results of different models of profile 1；（b） Heat flow results of models with different initial slab dips of profile 1 （convergence rate＝4.2 cm/a）；（c） Heat flow results of models with different initial slab dips of profile 2 （convergence rate＝1.8 cm/a）
![]()
图 8 剖面1（左）和剖面2（右）的P波速度扰动（Simmons et al，2010）
Figure 8. Vertical cross sections of P wave velocity perturbations （Simmons et al，2010） along the profile 1 （left） and the profile 2 （right）
![]()
图 9 剖面1 （a）和剖面2 （b）的地震分布及孕震区
白色圆点为地震活动性数据。图a汇聚速率为4.2 cm/a，图b汇聚速率为1.8 cm/a
Figure 9. Earthquake distributions and seismogenic zones of the profile 1 （a） and the profile 2 （b）
Seismicity data are indicated by the white dots. The convergence rate of fig.（a） is 4.2 cm/a and 4.2 cm/a for fig.（b）
表 1 数值模型的相关参数
Table 1 Relevant parameters of numerical model
物质名称 厚度
/km热扩散率κ
/10−6 （m2·s−1）比热容Cp
/（J·kg−1· K−1）密度ρ
/（kg·m−3）热膨胀系数
α/10−5 K−1内摩擦角
φ/°黏聚力
C/106 Pa生热率A
/（W·m−3）流变
准则①晶粒大小
指数m地幔 660 0.989 750 3 300 2 20 20 干橄榄岩 3 大陆上地壳 20 1.21 750 2 800 2 20 20 10−6 湿石英 1 大陆下地壳 20 1.15 750 2 900 2 20 20 4×10−7 湿钙长石 1 岩石层地幔 0—87 9.87 750 3 300 2 20 20 干橄榄岩 3 沉积层 4 1.21 750 3 000 2 5 10 辉长岩 1 大洋地壳 8 1.15 750 3 100 2 20 10 辉长岩 1 薄弱带 1.21 750 3 300 2 0.03 1 辉长岩 1 
下载: 导出CSV
物质名称 前因子② （Pa−n·m−p·s−1） 应力指数n 活化能E/103 （J·mol−1） 活化体积V/10−6 （m3·mol−1） 扩散蠕变 位错蠕变 扩散蠕变 位错蠕变 扩散蠕变 位错蠕变 扩散蠕变 位错蠕变 地幔 2.37×10−15 6.52×10−16 1 3.5 375 530 4 13 大陆上地壳 1×10−50 8.57×10−28 1 4.0 0 223 0 0 大陆下地壳 1×10−50 7.13×10−18 1 3.0 0 345 0 0 岩石层地幔 2.37×10−15 6.52×10−16 1 3.5 375 530 4 18 沉积层 1×10−50 1.12×10−10 1 3.4 0 497 0 0 大洋地壳 1×10−50 1.12×10−10 1 3.4 0 497 0 0 薄弱带 1×10−50 1.12×10−10 1 3.4 0 497 0 0 注：① 流变准则：干橄榄岩来自Hirth和Kohlstedt （2004）；湿石英来自Gleason和Tullis （1995）；湿钙长石来自Rybacki （2006）；辉长岩来自Wilks和Carter （1990）。② 黏滞系数的前因子来自平面应变单轴试验结果（Naliboff，Buiter，2015；刘梦雪等，2019）.
下载: 导出CSV
表 2 参考模型孕震区宽度演化
Table 2 Evolution of the width of the seismogenic zone of the reference model
时间/Ma 闭锁区宽度/km
x（350℃）－x（100℃）过渡带宽度/km
x（450℃）－x（350℃）孕震区宽度/km
x（350℃）－x（100℃） ＋0.5× ［ x（450℃） －x（350℃） ］洋脊与海沟横
坐标差/km0 86 36 104 −164 3 70 31 86 −60 6 89 34 106 −13 10 68 44 90 32 15 52 39 72 78 19.5 49 34 66 112
下载: 导出CSV
表 3 不同初始俯冲角度模型孕震区宽度演化
Table 3 Evolution of the width of the seismogenic zone of models of different initial slab dips
时间/Ma 孕震区宽度/km 洋脊与海沟横坐标差/km 15° 30° 45° 15° 30° 45° 0 111 104 66 −145 −164 −158 3 96 86 76 −67 −60 −36 6 85 106 74 −33 −13 8 10 94 90 60 14 32 36 15 90 72 51 46 78 67 19.5 80 66 52 77 112 92
下载: 导出CSV
表 4 部分模型孕震区深度演化
Table 4 Evolution of the depth of the seismogenic zone for some models
时间/Ma 参考模型 汇聚速率＝6.6 cm/a 俯冲角度＝45° 剪切生热 孕震区上边界
深度/km孕震区下边界
深度/km孕震区上边界
深度/km孕震区下边界
深度/km孕震区上边界
深度/km孕震区下边界
深度/km孕震区上边界
深度/km孕震区下边界
深度/km0 7 47 7 47 8 39 8 43 3 23 70 23 70 12 74 24 67 6 14 74 18 94 7 71 11 73 10 17 71 17 91 10 60 11 66 15 17 69 − − 5 51 9 51 19.5 19 70 − − 1 47 19 26
下载: 导出CSV
-
贾鸿瑞,魏东平. 2021. 智利三联点相关的板块相对运动及其地球动力学意义[J]. 地球物理学报,64(10):3567–3575. doi: 10.6038/cjg2021O0470 Jia H R,Wei D P. 2021. Relativemotion of plates related to the Chile triple junction and geodynamic significance[J]. Chinese Journal of Geophysics,64(10):3567–3575 (in Chinese).
李忠海,石耀霖. 2016. 三维板块几何形态对大陆深俯冲动力学的制约[J]. 地球物理学报,59(8):2806–2817. doi: 10.6038/cjg20160808 Li Z H,Shi Y L. 2016. Constraints of 3-D plate geometry on the dynamics of continental deep subduction[J]. Chinese Journal of Geophysics,59(8):2806–2817 (in Chinese).
刘梦雪,魏东平,史亚男. 2019. 俯冲初始时板块分界面形状对俯冲过程的影响[J]. 地球物理学报,62(1):78–87. doi: 10.6038/cjg2019L0717 Liu M X,Wei D P,Shi Y N. 2019. Effect of plate interface geometry on the evolution of subduction[J]. Chinese Journal of Geophysics,62(1):78–87 (in Chinese).
史亚男,魏东平,皇甫鹏鹏,李忠海,刘梦雪. 2019. 海洋板块俯冲作用下上覆大陆岩石层减薄机制的动力学模拟[J]. 地球物理学报,62(1):63–77. doi: 10.6038/cjg2019L0785 Shi Y N,Wei D P,Huangfu P P,Li Z H,Liu M X. 2019. Dynamics of thinning of overriding continental lithosphere induced by oceanic plate subduction:Numerical modeling[J]. Chinese Journal of Geophysics,62(1):63–77 (in Chinese).
沈晓明,张海祥,马林. 2010. 洋脊俯冲及其在新疆阿尔泰地区存在的可能证据[J]. 大地构造与成矿学,34(2):181–195. doi: 10.3969/j.issn.1001-1552.2010.02.004 Shen X M,Zhang H X,Ma L. 2010. Ridge subduction and the possible evidences in Chinese Altay,Xinjiang[J]. Geotectonica et Metallogenia,34(2):181–195 (in Chinese).
王振山,魏东平. 2018. 全球板块运动三联点形成与演化规律的研究进展[J]. 地球物理学进展,33(5):1834–1843. doi: 10.6038/pg2018BB0286 Wang Z S,Wei D P. 2018. Research progress on the formation and evolution of triple junctions of global plate motions[J]. Progress in Geophysics,33(5):1834–1843 (in Chinese).
徐佳静,王振山,王少坡,魏东平. 2019. 智利三联点南部扩张洋脊俯冲区域岩石层热结构的数值模拟[J]. 地球物理学报,62(12):4729–4737. doi: 10.6038/cjg2019M0592 Xu J J,Wang Z S,Wang S P,Wei D P. 2019. Numerical simulation of the lithospheric thermal structure in the subduction zone of the South Chile triple junction[J]. Chinese Journal of Geophysics,62(12):4729–4737 (in Chinese).
张克亮,魏东平. 2011. 双地震带的影响因素探讨[J]. 地球物理学报,54(11):2838–2850. doi: 10.3969/j.issn.0001-5733.2011.11.014 Zhang K L,Wei D P. 2011. On the influence factors of double seismic zones[J]. Chinese Journal of Geophysics,54(11):2838–2850 (in Chinese).
Agurto-Detzel H,Andreas R,Klaus B,Miller M,Iwamori H,Priestley K. 2014. Seismicity distribution in the vicinity of the Chile Triple Junction,Aysén Region,southern Chile[J]. J South Am Earth Sci,51:1–11. doi: 10.1016/j.jsames.2013.12.011
Assumpção M,Mei F,Andrés T,Julià J. 2013. Models of crustal thickness for South America from seismic refraction,receiver functions and surface wave tomography[J]. Tectonophysics,609:82–96. doi: 10.1016/j.tecto.2012.11.014
Bagherbandi M,Bai Y,Sjöberg L E,Tenzer R,Abrehdary M,Miranda S,Alcacer Sanchez J M. 2017. Effect of the lithospheric thermal state on the Moho interface:A case study in South America[J]. J South Am Earth Sci,76:198–207. doi: 10.1016/j.jsames.2017.02.010
Bangerth W, Dannberg J, Gassmoeller R, Heister T. 2020. ASPECT: Advanced solver for problems in Earth’s convection, user manual[BE/OL]. [2021-10-21]. https://www.math.clemson.edu/~heister/manual.pdf.
Bohm M,Lüth S,Echtler H,Bataille K,Group I W. 2002. The Southern Andes between 36° and 40° S latitude:Seismicity and average seismic velocities[J]. Tectonophysics,356(4):275–289. doi: 10.1016/S0040-1951(02)00399-2
Bondár I,Storchak D A. 2011. Improved location procedures at the International Seismological Centre[J]. Geophys J Int,186:1220–1244. doi: 10.1111/j.1365-246X.2011.05107.x
Bourgois J,Michaud F. 2002. Comparison between the Chile and Mexico triple junction areas substantiates slab window development beneath northwestern Mexico during the past 12−10 Myr[J]. Earth Planet Sci Lett,201:35–44. doi: 10.1016/S0012-821X(02)00653-2
Breitsprecher K,Thorkelson D J. 2009. Neogene kinematic history of Nazca-Antarctic Phoenix slab windows beneath Patagonia and the Antarctic Peninsula[J]. Tectonophysics,464(1/2/3/4):10–20. doi: 10.1016/j.tecto.2008.02.013
Contreras-Reyes E,Flueh E R,Grevemeyer I. 2010. Tectonic control on sediment accretion and subduction off south central Chile:Implications for coseismic rupture processes of the 1960 and 2010 megathrust earthquakes[J]. Tectonics,29:1–27.
Eakin C M,Obrebski M,Allen R M,Boyarko D C,Brudzinski M R,Porritt R. 2010. Seismic anisotropy beneath Cascadia and the Mendocino triple junction:Interaction of the subducting slab with mantle flow[J]. Earth Planet Sci Lett,297:627–632. doi: 10.1016/j.jpgl.2010.07.015
Fraters M,Thieulot C,van den Berg A,Spakman W. 2019. The geodynamic world builder:A solution for complex initial conditions in numerical modeling[J]. Solid Earth,(10):1785–1807.
Gleason G C,Tullis J. 1995. A flow law for dislocation creep of quartz aggregates determined with the molten salt cell[J]. Tectonophysics,247(1/2/3/4):1–23. doi: 10.1016/0040-1951(95)00011-B
Goddard A,Fosdick J C. 2019. Multichronometer thermochronologic modeling of migrating spreading ridge subduction in southern patagonia[J]. Geology,47(6):555–558. doi: 10.1130/G46091.1
Gresho P M,Lee R L,Sani R L,Maslanik M K,Eaton B E. 1987. The consistent Galerkin FEM for computing derived boundary quantities in thermal and/or fluids problems[J]. Int J Numer Meth Fl,7(4):371–394.
Guo C,Sun P,Wei D. 2021. Numerical simulation of the effects of wedge subduction on the lithospheric thermal structure and the seismogenic zone south of Chile Triple Junction[J]. Front Earth Sci,9:782458. doi: 10.3389/feart.2021.782458
Hamza V M,Dias F,Gomes A,Terceros Z. 2005. Numerical and functional representations of regional heat flow in South America[J]. Phys Earth Planet Inter,152:223–56. doi: 10.1016/j.pepi.2005.04.009
Heister T,Dannberg J,Gassmöller R,Bangerth W. 2017. High accuracy mantle convection simulation through modern numerical methods,II:Realistic models and problems[J]. Geophys J Int,210(2):833–851. doi: 10.1093/gji/ggx195
Hirth G, Kohlstedt D. 2004. Rheology of the upper mantle and the mantle wedge: A view from the experimentalists[C]// Geophysical Monograph Series. Washington D. C.: American Geophysical Union: 83–105.
Kirby S, Engdahl E R, Denlinger R. 1996. Intermediate-depth intraslab earthquakes and arc volcanism as physical expressions of crustal and uppermost mantle metamorphism in subducting slabs[C]// Geophysical Monograph Series. Washington D C: American Geophysical Union: 195–214.
Klotz J, Abolghasem A, Khazaradze G, Heinze B, Vietor T, Hackney R, Bataille K, Maturana R, Viramonte J, Perdomo R. 2006. Long-Term Signals in the Present-Day Deformation Field of the Central and Southern Andes and Constraints on the Viscosity of the Earth’s Upper Mantle[M]. Berlin: Springer: 65–89.
Kronbichler M,Heister T,Bangerth W. 2012. High Accuracy mantle convection simulation through modern numerical methods[J]. Geophys J Int,191(1):12–29. doi: 10.1111/j.1365-246X.2012.05609.x
Lagabrielle Y,Christèle G,René C M,Bourgois J,Martin H. 2000. Magmatic–tectonic effects of high thermal regime at the site of active ridge subduction:The Chile Triple Junction model[J]. Tectonophysics,326:255–268. doi: 10.1016/S0040-1951(00)00124-4
Lange D,Rietbrock A,Haberland C,Bataille K,Dahm T,Tilmann F,Flüh E R. 2007. Seismicity and geometry of the south Chilean subduction zone (41.5°S—43.5°S):Implications for controlling parameters[J]. Geophys Res Lett,34:L06311.
Lucazeau F. 2019. Analysis and mapping of an updated terrestrial heat flow data set[J]. Geochem Geophys Geosyst,20(8):4001–4024. doi: 10.1029/2019GC008389
Maksymowicz A,Eduardo C,Ingo G,Flueh E. 2012. Structure and geodynamics of the post-collision zone between the Nazca–Antarctic spreading center and South America[J]. Earth Planet Sci Lett,345-348:27–37.
Murdie R E,Prioe D J,Styles P,Flint S S,Agar S M. 1993. Seismic response to ridge-transform subduction:Chile triple junction[J]. Geology,21:1095–1098.
Naliboff J,Buiter S J H. 2015. Rift reactivation and migration during multiphase extension[J]. Earth Planet Sci Lett,421:58–67. doi: 10.1016/j.jpgl.2015.03.050
Oleskevich D A,Hyndman R D,Wang K. 1999. The updip and downdip limits to great subduction earthquakes:Thermal and structural models of Cascadia,south Alaska,SW Japan,and Chile[J]. J Geophys Res:Solid Earth,104:14965–14991. doi: 10.1029/1999JB900060
Richards F D,Hoggard M J,Cowton L R,White N J. 2018. Reassessing the thermal structure of oceanic lithosphere with revised global inventories of basement depths and heat flow measurements[J]. J Geophys Res:Solid Earth,123:9136–9161. doi: 10.1029/2018JB015998
Rose I,Buffett B,Heister T. 2017. Stability and accuracy of free surface time integration in viscous flows[J]. Phys Earth Planet Inter,262:90–100. doi: 10.1016/j.pepi.2016.11.007
Rybacki E. 2006. Influence of water fugacity and activation volume on the flow properties of fine-grained anorthite aggregates[J]. J Geophys Res:Solid Earth,200-201:1–9.
Scherwath M,Flueh E,Grevemeyer I,Tillman F,Contreras-Reyes E,Weinrebe W. 2006. Investigating subduction zone processes in Chile[J]. Eos Trans Am Geophys Un,87(27):265–272.
Scherwath M,Contreras-Reyes E,Flueh E R,Grevemeyer I,Krabbenhoeft A,Papenberg C,Petersen C J,Weinrebe R W. 2009. Deep lithospheric structures along the southern central Chile margin from wide-angle P-wave modelling[J]. Geophys J Int,179:579–600. doi: 10.1111/j.1365-246X.2009.04298.x
Shi Y,Wei D,Li Z,Liu M Q,Liu M. 2018. Subduction mode selection during slab and mantle transition zone interaction:Numerical modeling[J]. Pure Appl Geophys,175(2):529–548. doi: 10.1007/s00024-017-1762-0,175(2):529—548
Simmons N A,Forte A M,Boschi L,Grand S P. 2010. GyPSuM:A joint tomographic model of mantle density and seismic wave speeds[J]. J Geophys Res,115:B12310. doi: 10.1029/2010JB007631
Sisson V B,Pavlis T L,Roeske S M,Thorkelson D J. 2003. Introduction:An overview of ridge-trench interactions in modern and ancient settings[J]. Geol Soc Am Spec Papers,371:1–18.
Stein C A,Stein S. 1992. A model for the global variation in oceanic depth and heat flow with lithospheric age[J]. Nature,359(6391):123–129. doi: 10.1038/359123a0
Storchak D A,Harris J,Brown L,Lieser K,Shumba B,Verney R,Giacomo D D,Korger E I M. 2017. Rebuild of the bulletin of the International Seismological Centre (ISC),part 1:1964–1979[J]. Geosci Lett,4:32. doi: 10.1186/s40562-017-0098-z
Storchak D A,Harris J,Brown L,Lieser K,Shumba B,Di Giacomo D. 2020. Rebuild of the bulletin of the International Seismological Centre (ISC),part 2:1980–2010[J]. Geosci Lett,7:18. doi: 10.1186/s40562-020-00164-6
Tebbens S F,Cande S C,Kovacs L,Parra,J C,LaBrecque J L,Vergara H. 1997. The Chile ridge:A tectonic framework[J]. J Geophys Res:Solid Earth,102:12035–12059. doi: 10.1029/96JB02581
Tichelaar B W,Ruff L J. 1991. Seismic coupling along the Chilean subduction zone[J]. J Geophys Res,96(B7):11997. doi: 10.1029/91JB00200
Tetreault J L,Buiter S J H. 2012. Geodynamic models of terrane accretion:Testing the fate of island arcs,oceanic plateaus,and continental fragments in subduction zones[J]. J Geophys Res:Solid Earth,117(B8):B08403.
van der Hilst R,Hoop M. 2005. Banana-doughnut kernels and mantle tomography[J]. Geophys J Int,163:956–961. doi: 10.1111/j.1365-246X.2005.02817.x
Völker D,Ingo G,Michael S,Wang K,He J. 2011. Thermal control of the seismogenic zone of southern central Chile[J]. J Geophys Res,116:1–20.
Wei D, Seno T, 1998. Determination of the Amurian plate motion[C]// Mantle Dynamics and Plate Interactions in East Asia: Geodynamics Series. San Francisco: AGU: 337–346.
Wilks K R,Carter N L. 1990. Rheology of some continental lower crustal rocks[J]. Tectonophysics,182(1/2):57–77. doi: 10.1016/0040-1951(90)90342-6
Zhang K,Wei D. 2012. Correlation between plate age and layer separation of double seismic zones[J]. Earthquake Science,25:95–101. doi: 10.1007/s11589-012-0835-5
计量
- 文章访问数: 682
- HTML全文浏览量: 265
- PDF下载量: 172
